Seminar Archive
2021-2022 * 2019-2020+ * 2018-2019 * 2017-2018 * 2016-2017 * 2015-2016 *
2014-2015 * 2013-2014 * 2012-2013 * 2011-2012 * 2010-2011
2009-2010 * 2008-2009 * 2007-2008 * 2005-2006 * [Current]
2021-2022
*WEDNESDAY May 11, 12:30p.m. – 1:30 p.m. in TSC 122
Selfish Self-driving Cars - An Adventure in Algorithmic Game Theory
Dr. Philip Brown
Assistant Professor of Computer Science at UCCS
Rating: PG (Some undergraduate mathematics or computer science knowledge assumed)
🍕Pizza will be served outside Tutt Science at Noon.
This talk brought to you by the Society for Industrial and Applied Mathematics (SiAM)
Friday April 29, 12:30p.m. – 1:30 p.m. in TSC 122
You Can Be An Actuary!
Joanna Coclough '13
CC graduate and actuary at Anthem
Rating: G (No mathematics or computer science knowledge assumed)
🍕Pizza will be served outside Tutt Science at Noon.
Note for Majors: This talk is eligible for write-up!

Math and CS Capstone presentations will be held on three afternoons in Block 7.
2:30 - 4:30 pm in Tutt Science Lecture Hall
(with refreshments prior, at 2pm outside Tutt Science)
Note: These presentations are not eligible for major write-ups
 .
. 
Friday April 8, 12:30p.m. – 1:30 p.m. in TSC 122
Machine Learning Without Structure
Dr. Cory Scott
Rating: PG-13 (Considerable undergraduate mathematics or computer science assumed)
🍕Pizza will be served outside Tutt Science at Noon. 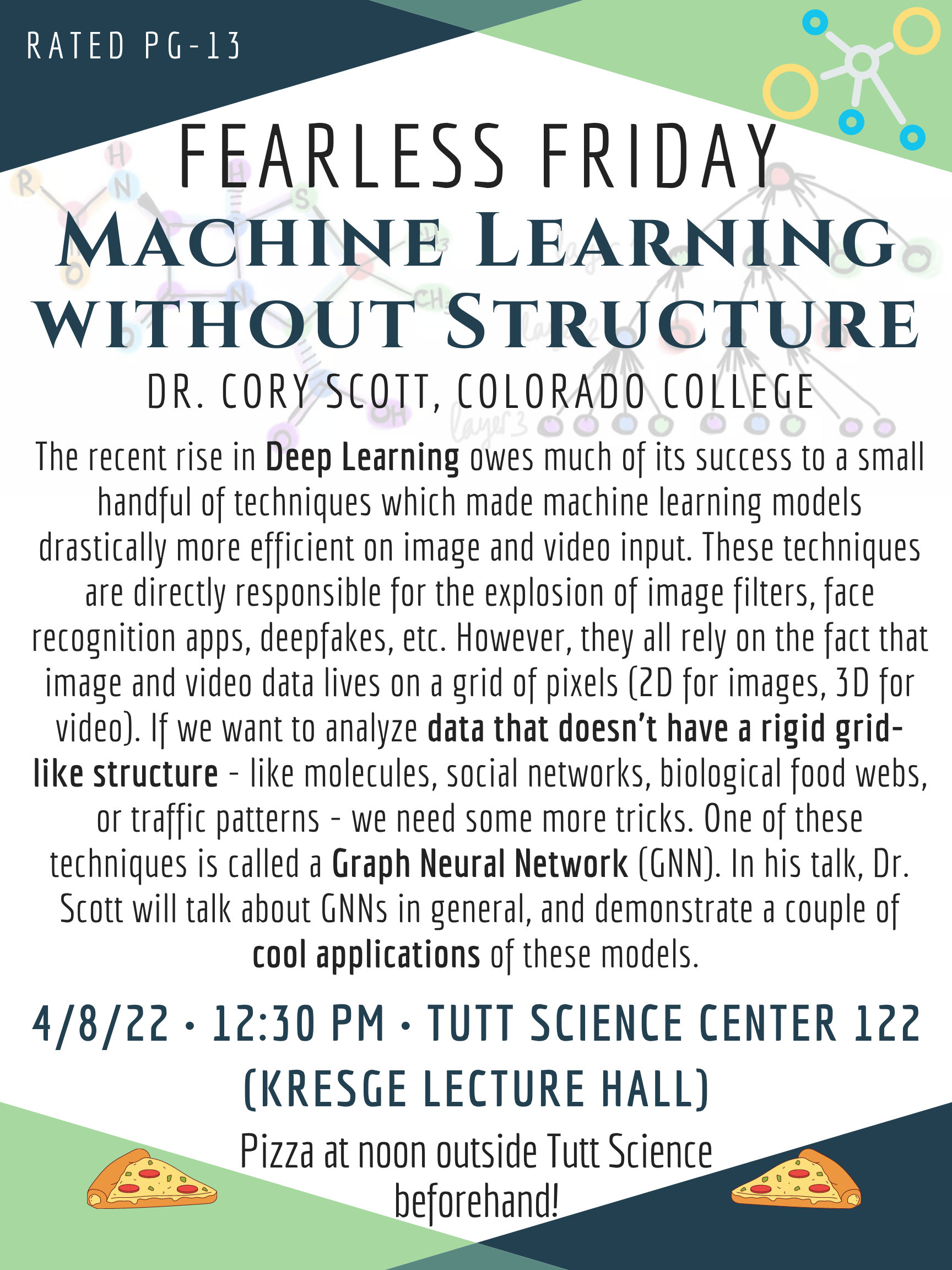
*Thursday* March 31, 12:30p.m. – 1:30 p.m. in TSC 223
Artistic Mathematics:Truth and Beauty
Dr. Henry Segerman
Rating: G (No mathematics or computer science background required)
LIVE on ZOOM from Oklahoma State University
🍕Pizza will be served outside Tutt Science at Noon. 
Friday Feb 25th, 12:30p.m. – 1:30 p.m. in TSC 229
Jane McDougall
🍕Pizza will be served outside Tutt Science at Noon.
Rating: PG13 (Considerable undergraduate mathematics or computer science assumed)

Friday October 29th, 12:30p.m. – 1:30 p.m. in TSC 229
Michael Zowada, UCCS 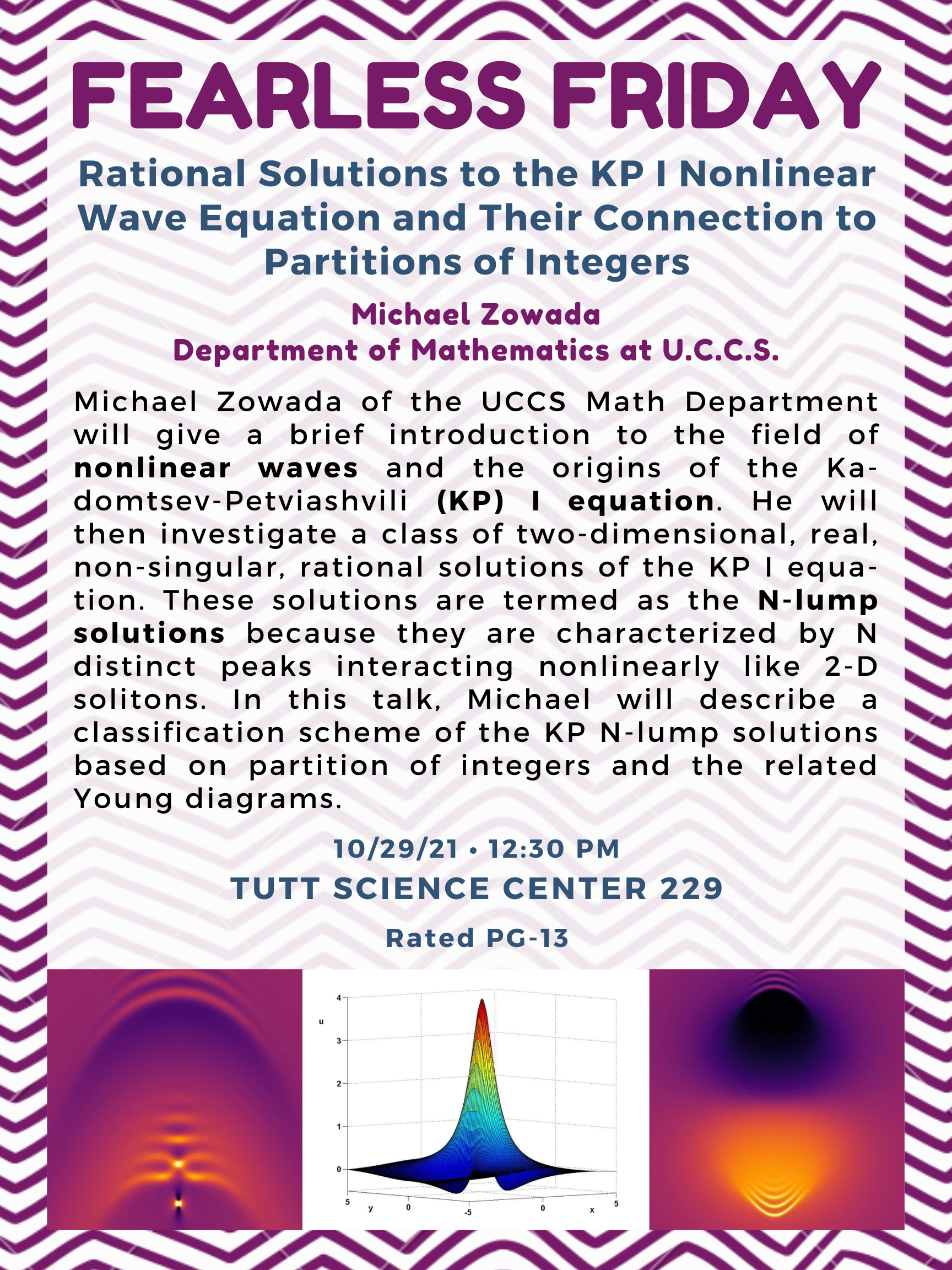
Friday November 5th, 12:30p.m. – 1:30 p.m. in TSC 122
Dr. Minho Kim, Colorado College
Friday November 12th, 12:30p.m. – 1:30 p.m. in TSC 229
Dr. Lauren Nelsen, Colorado College
Friday October 1st, 3p.m. – 4:15 p.m. in Tutt Science Tent
Student Poster Session
Friday October 8th at 12pm, Kresge Lecture Hall - Homecoming event
Alan Yeung - Class of 2016
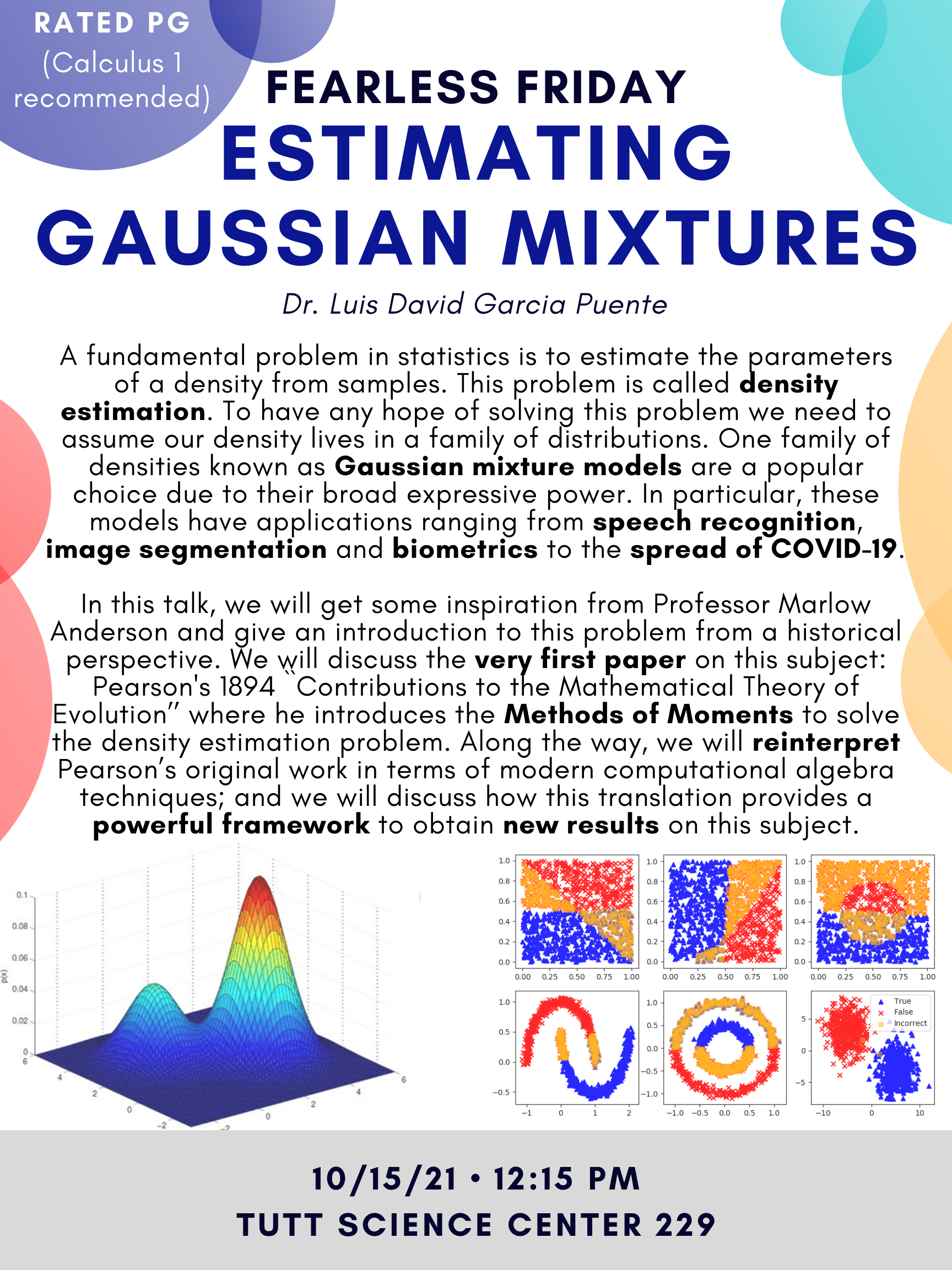
Friday October 15th at 230pm in TSC229
Title: Estimating Gaussian Mixtures
Speaker: Professor Luis Garcia Puente, Colorado College
2:30pm in Tutt Science 229
Jane McDougall - Rosette Minimal Surfaces
Followed by Ice Cream Social outside in the Tutt West Lawn at 3:30pm
12:30pm in SOUTH HALL COMMONS
Light refreshments afterward
David Brown - An Introduction to Infectious Disease Modeling
Rating PG. The main points will be accessible to anyone with a Calculus 1 background
2:30pm in TSC 229
Barry S Fagin, US Air Force Academy - Idempotent Integers: The complete class of numbers N = pq that work correctly in RSA -- A Mathematical Curiosity
2019-2020+
BLOCK 5- January/February 2020
Friday, February 7th, at 2:30pm
TSC 229
Speaker: Professor Mike Siddoway
Title: "The Wind Erosion Equation"
Rated: PG
Abstract:

***
Friday, January 31st, at NOON
TSC 122 Lecture Hall
Pizza will be provided!

Speaker: Professor Renzo Cavalieri of CSU
Title: "Geometry and Combinatorics of Moduli Spaces of Rational Pointed Curves"
Rated: PG
Abstract: The idea of a moduli space, i.e. a space whose points parameterize interesting geometric objects, is central in modern mathematics. In this talk we will introduce the notion of moduli space, and specifically investigate spaces whose point corresponds to configuration of points on spheres. These spaces have a very rich geometry (they are smooth, projective varieties) and a very rich combinatorial structure (they have a boundary stratification indexed by stable dual graphs). Time permitting, we will present a recent result, joint with Maria Gillespie and Leonid Monin, that connects the degree of some projective embedding of these spaces to the combinatorics of parking functions.

****
BLOCK 4 - December 2019
Friday, December 6th, at NOON
TSC 122 Lecture Hall
Speaker: Professor Benjamin Ylvisaker
Title: TBA
Rated: TBA
****
Wednesday, December 11th, at 4pm
TSC 122 Lecture Hall
Speaker: Cynthia Chapple - FemSTEM Keynote Speaker

BLOCK 3 October/November 2019
Friday, November 1st, at NOON
TSC 122 Lecture Hall
Pizza will be provided.
![]()
Speaker: Professor Andrew Kelley
Title: "A Proof of Stirling's Formula"
Rated: PG
Abstract:

***
Friday, October 25th, at 2:30pm
TSC 229
Speaker: Professor Kirsten Hogenson
Title: "Finding Long Cycles in Graphs"
Rated: PG-13
Abstract:

BLOCK 2 September/October 2019
Friday, September 27th, at 2:30pm
TSC 229
Speaker: Professor Matthew Whitehead
Title: "How to Generate Fake News"
Rated: PG (Some undergraduate computer science knowledge helpful)
Abstract:

BLOCK 1 August/September 2019
Friday, September 13th, at 2:30pm
TSC 229
Speaker: Jake Pichelmeyer of Kansas State
Title: "The Minimal Genus Problem"
Rated: G (No math knowledge required)
Abstract:

***
Friday, September 6th, at NOON
TSC 122 Lecture Hall
(Ice Cream Social to follow in the Math/CS lounge on the 2nd floor!)
Speaker: Professor Mike Siddoway
Title: "The Call of the Lune"
Rated: PG-13
Abstract:
***
Friday, August 30th, at 2:30pm
TSC 229
Speaker: Professor Marlow Anderson
Title: "Yet Another Solution of the Cubic Equation"
Rated: PG
Abstract:

***
2018-2019
BLOCK 8 -April/May 2019
Friday, May 10th, at 2:30pm
TSC 229
Speaker: Dr. Bill Eberle of Tennessee Tech
Title: "Graph-Based Anomaly Detection"
Rated: PG
Abstract:
***
Friday, May 3rd, at Noon
TSC 122 Kresge Lecture Hall
Speaker: Dr. Jenny Beineke
Title: "Splendor in the Graphs"
Rated: PG

Pizza Provided
Abstract:
***
Friday, April 26th, at 2:30pm
TSC 229
Speaker: Dr. Tom Williams, Colorado School of Mines
Title: "COME TO THIS TALK, HUMAN!"
Rated: PG
Abstract:

BLOCK 7 - March/April 2019
Thursday, April 4th, atNoon
TSC 122 Lecture Hall
Speaker: Dr. Amelia Taylor, Data Scientist, Zymergen Inc,
Title: "Data Did That!"
Rated:
Abstract:

BLOCK 6 - February/March 2019
Monday, February 25th, at 2:15pm
TSC 229
Speaker: Dr. Kaitlin Hill
Title: "Dynamical systems for a changing world: from Arctic tipping points to the leaky pipeline effect"
Rated: PG-13 (A lot of undergraduate mathematics knowledge assumed.)
Abstract:

*
Tuesday, February 26th, at Noon
TSC 122, Kresge Lecture Hall
Pizza will be provided!

Speaker: Dr. Kaitlin Hill
Title: "Dynamical systems, chaos, and the weather; or, Loki should have been a math major"
Rated: PG (some undergraduate mathematics knowledge assumed.)
Abstract:

*****
Thursday, February 21st, at 3pm
TSC 229
Speaker: Dr. Ike Agbanusi
Title: "Large Coupling Problems"
Rated: PG-13 (A lot of undergraduate mathematics knowledge assumed.)
Abstract:

Thursday, February 22nd, at Noon
TSC 122, Kresge Lecture Hall
Pizza will be served!

Speaker: Dr. Ike Agbanusi
Title: "How to Date and Other Stories"
Rated: PG (some undergraduate mathematics knowledge assumed.)
Abstract:

*****
Monday, February 18th, at 3pm
TSC 229
Speaker: Dr. Yi Jiang
Title: "Invariant-region-preserving High Order Discontinuous Galerkin Schemes For Hyperbolic Conservation Law Systems"
Rated: R (A lot of graduate mathematics knowledge assumed.)
Abstract:
Tuesday, February 19th, at NOON
TSC 122, Kresge Lecture Hall
Pizza will be served!

Speaker: Dr. Yi Jiang
Title: "Solving Equations in Ancient China"
Rated: PG (Some undergraduate mathematics knowledge assumed.)
Abstract:

BLOCK 5 - January/February 2019
Monday, February 11th, at NOON
TSC 122, Kresge Lecture Hall
Speaker: Dr. Wan Bae
Title: "Voronoi Diagrams - How Voronoi Diagrams Help Computer Scientists Solve Real-world Problems"
Rated: PG (Some undergraduate computer science knowledge assumed.)
Abstract:

*
Monday, February 12th, at 3:30pm
TSC 229
Speaker: Dr. Wan Bae
Title: "Predictive and Exposome Analytics for Individual-level Health Risk Management"
Rated: PG (Some undergraduate computer science knowledge assumed.)
Abstract:

*****
| Monday, STUDENT TALK February 4th, at NOON | Monday, RESEARCH TALK February 4th, at 3pm |
| TSC 122, Kresge Lecture Hall | TSC 229 |
| Speaker: Dr. Aaron Bauer | Speaker: Dr. Aaron Bauer |
| Title: tba | Title: tba |
| Rated: tba | Rated: tba |
| Abstract: | Abstract: |
Thursday, January 31st, at 3pm
TSC 229
Speaker: Dr. Kelly Shaw
Title: "Moving Away From Unchecked Power"
Rated: PG-13 (Some graduate computer science knowledge assumed.)
Abstract:
![Jan 31 - Helping Software Use Parallel Systems[1]](../images/FF/Jan 31 - Helping Software Use Parallel Systems1.jpg)
*
FEARLESS FRIDAY Talk, February 1st, at NOON
TSC 122, Kresge Lecture Hall
Speaker: Dr. Kelly Shaw
Title: "Programming Modern Computer Systems"
Rated: PG (Some undergraduate computer science knowledge assumed.)
Abstract:
![Feb 1 - Programming Modern Computer Systems[1]](../images/FF/Feb 1 - Programming Modern Computer Systems1.jpg)
***
Tuesday, January 29th, at NOON
TSC 122 Kresge Lecture Hall
PIzza will be provided!

Speaker: Dr Dan Ellsworth
Title: "Writing for a Non Human Audience"
Rated: G (No math or cs knowledge assumed.)
Abstract:

***
Monday, January 28th, at 3pm
TSC 229
Speaker: Dr Dan Ellsworth
Title: "Moving Away From Unchecked Power"
Rated: PG (Some undergraduate computer science knowledge assumed.)
Abstract:
![Jan 28 - Moving away from unckecked power[2]](../images/FF/Jan 28 - Moving away from unckecked power2.jpg)
***
| Monday, January 28th, at 3pm | Tuesday, January 29th, at NOON |
| TSC 229 | TSC 122, Lecture Hall |
| Speaker: Dr. Dan Ellsworth | Speaker: Dr. Dan Ellsworth |
| Title: "Moving Away from Unchecked Power" | Title: |
| Rated: PG | Rated: tba |
Abstract:![Jan 28 - Moving away from unckecked power[2]](../images/FF/Jan 28 - Moving away from unckecked power2.jpg) |
Abstract: |
Wednesday, January 23rd, at NOON
TSC 122 Kresge Lecture Hall
PIzza will be provided!

Speaker: Dr Bjorn Poonen from MIT
Title: "Undecidability in Number Theory"
Rated: PG-13 (Some graduate mathematics knowledge assumed.)
Abstract:

***
BLOCK 4 - November/December 2018
Friday December 14th, at 2:30pm
TSC 229
Speaker: Dr. Fred Tinsley
Title: "Sometimes Extreme Measures are Required"
Rated: PG-13 (Some graduate mathematics knowledge assumed.)
Available to be written up for the computer science and math seminar talk write up requirement. Do your writeups!!
Abstract:

***
Friday November 30th, at 2:30pm
TSC 229
Speaker: Professor Lance Littlejohn from the Department of Mathematics at Baylor University
Title: "Two Applications of Green's Functions"
Rated: PG-13 (Some graduate mathematics knowledge assumed.)
Abstract:

Friday, December 7th, noon (TSC122)A Declaration of Dependence - Modeling Dependent Random Variables and Some Earth-shattering Implications of Doing it Wrong, by Dr. David Brown, Colorado College (Rated PG)
The concepts of independence and dependence are fundamental in probability and statistics. In this talk, Dr. Brown will review the basic idea of independence and why it is so pervasive. He will discuss the uses and misuses of correlation to measure the dependence between two or more variables, and introduce a tool called a "copula" for studying dependence structures. When mathematical models of complicated phenomena are based on erroneous assumptions about dependence, things can go very wrong. Dr. Brown will illustrate this fact with examples from the 2016 presidential election forecasts and the calculation of risk for complex financial instruments that contributed to the 2008 financial crisis.
Friday, December 14th, 2:30 (TSC229), Sometimes Extreme Measures Are Required, by Dr. Fred Tinsley, Colorado College (Rated PG13)
Mathematics initially attracts many enthusiasts with its unending varieties of fascinating problems and puzzles whose solutions require good intuition and ability but very few mathematical tools. However, further exploration can lead very quickly to simply stated problems for which no amount of cleverness can provide a succinct solution. A resolution may result from decades of the concerted efforts by the best mathematicians in the world. Fermat's Last Theorem is the most famous (and perhaps most extreme) such example. Dr. Tinsley will investigate a bit more modest illustration involving the interplay of geometry and algebra. Having had courses in abstract algebra, geometry or analysis are not specifically assumed, but should enhance your experience. Moreover, this fact is very much in the spirit of the talk.
BLOCK 3 - October/November 2018
Friday November 9th, at 2:30pm
TSC 229
Speaker: Dr. Marlow Anderson
Title: "Ramanujan Simple Cubics"
Rated: PG-13 (Some graduate mathematics knowledge assumed.)
Abstract:

***
Friday November 2nd, at Noon
TSC 122 Lecture Hall
Pizza will be provided
![]()
Speaker: Joe Howard, a CC alumnus (class of 2015), Peak to Peak Charter School
Title: "Tackling Derivatives for the First Time: Mastering the Basics of Differential Calculus"
Rated: PG (Some undergraduate mathematics knowledge assumed.)
Abstract:

***
Friday, October 26th, at 2:30pm
TSC 229
Speaker: Dr. Jacob Rezac from CU Boulder and the National Institute of Standards and Technology
Title: "Moving beyond F=ma: Inverse Problems in Dynamic Force Measurements"
Rated: PG-13 (Some graduate mathematics knowledge assumed.)
Abstract:

BLOCK 2 - October 2018
Friday October 12th, at Noon
TSC 122 Lecture Hall
Pizza will be provided
![]()
Speaker: Dr. Rebecca Mitchell, '12
Title: "Designing a finite-time mixer of viscous fluids: optimizing stirring for Two-dimensional maps"
Rated: PG (Some mathematics knowledge assumed.)
Abstract:

*
Friday October 5th, at Noon
TSC 122 Lecture Hall
Pizza will be provided
![]()
Speaker: Professor Richard Wellman, Colorado College
Title: "Weaving Looms, Quantum Dots, and Self-Aware Robots: what is a computer and what does it do?"
Rated: PG (Some computer science knowledge assumed.)
Abstract:
BLOCK 1 - August/September 2018
Friday September 7th, at Noon
TSC 122 Lecture Hall
Speaker: Professor Ben Ylvisaker, Colorado College
Title: "You Can't Roll Back Guided Missiles"
Rated: PG (Some computer science knowledge assumed.)
Abstract:

*
Friday August 31st, at 2:30pm
TSC 229
Speaker: Professor Marlow Anderson, Colorado College
Title: "Italian Integration"
Rated: PG (Some mathematics knowledge assumed.)
Abstract:

*
2017-2018
BLOCK 8 - April/May 2018
Friday, May 11th, 2:30PM
TSC 229
Speaker: Stefan Erickson
Title: The Fantastic P-Adics
Rated: PG

**********
Friday, May 4th, Noon
TSC 122
Pizza will be served!
Speaker: Dr. Amelia Taylor, Data Scientist at Zymergen Inc
Title: Robots, Biology, and Data Science
Rated: G/PG

**********
Friday, April 27th, 2:30 pm
TSC 229
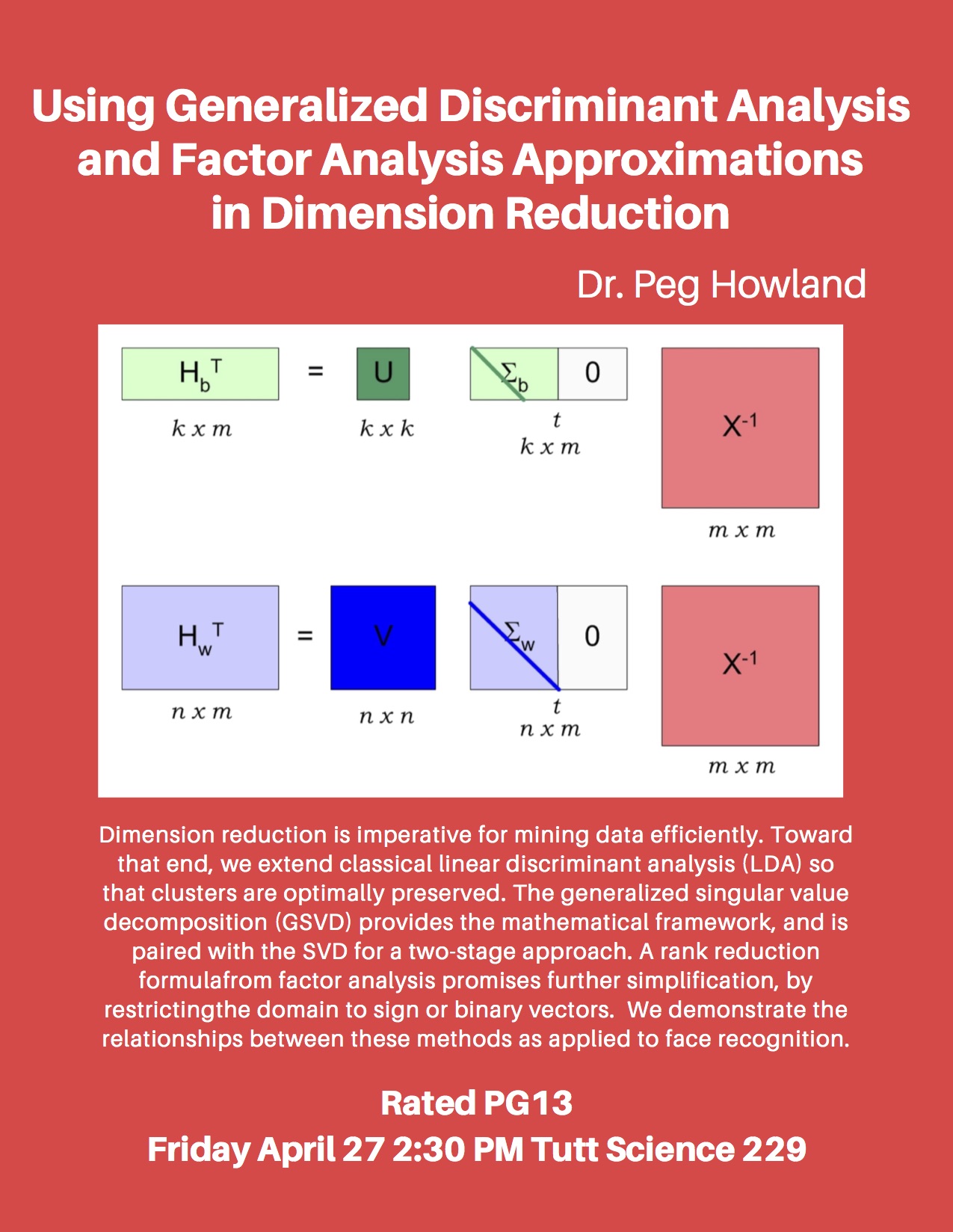
Speaker: Peg Howland, Visiting Professor
Title: Using Generalized Discriminant Analysis and Factor Analysis Approximations in Dimension Reduction
Rated: PG-13
BLOCK 5 - January/February 2018
Friday, February 9th, Noon
TSC 122 Lecture Hall
Title: Getting into the fold: connections between mathematics and origami
Rated:PG-13
PIZZA WILL BE PROVIDED!

____
Friday, February 2nd, Noon
TSC 122 Lecture Hall
Title: A Smorgasbord of Arithmetic Geometry
Rated:G
PIZZA WILL BE PROVIDED!

Friday, February 2nd, 3pm
TSC 229
Title: Rigidity: Local Systems and Beyond
Rated: R

____
Friday, January 26th, 2:30pm
TSC 229
Speaker: Dan Ellsworth, visiting professor, Colorado College
Title: Maintenance of Hard Power Caps and Job Throughput Performance in HPC Systems: Better to go slow now or faster later
Rated:PG/PG-13

BLOCK 4 - November/December 2017
**Note: This block will feature talks predominately by candidates for Statistics Tenure Track position.**
Thursday, December 14th, 3pm
TSC 229
Title: An Option Pricing Model With Memory
Rated: PG-13
and
Friday, December 15th, 12pm
TSC 122 Lecture Hall
(Pizza will be served!)

Title: A Random Walk Through Probability and Statistics
Rated: PG

____
Thursday, November 30th, 3pm
TSC 229
Title: Computational Probability: Lifetime(s) of Research
Rated: PG-13 (with some R scenes)

and
Friday, December 1st, 12pm
TSC 122 Lecture hall
Title: A First Year Experience: Real World Solutions with Simulation
Rated: G

BLOCK 3 -October/November 2017
Friday November 10th, 2:30pm
TSC 229
Speaker: Kathy Merrill, Professor Emerita
Title: Wavelets for Crystallographic Groups
Rated: R

Friday November 3rd, Noon 12pm
TSC 122 Lecture Hall
with Pizza!

Speaker: Robert Gordon
Title: A sampling of Statistics at Intel Corporation
Rated: PG

Week 1: Kirsten Hogenson
BLOCK 2 - September/October 2017
Friday October 6th, Noon - 1pm
TSC 122 Lecture Hall
Pizza will be provided!

Speaker: Steven Janke, Professor Emeritus
Title: "Celtic Knots: Drawing and Analysis"
Rated: PG (Some undergraduate mathematics or computer science knowledge assumed.)

*
Friday September 29, 2:30pm
TSC 122 Lecture Hall
Speaker: Richard Koo, Visiting Assistant Professor
BLOCK 1 - September 2017
Friday September 15th,
Skilling Commons (TSC 2nd Floor)
Student Poster Session
*
Friday September 8th, Noon - 1pm
TSC 122 Lecture Hall
Department Research Overview, followed by an ICE CREAM SOCIAL in the Math/CS Lounge.
Thinking about writing a senior thesis? Or a summer research project? Come to the Fearless Friday this week to get an idea of what your professors are working on. Each department member will give a brief overview of their research and projects that students might get involved in. Followed by an ice cream social in the Math&CS Lounge.

*
Friday, September 1st at 2:30pm
TSC 122 Lecture Hall
Speaker: Assistant Professor Matthew Whitehead, Colorado College
Title: "Visualizing Artificial Neural Network Language Learning"
Rated: PG/PG13 (A lot of computer science knowledge assumed.)
Abstract: When working with artificial neural networks that have complex structure, perhaps consisting of millions of parameters, it can be extremely difficult for humans to understand how the system is making decisions. For example, software emulating a pathologist may process visual biopsy data and classify a tumor as malignant, but it would have no ability to relay how and why that classification was made. In this talk, we will discuss a technique for visualizing what language processing deep artificial neural networks have learned and how they have learned it.

*
2016-2017
BLOCK 8 - April/May 2017
Friday, May 12th at 3pm
TSC 229
Speaker: Visiting Math Candidate
Title: "Counting Maximal Subthings"
Rated: PG-13 (A lot of undergraduate mathematics or computer science knowledge assumed.)
Abstract: Suppose we have an infinite group. How can we count its maximal subgroups? Assuming there are infinitely many, what then? Let's arrange them by their size - i.e., their index, and count only those of a given index. Then send the index to infinity. In this talk, we will count maximal subgroups of certain groups--- arranged by their index. We will also count maximal ideals in a couple of rings and maybe even count other maximal subthings in other mathematical objects.
*
Friday, May 5th at Noon
TSC 122 Lecture Hall
Speaker: Jane McDougall
Title: "Complex Mappings and Logarithmic Exercises"
Rated: PG (Some undergraduate mathematics or computer science knowledge assumed.)
Abstract: In working with complex variables, we have to shed our attachment to single picture graphs, given that we are working with a two dimensional domain and range. But there are still ways to visualize complex functions. In this talk I will discuss the benefits of viewing complex functions as mappings, especially those that are one to one. I will mention some recent student projects, and moreover involve you in some exercises involving the logarithm that will allow us to revisit ideas about continuity and connectedness.
*
Friday April 28th at 2:30pm
TSC 122 Kresge Lecture Hall.
Speaker: Craig Guilbault, University of Wisconsin-Milwaukee, will give a talk in honor of Fred Tinsley
Title: "Group theory, topology, and an attempt to capture infinity: Some mathematical adventures with Fred Tinsley".
Abstract: In this talk, I will describe my long-running collaboration with Fred Tinsley, which has aimed for a better understanding the topology of "non-compact manifolds". Think of these objects as possible shapes for an infinite universe. Our goal is to better understand those shapes by finding hidden structures on them. One of our primary tools is elementary group theory. By using lots of pictures and lots of examples (and avoiding technical details) , I will show how the algebraic tools of group theory play a natural and sometimes surprising role in the very geometric field of manifold topology.
Fred's retirement reception will follow at 4:00 in the Stewart House (1228 Wood Avenue)
*
**************************************************************************************
BLOCK 7 - March/April 2017
Friday, April 7th at 2:00pm
TSC 122 Lecture Hall
Speaker : Danny Krizanc Wesleyan University
Title: THE COW PATH PROBLEM & SOME OF ITS VARIATIONS
Rated: PG (Some undergraduate mathematics or computer science knowledge assumed.)
Abstract: A myopic cow stands up against a fence pining for the pasture on the other side. She knows there is a gate somewhere along the fence allowing entrance to this little piece of heaven but is not sure where it is. What strategy should she use to find it? This problem, variously called The Cow Path Problem or The Linear Search Problem, was introduced in the 1960s and has been the subject of a lot of research. In computer science, it is seen as one of the prototypical problems in the study of so-called competitive algorithms. In this talk, solutions to the original problem as well as a number of its variations (including the "faulty" cow version) will be surveyed.
*
Friday March 31st at 2:30 PM
TSC 229
Speaker:Cecilia Diniz Behn Colorado School of Mines
Title: A MAP-BASED APPROACH TO UNDERSTANDING CIRCADIAN MODULATION OF SLEEP-WAKE PATTERNS
Rating: PG-13 (A lot of undergraduate mathematics or computer science knowledge assumed.)
Abstract: The 24-h circadian pacemaker interacts with the homeostatic sleep drive to strongly modulate the timing of human sleep, and the interactions among these processes change across the lifespan. To investigate the modulation of sleep-wake cycle dynamics by the circadian rhythm, we computed a one-dimensional map for a high dimensional physiologically-based model of the sleep-wake neuronal network regulating human sleep. Analysis of this piecewise continuous map reveals changes in sleep patterning, including the occurrence of rapid eye movement sleep, for sleep occurring over different circadian phases. Using the map, we analyze model bifurcations as homeostatic sleep drive parameters are varied. This analysis has implications for understanding the dynamics of the transition from napping to non-napping behavior that occurs in early childhood.
*
**************************************************************************************
BLOCK 6 - March 2017
Friday, March 10 at 2:30 PM
TSC 229
Speaker: Oksana Bihun, University of Colorado, Colorado Springs
Title: Isospectral Matrices and Zeros of Orthogonal Polynomials
Rating: PG-13 (A lot of undergraduate mathematics or computer science knowledge assumed.)
Abstract: We will present a newly discovered matrix M, and several other new matrices, with the following properties. The matrix M is defined in terms of the zeros and the parameters of a N-th degree polynomial p_N. The polynomial p_N belongs to an orthogonal polynomial family from the top of the q-Askey scheme. The matrix M is isospectral, that is, its neat eigenvalues do not depend on the zeros of the polynomial p_N and depend only on some of its parameters. This remarkable matrix encodes multiple new algebraic relations satisfied by the zeros of the polynomial p_N. The matrix M is discovered via a linearization of the system of ODEs satisfied by the zeros of a certain polynomial, with time-dependent coefficients, which itself solves a certain Differential q-Difference Equation (DqDE). This is a joint work with Francesco Calogero.
*
Friday, March 3 at 12:00 PM
TSC 122 Kresge Lecture Hall
FREE PIZZA!
Speaker: Hanson Smith, CC alum '14, Math Graduate Student at CU Boulder
Title: A HISTORICAL MOTIVATION QUADRATIC RECIPROCITY
Rating: PG* (*a familiarity with modular arithmetic will be assumed)
Abstract: When I first saw quadratic reciprocity, I remember being quite confused as to what the theorem was actually saying and as to why I should care. It wasn't until much later that I began to appreciate how elegant and useful quadratic reciprocity actually is. The goal of this talk is to skip the confusion and motivate quadratic reciprocity historically. Working from Fermat forward, we will see the kind of questions that likely motivated quadratic reciprocity. In stating quadratic reciprocity, we will see how quickly and simply it can solve a class of Diophantine problems. Finally, we will give a proof of quadratic reciprocity using Gauss sums. Though this will require us to do some arithmetic over finite fields, intuition will be a priority
Followed by an Informal Grad School Info Session at 1:00 PM - TSC 122
**************************************************************************************
BLOCK 5 - Jan/February 2017
Math Professor Candidate Talks:
Thursday February 2nd, 3:00pm
TSC 229
(Research Talk) Title: Can you hear the shape of a drum?
Rating: PG-13 (A lot of undergraduate mathematics or computer science knowledge assumed.)
Abstract: I will discuss Mark Kac's famous question as to what geometric information is encoded in the Laplace spectrum of a manifold. The Laplacian is a generalized second derivative and we consider its eigenvalues and eigenfunctions. We'll see examples of spaces which have the same Laplace spectrum (isospectral) yet which are geometrically distinct (non-isometric). I'll present a pair of isospectral spaces that I've constructed together with Mary Sandoval in order to examine their inaudible geometric properties.
and
Friday February 3, Noon
TSC 122 Lecture Hall
(General Talk) Title: The classification of closed surfaces
Rating: G/PG
Abstract:I'll start by discussing the basic distinction between geometry and topology. The notion of topological equivalence will allow us to classify closed surfaces by their genus. I'll give a pictorial definition of Gaussian curvature and conclude by exploring a fundamental relationship between geometry and topology via the theorem of Gauss-Bonnet.
*
Math Professor Candidate Talks:
Monday January 30th, 3:00pm
TSC 229
(Research Talk) Title: VERTEX ALGEBRAS AND AUTOMORPHISMS
Rating: PG-13 (A lot of undergraduate mathematics or computer science knowledge assumed.)
Abstract: The notion of a vertex algebra was introduced in 1986 by Richard Borcherds and refined by Frenkel, Lepowsky, and Muerman in 1988. These objects have been fundamental in the years since in subjects as diverse as the theory of finite simple groups, modular functions, string theory, and the representation theory of infinite dimensional Lie algebras. In this talk, we explore the notion of a vertex algebra as the simultaneous generalization of a Lie algebra and a commutative ring. Further, there will be a discussion of the role of automorphisms of vertex algebras - both in terms of twisted modules and invariant subspaces. We finish with an in depth example, which is the product of a summer research project involving CC students Olivia Chandrasekhar and Hanbo Shao.
and
Tuesday January 31st, Noon
TSC 122 Lecture Hall
(General Talk) Title: THE BASICS OF INTEGER PARTITIONS
Rating: G/PG
Abstract:
A partition of n is any list of positive integers such that their sum is n. For example (3,2,1) is a partition of six because 6=3+2+1.
Notice that there are exactly four partitions of seven with odd parts: 7
5+1+1
3+1+1+1+1
1+1+1+1+1+1+1
There are also exactly four partitions of seven with distinct parts: 7
6+1
5+2
4+3
The fact that seven splits four ways under each of these conditions is not a coincidence! During this talk we will explore the theory of integer partitions and uncover and prove some surprising identities.
*
Math Professor Candidate Talks:
Thursday January 26th, 2:30pm
TSC 229
(Research Talk) Title: Periodic Paths on the Pentagon
Rating: PG (assumes Linear Algebra)
Abstract: Periodic billiard paths on regular polygons are nice to look at, and have beautiful mathematical structure related to continued fractions. I'll show you what happens on the familiar square, and then delve into my recent work on the regular pentagon.
and
Friday January 27th, Noon
TSC 122 Lecture Hall
(General Talk) Title: Three flavors of billiards
Rating: PG (assumes knowledge that there are a lot more irrationals than rationals)
Abstract: The mathematical study of billiards extends well beyond the popular table game. I'll tell you about three different types of billiards (inner billiards, outer billiards and tiling billiards), and I'll explain some of the research I've done in two of these areas.
*
**************************************************************************************
BLOCK 3
Friday, November 11th, 2:30 PM
TSC 229
Speaker: Robert Gordon
Title: An Application of Combinatorial Probability for Measuring Fairness of a Corporate Personnel Process
Rating: PG
Abstract: A class of probability models that may be utilized to evaluate fairness of a personnel process upon a subgroup of employees of an organization is presented. The data describing the outcome of the personnel process under evaluation is a 2 x k table where the column categories are ordered. The group of employees is partitioned into two subsets, the subset of employees against whom adverse impact of the employment process is being evaluated, called the protected class and the complementary subset. In this application, the row and column totals are fixed at the observed values. I compute an index of adverse impact on the protected class of employees. Two examples are presented. The first, evaluating potential employment discrimination, is an inference upon the initial job assignments of protected class members in the unskilled workforce of a large factory. The second, utilized to analyze tables from an employee job satisfaction survey in an engineering R&D organization, evaluates whether female employees as a group show lower job satisfaction than male employees.
**************************************************************************************
**************************************************************************************
2015-2016
BLOCK 8 - April/May 2016
Friday, April 29th at Noon
TSC 122 Lecture Hall
PIZZA PROVIDED
Speaker: Professor Emerita, Colorado College - Kathy Merrill
Title: "Taming Messy numbers"
Rated: G (General Audience - No mathematics or computer science knowledge assumed.)
Abstract: Mathematical applications regularly require that we make simple rational approximations to messy numbers. For example, building the scale used in western music requires reconciling fifths and octaves, and thus making a simple approximation to the log (base 2) of 3. Developing a calendar based on both lunar and solar cycles requires a simple approximation to (365.242199)/(29.350588). Continued fractions provide the best way of approximating irrationals and messy fractions with simple fractions. This talk will describe the continued fraction algorithm both numerically and geometrically, and discuss applications that make use of it, such as those mentioned above. We will also explore continued fraction approximations for old friends like e, pi, the square root of 2, and the golden mean.
*
**************************************************************************************
BLOCK 7 - March/April 2016
Friday, April 1st
TSC 122 Lecture Hall
Pizza Provided
SENIOR CAPSTONE PRESENTATIONS
Seniors present their capstone projects. Tentative Schedule of speakers:
12:00-12:20 - Yichun Shi - An Investigation of Bullying in Social Networks
12:25-12:45 - Venkatasai Ganesh Karapakula - Weird Variation in Bounded Stuff
12:50-1:10 - John Moody - High-Performance Persistent Graphs
1:15-1:35 - Nick Kramer - An Introduction to Logic Algebras
*
Thursday, March 31st at 3pm
TSC 229
Speaker: Robin Wilson, Emeritus Professor at Open University UK
Title: "A century of graph theory"
Rated: PG-13 (A lot of undergraduate mathematics or computer science knowledge assumed.)
Abstract: This talk covers the period from around 1890, when graph theory was mainly a collection of isolated results, to the 1990s when it had become part of mainstream mathematics. Among many other topics it includes material on the four-colour problem, trees, graph structure, and graph algorithms. No particular knowledge of graph theory is assumed.
*
Friday, March 25th at NOON
TSC 122 Lecture Hall
PIZZA PROVIDED
Speaker: Robin Wilson, Emeritus Professor at Open University UK
Title: "Stamping Through Mathematics"
Rated: G (No Mathematics knowledge assumed)
Abstract: In this talk, I shall cover the entire history of mathematics in one hour, illustrating the narrative with 300 postage stamps from around the world and illustrating a wide range of mathematics and mathematicians. Some of these stamps are highly bizarre and amusing. This talk is aimed at a general audience.
*
**************************************************************************************
BLOCK 6 - February/March 2016
Friday, March 4th at 2:30pm
TSC 229
Speaker: Visiting Assistant Professor Molly Moran
Title: "Baumslag-Solitar Groups and their Boundaries"
Rated: PG-13 (A lot of undergraduate mathematics or computer science knowledge assumed.)
Abstract: In 1962, Gilbert Baumslag and Donald Solitar introduced a family of groups, BS(m,n), that are simple to describe and yet provide very interesting examples and counterexamples in group theory. We will discuss Baumslag-Solitar groups, not from an algebraic standpoint, but rather we will describe how we can think of these groups (and any finitely presented group) as geometric objects and discuss why these groups also provide interesting examples in this new setting. In particular we will discuss how to construct the boundary for Baumslag-Solitar groups, which provides many new examples of Z-structures.
Joint work with Craig Guilbault (University of Wisconsin-Milwaukee) and Carrie Tirel (University of Wisconsin-Fox Valley).
*
Friday, February 26th at NOON
FearlessFriday Student Level Talk
TSC 122 Lecture Hall
PIZZA PROVIDED
Title: "Are you a topological pool shark?"
Rated: PG (Some undergraduate mathematics or computer science knowledge assumed.)
Abstract: The classic game of pool is played on a flat table. What other kinds of surfaces make sense to play on? What about a torus? Could YOU make a shot on a torus? This talk will be an interactive exploration of the topological properties of a surface that may (or may not!) make it desirable for pool.
*
Thursday, February 25th at 3pm
Research Talk
TSC 229
Title: "Pretzel knots: Slices, Ribbons, and Mutations"
Rated: R (A lot of undergraduate mathematics or computer science knowledge assumed.)
Abstract: A long-standing open conjecture, the Slice-Ribbon Conjecture, posits the equivalence of two types of knots: slice knots and ribbon knots.* While many (most?) mathematicians seem to believe that the conjecture is false, all empirical evidence points to it being true. In 2011, Greene and Jabuka showed that the Slice-Ribbon Conjecture holds for the infinite family of odd 3-stranded pretzel knots. In their paper, they give a complete characterization of the slice/ribbon knots in that infinite family. The speaker will give an overview of her recent work regarding the Slice-Ribbon Conjecture for the class of odd 5-stranded pretzel knots. The two extra strands in this case add a level of complexity not seen in the 3-stranded case, specifically with regard to knot mutation. In fact, the main result states that if an odd 5-stranded pretzel knot is slice, then it is mutant to a ribbon knot.
*Slice knots, ribbon knots, pretzel knots, and knot mutation will all be defined in the talk.
*
Friday, February 19th at NOON
TSC 122 Lecture Hall
PIZZA PROVIDED
Title: "Graphs and Matrices and Zombies "
Rated: PG (Some undergraduate mathematics or computer science knowledge assumed.)
Abstract: A simple graph is an ordered pair G=(V,E) where V is a set of vertices and E is a set of edges, which are two-element subsets of V. The maximum nullity of G, denoted M(G), is the largest possible nullity over all real symmetric matrices whose ij-th entry (for i,j distinct) is nonzero whenever {i,j} is in E and is zero otherwise. Maximum nullity can be difficult to calculate on its own, but can be estimated using another graph parameter called the zero forcing number. The zero forcing number of G, denoted Z(G), is the size of a minimum zero forcing set in G and can be found by playing a color-changing infection game on the vertices of G. In this talk, I will introduce the concept of zero forcing and discuss its usefulness with regard to calculating the maximum nullity of a graph.
*
Thursday, February 18th at 3pm
TSC 229
Title: "A random version of the r-fork-free theorem"
Rated: R (A lot of undergraduate mathematics or computer science knowledge assumed.)
Abstract: Let P(n) denote the set of all subsets of [n] and let P(n,p) be the set obtained from P(n) by selecting elements independently at random with probability p. The r-fork partially-ordered set, or poset, is the family of distinct sets F, G_1,...,G_r such that F \subset G_i for all i. De Bonis and Katona showed that, for fixed r, any (r+1)-fork-free family in P(n) has size at most (1+o(1)){n\choose \lfloor n/2\rfloor}. In this talk, I will discuss a similar result for (r+1)-fork-free families in P(n,p). In particular, if pn\to\infty, then with high probability, the largest (r+1)-fork-free set in P(n,p) has size at most (1+o(1))p{n\choose \lfloor n/2\rfloor}. This result is influenced by the work of Balogh, Mycroft and Treglown, who proved a random version of Sperner's theorem using the hypergraph container method.
*
**************************************************************************************
BLOCK 5 - January/February 2016
Friday, February 5th at NOON
TSC 122 Lecture Hall
PIZZA PROVIDED
Title: "The What and Why of Software Engineering"
Rated: PG (Some undergraduate mathematics or computer science knowledge assumed.)
Abstract: Software systems are growing in complexity as they grow in criticality. The stakes are high for software developers-software failures can have serious consequences-loss of money, loss of market, and even loss of human life. Software engineering processes are mechanisms for developing software systems with the goal of producing high quality software while managing time and budgetary concerns. This talk provides a high level introduction to software engineering by introducing the critical software engineering activities and describing the two main categories of software processes used to manage them.
*
Thursday, February 4th at 3pm
TSC 229
Title: "Using Text Mining Techniques to Extract Rationale from Unstructured Text"
Rated: PG13 (A lot of undergraduate mathematics or computer science knowledge assumed.)
Abstract: Design rationale has been an active area of research for over 30 years. The reasons for interest are clear-rationale captures the decisions, solutions, and intent during design. Still, rationale capture and use have not made the transition from research to practice. A critical issue is capture-rationale capture is viewed as expensive and tedious. Still, while rationale is not explicitly captured, that does not mean that rationale is not captured at all-decisions are documented in many ways throughout the development process. In this talk I will describe our investigation into techniques to identify and extract rationale from existing documentation. We have been experimenting with different text mining algorithms as well as comparing the results of using different features as the basis of classification, most recently using genetic algorithms to search for optimal feature combinations. Our results have accuracy similar to that achieved by humans attempting the same task.
*
Tuesday, February 2nd at NOON
TSC 122 Lecture Hall
PIZZA PROVIDED
Title: "Sequence alignment: From brute force to BWA"
Rated: PG (Some mathematics or computer science knowledge assumed.)
Abstract: In this talk I will introduce the sequence alignment problem, particularly as it arises in genome sequencing, describe the dynamic programming algorithms that made sequence alignment tractable and conclude with the data structures and algorithms used in modern aligners, such as BWA, to efficiently align the billions of short reads produced by modern sequencing instruments.
*
Monday, February 1st at 1pm
TSC 229
Title: "From GPUs to genomes: Enabling large-scale biological data science"
Rated: PG - PG13 (Some to a lot of mathematics or computer science knowledge assumed.)
Abstract: Driven by new instrument technologies, the life sciences are experiencing an ongoing data explosion. That data is creating a corresponding need for new computational tools that can help scientists, clinicians and other domain experts extract meaningful insights and do so at scale. In this talk I will describe example applications from my past work in systems biology and my current work in medical genomics that demonstrate how innovations in high-performance computing and other related domains can be adapted to solve important data analysis challenges in the life sciences.
*
Friday, January 29th at Noon
TSC 122 Lecture Hall
PIZZA PROVIDED
Title: "Algorithms for Aggregating Inconsistent Information"
Rated: PG (Some mathematics or computer science knowledge assumed.)
Abstract: We have the ability to gather enormous amounts of data, and there are numerous challenges in organizing this data into usable information. One of the questions that arises is the question of how to combine inconsistent information from different sources: for example, how do rank movies based on user's ratings? How should we combine recommendations to a user based on different criteria into one ranking?
Can we get better search engines by combining the results of different algorithms? These problems are instances of the rank aggregation problem, which has a long history. In this talk, we will give an algorithm for this problem that efficiently finds a solution that is provably close to optimal. The key ideas in our analysis can also be used to give provably good algorithms for problems where we want to organize data into clusters, for example clustering documents based on similarity scores, and clustering genes based on microarray experiments.
*
Thursday, January 28th at 3pm
TSC 229
Title: "The Traveling Salesman Problem"
Rated: PG-13 (A lot of mathematics or computer science knowledge assumed.)
Abstract: The traveling salesman problem (TSP) is perhaps the most famous problem in combinatorial optimization: given a set of cities and the distances for traveling between each pair of cities, the goal of the problem is to find the shortest tour that visits each city once and returns to its starting point. Besides applications in routing, algorithms for the TSP have been used in areas as diverse as finding the most efficient way to drill circuit boards to mapping genomes.
Finding an optimal tour is known to be NP-hard; nevertheless, the past decades have seen great progress in solving real-world instances of the TSP. A starting point for solving TSP instances is a linear programming relaxation called the subtour LP. The subtour LP is known to give excellent lower bounds on TSP instances in practice, however, its theoretical worst-case behavior (the "integrality gap'') is not well understood. A famous conjecture states that the integrality gap of the subtour LP is at most 4/3. In this talk, we will discuss some recent new algorithms and results for certain cases of the TSP, that can be seen as steps towards resolving this conjecture.
*
Friday, January 22nd at Noon
TSC 122 Lecture Hall
PIZZA PROVIDED
Title: "Lines on polygon surfaces"
Rated: G (No mathematics or computer science knowledge assumed.)
Abstract:If you glue together opposite edges of a square, you get a torus (the surface of a donut). What happens if you travel forever in a straight line on the square torus surface? How can you keep track of where you've been? I'll discuss this question for the square torus, and for other surfaces made from polygons, and I'll explain the surprising connection to continued fractions. There will be lots of pictures, and a dance video.
*
Thursday, January 21st at 3:00pm
TSC 229
Title: "Veech surfaces and the Modules Miracle"
Rated: PG (A little undergraduate mathematics or computer science knowledge assumed.)
Abstract: A square has reflectional and rotational symmetries. The square torus surface has an additional symmetry, a shear. I will explain what this is good for, and I will show you that amazingly, many other surfaces also have these three symmetries.
*
**************************************************************************************
BLOCK 4, 2015 - November/December
Friday, December 11th at 2:30pm
TSC 229
Speaker:Andy Glen
Title: "Goodness of Fit: Memoryless No Hitters"
Rated: PG-13 (A lot of undergraduate mathematics or computer science assumed.)
Abstract: This talk will be a primer on Goodness of Fit statistical testing with applications to "No Hitters" in major league baseball. The empirical distribution function will be introduced and used as means to understand various goodness of fit methods to include: Chi-square techniques, Kolmogorov-Smirnov techniques and most importantly Anderson - Darling techniques. A review of the exponential distribution and the memoryless property will follow. Finally we will show that time between no hitters in baseball is adequately fit by the exponential distribution, thus giving us a very intuitive example of the memoryless property, a rare enough occurrence in real world data. Other rare events also have this property.
*
Friday, December 4th at NOON
TSC 122 Kresge Lecture Hall
Pizza is Provided and there will be a recruitment session for the CU Boulder graduate CS program immediately after the talk.
Speaker: Dr. Sriram Sankaranarayanan, CU Boulder, Computer Science
Title: "Towards a Verified Artificial Pancreas"
Rated: PG (A little undergraduate mathematics or computer science assumed.)
Abstract: We describe our ongoing research on the mathematical modeling and analysis of artificial pancreas controllers. The artificial pancreas project is developing a series of increasingly sophisticated, software controlled devices that will automatically control insulin infusions to patients with type-1 diabetes in order to manage their blood glucose levels. On one hand, the device promises to greatly ease the process of managing blood glucose levels for people with type-1 diabetes. However, the device can potentially malfunction due to a combination of software design bugs and adversarial usage conditions. Such malfunctions may expose the patients to dire consequences that include coma or even death. How do we convince ourselves that artificial pancreas devices are free of such malfunctions? This problem of verifying computer systems is one of the grand challenges of computer science as a whole, and is well known to be undecidable in its full generality. We describe the process of mathematically modeling the artificial pancreas closed loop to simulate real-life situations directly inside a computer. Next, we describe a simulation-based approach to automatically explore the behaviors of these models to isolate interesting, potentially life threatening behaviors. While this approach does not completely automate the verification process, it provides useful results when applied to some artificial pancreas control algorithms. We conclude by emphasizing the need for rigorous mathematical modeling combined with computational analysis of these models to combat the potential risks posed by increased automation in areas such as robotics, transportation and medicine.
*
Friday, November 20th at 2:30pm
TSC 229
Speaker: Michael Penn
Title: "The Universality of the Virasoro Algebra in Mathematics and Physics"
Rated: PG-13
Abstract: The study of representation theory is, in essence, the reduction of the study of abstract algebraic structures to linear algebra - the study of vector spaces and linear transformations. The Virasoro algebra finds its way into the representation theory of all suitably interesting Lie algebras. During this talk, we will explore the Virasoro algebra by its definition, its appearance in the representations of other Lie algebras, and its applications to the seeming disparate subjects of number theory and physics.
This talk will provide all of the necessary definitions and consider several in depth examples, a strong knowledge of linear algebra is all that is required for full understanding.
*
**************************************************************************************
BLOCK 3, 2015 - October/November
Friday, November 6th, at 2:30pm
TSC 229
Speaker: Steven Janke
Title: "The Central Limit Theorem: More of the Story"
Abstract: Undergraduate probability courses present a version of the Central Limit Theorem published in 1810. Basically, it claims (under certain constraints) that the sum of several random variables converges to the normal distribution (bell-shaped curve). For statistics, one result of the theorem is that the sample mean is approximately normal. Yet, the common version of the theorem is really only the beginning of a series of results that start to explain why, for example, experimental errors are nearly normally distributed. This talk will trace these results, along with the development of the relevant analysis, and highlight the more recent connections with entropy and the second law of thermodynamics.
Rated: PG13 with R sections (A lot of undergraduate with some graduate mathematics or computer science assumed.)
*
Friday, October 30th, at 2:30pm
TSC 122 Kresge Lecture Hall
Speaker: Jeff Treviño, Assistant Professor of Music and Technology at CC
Title: "One Through Eight...Except Five: Problems and Potentials of Computationally Represented and Processed Music Notation"
Abstract: For over four decades, music researchers have attempted to computationally model common practice Western music notation and a canonic repertoire of symbolic manipulations thereof. I'll introduce some of the basic representational and processing challenges of this field (with an emphasis on rhythmic notation), review some of my research team's novel solutions, and finally demonstrate some of the artistic applications of Python programming in our team's creative work as composers.
Rated: PG
*
Friday, October 23rd, at NOON
TSC 122 Kresge Lecture Hall
Pizza Provided
Speaker: Amy Hepner '09
Title: "The Analytics of Social Progress."
Abstract: Come explore how the ideas of data science are being used to inform social progress efforts. Examples include: Predicting police officers at an increased risk of having an adverse interaction with the public; identifying students in danger of not graduating on time; improving environmental advocacy programs; identifying causes of home abandonment.
Mathematical concepts will be explained in everyday language. Career opportunities presented throughout.
Rated: G
*
**************************************************************************************
BLOCK 2, 2015 - September/October
Friday, October 9th at NOON
TSC 122 Kresge Lecture Hall
Pizza Provided
Speaker: Sarah Fletcher Mercaldo, Vanderbilt University
Title: "Best Practices for Constructing and Applying Clinical Prediction Models in the Presence of Non-Ignorable Missing Data"
Rated: PG-13
Abstract: Clinical prediction models use clinical and demographic information to predict the probability that a patient has a certain disease or clinical status. When patient data is missing, care must be taken in both constructing and applying the prediction model. In this presentation, I will introduce different types of missing data often observed in clinical and electronic medical record data. While certain kinds of missing data can be imputed successfully with simple or fancy imputation schemes, the most complicated case when the data are missing NOT at random presents substantial problems for researchers. Here I present several strategies for imputing data that are missing NOT at random and I evaluate and compare the performance of these strategies.
For demonstration, I will use the TREAT risk prediction model, which was developed from a cohort of 492 participants with known or suspected lung cancer evaluated for lung surgery. In this cohort 264 patients had complete data, and 228 patients were missing at least one predictor. When a physician is applying the TREAT model at the bedside to a patient, he may not know or have access to all the information the model requires, and thus how that data is imputed becomes critical to the application of the model. Prediction models such as the TREAT model are being used more frequently to inform clinical decisions. However, published prediction models cannot be applied when a patients clinical data is missing. Proper handling of missing data is key to developing a robust and accurate prediction model
*
Friday, October 2nd at NOON
TSC 122 - Kresge Lecture Hall
Pizza will be provided!
Speaker: Benjamin Ylvisaker
Title: "Google, The NSA and The Supreme Leader are Listening!"
Rated: PG
Abstract: There is an enormous amount of private data sent and stored insecurely over the Internet. Powerful governmental, corporate and/or criminal organizations are actively collecting and exploiting this data. This talk will introduce a broad range of concepts in
information security and privacy. In particular we will discuss new ideas related to storing data securely in public cloud storage services.
All majors must write up four talks in order to graduate. Talk write ups should be submitted through Canvas. If you wish to be added to the Canvas page, please contact one of the paraprofs.
*
Friday, September 4th at 2:30pm in TSC 229
Speaker: Darren Funk-Neubauer
Title: "Bidiagonal Pairs and Bidiagonal Triples."
Rated: PG-13 with R rated sections
Abstract: Roughly speaking, a bidiagonal pair is a pair of diagonalizable linear transformations on a finite-dimensional vector space, each of which acts in a bidiagonal fashion on the eigenspaces of the other. A classification of bidiagonal pairs will be presented. Then I will describe how to construct all bidiagonal pairs using representations of the Lie algebra sl2 and the quantum group Uq (sl2) . Next, I will explain how to extend the definition of bidiagonal pair to a triple of linear transformations, thus defining a bidiagonal triple. I will discuss in what sense a given bidiagonal pair determines a bidiagonal triple. Lastly, I will describe the classification of bidiagonal triples.
**************************************************************************************
BLOCK 1, 2015 - August/September
Departmental Research Overview and Ice Cream Social
Friday, September 4th at NOON
TSC 122 - Tutt Science Lecture Hall
Rated G
This general-audience talk will showcase the various research interests of the Department of Mathematics and Computer Science at Colorado College. Each faculty member will provide a brief description of their scholarly pursuits. Pizza will be provided!
Faculty speakers will include
• Marlow Anderson - Wild Automorphisms of the Complex Plane, History of Mathematics
• David Brown - Mathematical Biology
• Andrea Bruder - Differential Equations
• Rodney James - Tropical Geometry
• Steven Janke - Computational Complexity
• Molly Moran - Geometric Group Theory
• Stefan Erickson - Computational Number Theory and Cryptography.
Immediately after the talk, we will be hosting an ice cream social for all students in the department lounge on the second floor of Tutt Science
**************************************************************************************
**************************************************************************************
2014-2015
BLOCK 8, 2015 - April/May
Friday, April 24th at 2:30pm
TSC 229
Speaker: Andy Glen
Title: Computational Probability: Algorithms and Applications
Abstract: The open source computational package A Probability Programming Language (APPL) represents an ongoing 20 year effort at combining symbolic algebra software with probabilistic results that, in the end, can be used to automate a number of theorems from probability theory. This talk will give a demonstration of the language, discuss some of the computational issues associated with the project and give some examples of application of the software. APPL is one of a kind probabilistic software (differentiated from statistical software) that primarily manipulates functions, not numbers. Applications can be found in all areas of probability and statistics to include stochastic processes, reliability engineering, probabilistic models, statistical models, financial mathematics, and so forth.
Rated: PG-13
*
*********************
BLOCK 7, 2015 - March/April
SENIOR CAPSTONE TALKS
Wednesday, Thursday and Friday April 8-10
TSC 122 Lecture Hall
WEDNESDAY
1:00-1:25 Minqi Liu - A Three-species Model With Predator-prey, Competition,
And Mutualistic Interactions
Thesis Advisor: Andrea Bruder
1:30-1:55 Katy Martinez - Epidemiological Methods for Examining Bullying
Thesis Advisor: Andrea Bruder
2:00-2:25 Julia Napolitano - The Cantor set as a Fractal and its Applications in Art
Thesis Advisor: Marlow Anderson
2:30-2:55 BREAK
3:00-3:25 Gautam Webb -Generalization of the Heisenberg Algebra
Thesis Advisor: Michael Penn
3:30-3:55 Nicole Pey - From Markov Chains to Brownian Motion: Let's take a Random Walk
Thesis Advisor: Steven Janke
THURSDAY
1:00-1:25 Fanpei Liu - Introduction to Lebesgue Integral
Thesis Advisor: Fred Tinsley
1:30-1:55 Joe Howard - Using Hercules and the Hydra to Build Problem Solving Skills
Thesis Advisor: Michael Penn
2:00-2:25 Sam Spellman - Lobachevsky's Spherical Trigonometry
Thesis Advisor: Marlow Anderson
2:30-2:55 BREAK
3:00-3:25 Maggie Bailey - Epidemics and Network Structures: A Stochastic Study
Thesis Advisor: David Brown
3:30-3:55 Chris Van Dusen - Methods to prevent over-fitting and solve ill posed problems in statistics: Ridge Regression and LASSO
Thesis Advisor: Fred Tinsley
FRIDAY
1:00-1:25 Emma Holmes - Realistic Driver Behavior Characteristics on Microscopic Traffic Flow
Modeling
Thesis Advisor: David Brown
1:30-1:55 Emily Stearns - Modeling Ocean Waves: Linear Wave Theory
Thesis Advisor: Amelia Taylor, Andrea Bruder, & Rodney James
2:00-2:25 Lou Brand - Neural Networks, Genetic Algorithms, and the Blood Brain Barrier
Thesis Advisor: Matthew Whitehead
*
Friday April 3 at Noon
TSC 122 Lecture Hall
Pizza Provided!
Speaker: Ben Ylvisaker
Title: Persistent Data Structures
Abstract: In the vast majority of the programming world, modifying a data structure results in the "destruction" of the previous version. With persistent data structures, it's easy for a program to keep multiple versions of a structure simultaneously. In this talk we'll cover why this is a useful idea and how it's possible to implement with not-totally-ridiculous memory and time overhead.
Rated: PG
*
Friday March 27 at 2:30pm
TSC 229
Speaker: Rodney James
Title: Real Sandpile Groups
Abstract: Sandpile models were first introduced by physicists in the 1980's as a way to study the dissipative dynamics of discrete systems on a lattice. In this model, grains of sand are placed on the vertices until a critical number of grains is present, at which point an avalanche occurs and the pile of sand topples over to its neighbors. Certain stable configurations of sand resulting from avalanches were later shown to form an abelian group. In this talk we will extend the sandpile model to real-valued quantities on each vertex, and show that there are stable configurations of these real-valued sandpiles that form a different abelian group.
Rated: PG-13
*********************
BLOCK 5, 2015 - January/February
Friday February 6 at 2:30pm
TSC 229
Speaker: Kristen Walcott Justice (UCCS)
Title: Machine Learning in Software Test Prioritization Techniques
Abstract: TBA.
Rated: PG-13
*
Friday February 6th at Noon
TSC 122 Tutt Lecture Hall
Pizza provided!
Speaker: Molly Moran
Title: Triangles: Using a Basic Shape to Understand Modern Goemetry
Abstract: TBA
Rated: G
*
Thursday February 5th at Noon
TSC 229
Speaker: Molly Moran
Title: A Look at Group Boundaries and their Dimension
Abstract: TBA
Rated: R
*
*********************
BLOCK 4, 2014 - December
Friday December 12 at Noon
TSC 122 Lecture Hall
Pizza provided!
Speaker: Marlow Anderson
Title: Recent Progress on the Twin Prime Conjecture
Abstract: The Twin Prime Conjecture asserts that there are infinitely many pairs of primes separated by 2, like 3 & 5, 5 & 7, 11 & 13, and so on. In almost two centuries, there has been no real progress in proving this. However, in May of 2013, Zitang Zhang thrilled the mathematical world by announcing a proof that there are infinitely many pairs of primes separated by no more than 70,000,000. This is quite a bit bigger than 2, but it was the first such finite bound ever proved. In an exciting summer, a collaborative on-line team worked hard to reduce the bound, and we know now that there are infinitely many prime pairs separated by no more than 246. In this talk, I will describe the historical and mathematical background to this problem, and describe a bit about the recent developments.
Rated: PG
*
Friday, December 5 at 2:30 pm
TSC 229
Speaker: C. Edward Chow, Department of Computer Science University of Colorado at Colorado Springs
Title: Intrusion Tolerance and Cloud
Abstract: In this talk I will review the assumptions, ideas, and techniques developed for intrusion tolerance, which can be used to tolerate Distributed Denial of Service (DDoS) attacks, with the side effect of providing multiple alternate routes that improve performance and reliability. We will then discuss new ideas for utilizing cheap cloud resource for enhancing DDoS defense and building Secure Systems. With this new intrusion tolerance paradigm in mind, we have developed enhanced secure Domain Name System (DNS) for querying new evolving IP address mapping and multipath indirect routing using a set of proxy servers. The multiple paths established in this secure overlay network provide better bandwidth, higher reliability, and enhanced security.
Rated PG-13
*********************
BLOCK 3, 2014 - October/November
Friday November 14 at 2:30pm
TSC 229
Speaker: Chris Sadowski of Ursinus College
Title: Representations of Affine Lie algebras and Integer Partitions
Abstract: The Rogers-Ramunujan partition identities have many deep ties to the representation theory of affine Lie algebras. In this talk, we introduce these identities and recall some facts about them. Then, following the work of Feigin, Stoyanovsky, Calinescu, Capparelli, Lepowsky, and Milas, we discuss how these identities arise in the study of "prinicpalsubspaces" of the standard modules for the affine Lie algebra sl(2)^ and we also discuss generalizations to higher rank cases. It turns out that generators-and-relations results for these principal subspaces are crucial to this work. We describe new generators-and-relations results about the principal subspaces of the standard sl(3)^-modules and we give a conjecture for the corresponding presentations of the principal subspaces of the standard sl(n)^-modules for general n. We also provide new results for the principal subspaces of certain standard sl(n)^-modules, generalizing earlier work by Calinescu
Rated: PG-13
*
Friday November 7, Noon
TSC 122 Lecture Hall
Speaker: Steven Janke
Title: Shadows in Computer Graphics
Abstract: Constructing simple shadows for computer graphics scenes is a relatively straightforward geometry problem, but to make the procedure efficient and to deal with more complex cases, it helps to call on projective geometry. This talk will introduce some projective geometry ideas and show how shadows and perspective drawing are related. By using special coordinates (homogeneous), we can make it easier to draw more complex shadows on the computer screen.
Rated: PG
*
Friday Oct. 31, 2:30 pm
Tutt Science Center 229
Speaker: Molly Moran, University of Wisconsin-Milwaukee, CC Class of 2009
Title: How to Measure Distance between Two Points at Infinity: Metrics on the Visual Boundary of CAT(0) Spaces
Abstract: Two types of metric spaces that are widely studied in Geometric Group Theory are CAT(0) spaces and δ-hyperbolic spaces. Both spaces can be defined in terms of thinness of triangles. Somewhat surprisingly, the rather simple definitions turn out to capture the large-scale behavior of these spaces, in particular their curvature. In this talk we will define these spaces, discuss their boundaries at infinity, and how we could measure distances between points in the boundary.
Rated: PG-13
*********************
BLOCK 2, 2014 - October
Friday Oct. 17, 3:00pm
Tutt Science Center 229
Speaker: Kathy Merrill
Title: Wavelet sets for Determinant ±2 Matrices
Abstract: A wavelet set is a set that tiles ℝn under both translation by the integer lattice and dilation by a matrix. I recently discovered a proof that all determinant ±2 matrices have wavelet sets that are simple in the sense of being finite unions of convex sets. This talk will describe what is special about determinant 2, and then present the main ideas of this proof.
Rated: PG-13
*
Friday October 10th, Noon
Tutt Science 122 - Kresge Lecture Hall
Pizza will be served!
Speaker: CC alumna Sarah Wolff
Title: A Random Walk through Algebra
Abstract: How many shuffles does it take to completely randomize a deck of cards? This simple question, answered by Persi Diaconis in 1986, opens up an entire world of mathematics: the theory of randomness. In this talk, we will develop and analyze the random walk created by shuffling a deck of cards and then apply what we've learned to other interesting settings. The theory of randomness has applications not only in card shuffling but also economics, genetics, computer science, psychology, and much more!
Rated: G
*
Friday October 3rd, 2:30pm
Tutt Science 229
Speaker: Fred Tinsley
Title: Group embeddings, recursiveness (logic, argh!), and topology
Abstract: In the early 1960's Graham Higman characterized those finitely generated groups that embed in finitely presented groups. [i] This amazing theorem pinpointed those finitely generated groups whose relation set is recursively enumerable as precisely those groups that embed in some finitely presented group. Such groups are called recursively presented. In obtaining this result, Higman "firmly established that the connection between the logical notion of recursiveness and questions about finitely presented groups is not accidental but very deep". [ii] Of course, these concepts also are important in theoretical computer science. But to a topologist such as me, finitely presented groups are quite desirable as they are naturally the fundamental groups of high-dimensional, closed manifolds (surfaces) while at the same time are potentially better understood using computational methods (computers). We will investigate whether the necessarily universal techniques used by Higman (and others since) can be helpful in obtaining embeddings of specific recursively presented groups that are important to a project in which I am currently involved.
[i] Higman, G: "Subgroups of finitely presented groups", Proc Royal Soc London Ser A 262, 455-475 (1961)
[ii] Lyndon, R, Schupp, P: Combinatorial Group Theory, Springer-Verlag, Berlin Heidelberg New York (1977), p 214.
Rated: PG13 (though X in some places)
*********************
BLOCK 1, 2014 - September
Friday September 19th, 3pm
Tutt Science Second Floor Skilling Commons
This week, on Sept. 19, we will have a special edition of Fearless Friday. Some of our students who did research projects this past summer (either here or elsewhere) will be presenting their work in a small poster session. There will be approximately 7 posters, on topics from pure and applied math and computer science . There is a special time and location: 3:00 - 4:00 pm, in the 2nd floor open area ("Skilling Commons") of Tutt Science. Light refreshments will be served.
- Lou Brand: Genetic algorithms, neural networks, and the blood-brain barrier
- Emma Holmes: Modeling microscopic and macroscopic traffic flow utilizing the particle filter and the ensemble Kalman filter
- Melissa Jay: Speech intelligibility index model: A key aspect to a child's development of speech and language
- Nick Kramer: Multi-valued logics and their algebras
- Minqi Liu: A three-species model with predator-prey, competition, and mutualistic interactions
- Katy Martinez: How to prevent bullying: A mathematical approach
- Denali Molitor: Self-organized criticality for optimal random search
*
Friday September 12th, Noon
TSC 122 and Math Dept Lounge
Ice cream social and Dept Research Overviews
Come join the Mathematics and Computer Science department with the annual welcome back event, where every professor will be giving a brief overview of their research. Topics covered will range from applying abstract algebra to evolutionary biology to topology and designing robust cellular automata. This is a chance for returning students to reconnect with faculty and peers, and new students to introduce themselves and discover what's happening. It's also a chance for majors to consider research alongside their professors.
Rated: G - PG13
*
Friday September 5th, 2:30pm
TSC 229
Speaker:Michael Penn
**************************************************************************************
**************************************************************************************
2013-2014
BLOCK 8, 2014 - April/May
Friday May 9, 2:30pm
Tutt Science 229
Speaker: Stefan Erickson
Title: Computer Security in the Post-Snowden Era
Abstract: The Snowden leaks about NSA spying have sparked a public debate about cybersecurity. There are many questions that people are struggling to answer. What is privacy? What is security? What is the right balance between individual privacy and national security? What will the effect of "big data" have on Internet commerce and security? The answer to these questions will inevitably have a huge impact on the future of society.
In this talk, we will explore many aspects of cybersecurity. We will explain how the Target Point-Of-Service breach occurred, what the Stuxnet worm is and why it was important, and how the recent "Heartbleed" bug worked. We will see what information the NSA has been gathering and how they exploited a weak random number generator to break a commonly used cryptosystem. We also hope to start a dialogue about the proper role of computer security. The talk is mostly rated G with occasional flashes of PG-13 material.
90% Rated G, 10% Rated PG13
Friday May 2, Noon
Tutt Science 122 - Lecture Hall
Speaker: Jim Powell, University of Utah (visiting professor)
Title: Mathematics of the Life-Impaired: How Disease Theory Predicts the Zombie Apocalypse
Abstract:
From movies to pop music ("If I were a zombie, I'd never eat your brain...), it seems the undead are already taking over the world. The usually staid Centers for Disease Control launched a tongue-in-cheek "Preparedness 101: Zombie Apolcalypse" public campaign in 2011 to drive home the importance of emergency preparation. Even Utah State University has been infected as evidenced by USU Housing's wildly popular, campus-wide"Humans vs. Zombies" (HvZ) war this past fall.
Anthropologist Krystal D'Costa suggests zombies capture our imagination because they represent modern society and technology gone awry and offer the perfect metaphor for an unstoppable pandemic. USU professor Jim Powell expands the zombie metaphor to illustrate the concepts and results of mathematical epidemiology. Using storylines from such movies as "Night of the Living Dead," "28 Days Later," "The Walking Dead" and "I am Legend," as well as data from the USU HvZ games, Dr. Powell will show how mathematicians model diseases. He'll talk about how scientists predict the course and impact of epidemics, discuss how "herd immunity" (vaccination levels for disease eradication) works and apply some of these modeling strategies to the understand and predict the spread of Chronic Wasting Disease (Zombie Deer) in southern Utah.
Rated PG
Friday April 25, Noon
Tutt Science 122- Lecture Hall
Speaker: Gene Abrams, University of Colorado at Colorado Springs
Title: Fibonacci's Rabbits Visit the Mad Veterinarian
Abstract: Since its origin (more than eight centuries ago) as a puzzle about the number of rabbits in a fantasmagorically expanding) colony, the Fibonacci Sequence 1,1,2,3,5,8,13,... has arguably become the most well-known of numerical lists, due in part to its simple recursion formula, as well as to the numerous connections it enjoys with many branches of mathematics and science.
Since their origins (less than two decades ago) as puzzles about the number of animals in a(fantasmagorically strange) veterinarian's oce, the (not so well-known) Mad Vet Scenarios have provided a source of thought-provoking entertainment to internet gamers and math enthusiasts alike. In this talk we'll show how Fibonacci's puzzle about rabbits is naturally connected to the puzzles found in the Mad Vet's oce. Along the way, we'll show how an investigation into Mad Vet Scenarios has led to the discovery of some heretofore unrecorded properties of the Fibonacci Sequence.
Rated G. This talk is rated G, meaning that it is intended for the most General of audiences. No prior familiarity with Fibonacci's breeding rabbits, or with the Mad Veterinarian's transmogrication machines, or with any other type of fantasmagorical animal population dynamics, will be assumed.
Pizza wiill be served.
BLOCK 7, 2014 - March/April
Wednesday April 9 AND Thursday April 10, 1:30-3:30
Tutt Science 122- Lecture Hall
Student Capstone Talks!!
Light refreshements will be served.
...
Friday March 28 at NOON
Tutt Science 122 - Lecture Hall
Speaker: Loren Cobb
Title: War, or Peace, or What? A Math Clinic Studies the 21st Century
Abstract: This talk will present the results of a semester-long mathematics clinic on predicting the fate of human society in the Twenty-First Century. This clinic, a required course for both undergraduates and graduate students in UC-Denver's Math Department, focused on what we can deduce about the coming century from the use of interlocking dynamic models of population growth, resource exhaustion (food, water, and fossil fuels), urbanization, economic growth, governance, education, and policy optimization. The results were surprisingly positive, although several very specific areas of severe conflict and human misery were identified.
BLOCK 6, 2014 - February/March
Friday March 7 at NOON
Tutt Science 122 - Lecture Hall
Speaker: Marlow Anderson
Title: CYCLOID WARS: A case study in 17th century mathematics.
Abstract: The cycloid is a curve known as the "apple of discord", because it produced so much controversy and debate among 17th century mathematicians. We will examine this history, with particular attention paid to how these debates illustrate the evolving 17th century answers to the following questions: What is geometry? What is a curve? What is a proof? Almost all of the great mathematicians of the century play a role, from Galileo and his students Torricelli and Cavalieri; to the French mathematicians in the Mersenne circle such as Roberval, Descartes and Fermat; to Leibniz, his mentor Huygens, and his students the Bernoulli brothers; and to Newton and his English predecessors Wren and Wallis.
Rated PG
Friday February 28 at NOON
Tutt Science 122 - Lecture Hall
Speaker: David Brown
Title: The Power of Positive Feedback
Abstract: Many biological (and other) systems can be understood in terms of linked positive and negative feedback loops. Over the past 10 years, a common theme in much of my own research has been the role of positive feedback in driving interesting dynamics in biological systems. Here, positive feedback refers to self-reinforcing dynamical processes. I will show how feedback diagrams can be turned into mathematical models consisting of differential equations. I will then show how positive feedback can cause phenomena like bistability, rapid decision making, and spatial pattern formation. I will illustrate these ideas with examples from my research on predator-prey dynamics, chemical communication between bacteria, and the dynamics of tree line on Pikes Peak.
Rated: PG/PG-13. Familiarity with differential equations is assumed, but the technical details will be kept to a minimum.
BLOCK 5, 2014 - January/February
Friday, February 7 at Noon
Tutt Science 122 Lecture Hall
Title: Finite State Automata
Abstract: Automata Theory is an exciting, theoretical branch of computer science. Simply stated, automata theory is the study of abstract computing devices or "machines". Through automata, computer scientists are able to understand how machines compute functions and solve problems and more importantly, what it means for a function to be defined as computable or for a question to be described as decidable. Before there were computers in 1930's A Turing studied an abstract machine that model the power of real computers. Turing machine allow us to describe precisely what a computing machine could do and could not do. The simplest automata used for computation is a finite state automaton, also called a finite state machine. Finite automaton is a basic model of computational systems with finite memory. It is much more restrictive in its capabilities than a Turing machine. Finite state machines originally proposed to model brain functions, turned out to be extremely useful for variety of other purposes. They can be used to recognize a type of formal languages called regular languages and used for building certain types of software including lexical analyzers. This lecture will provide an introduction to theory of computation and finite state automata.
Rating: PG
Friday, January 31 at Noon
Tutt Science 122 Lecture Hall
Title: All Your Code Are Belong to Us
Abstract: Software today exists in a hostile environment. Vandals, thieves, hacktavists, and various government agencies are hard at work subverting computer systems of all sorts. Understand the common attack vectors and what can be done to defend against them is important for nearly everyone in modern society (to different depths, depending on one's role, of course). In this talk we will take a guided stroll through the Common Weakness Enumeration Top 25 software errors. We will cover the basics like stack overruns, script injections and public key cryptography or related topics, according to audience interest.
Rating:
20% G
60% PG
20% PG13
Friday, January 24 at Noon
Tutt Science 122 Lecture Hall
Speaker: Jim Boerkoel from Harvey Mudd College
Title: Helping Human-robot Teams Stay Coordinated
Abstract: An emerging trend in robotics is the development of inherently safe, mobile robots that are designed to assist humans across a wide variety of tasks. Humans are good at intuitively perceiving and quickly adapting to the intentions and conventions of their human teammates with little or no explicit communications. Robots, on the other hand, face the challenge of optimizing and adapting their schedules to, what to them appears to be, their inherently unreliable, non-communicative, and irrational human teammates. A primary goal of my work is to equip robots with methods to quickly and robustly develop plans that (1) adapt to established conventions to successfully negotiate human-oriented environments and (2) augment the workflow of human workers to increase overall productivity, safety, and quality. In this talk I discuss a new, distributed robotic scheduling approach that builds on classical shortest-path algorithms to enable a robot to coordinate its activities with its human teammates while providing them flexibility and autonomy.
Rated PG: A little undergraduate mathematics assumed.
BLOCK 4, 2013 - December
Friday, December 13 at 2:30 pm
Tutt Science 229
Speaker: Jonathan Portiz
Title: Computation in Nearby Universes
Rated: mostly PG with brief interludes of PG13/R (A little to a lot of undergraduate mathematics or computer science assumed.)
Abstract:
When first introduced, the Turing Machine (TM) seemed an entirely non-physical formalization of computation, but insights from thermodynamics and quantum mechanics showed this was not entirely true. The good news was the discovery of algorithms for quantum TMs which were much faster than the best classical ones -- even breaking important modern cryptographic algorithms. I will describe some of these physical influences on computation, including a brief introduction to quantum computation (which could be useful since all signs point to the imminent arrival of actual quantum computers). I will then propose a way to vary the "structure group" underlying the physics of TMs, in essence to do computation on other universes. A surprising mathematical connection is the relationship of probabilistic computation with some beautiful classical linear algebra.
BLOCK 3, 2013 - November
Friday, November 15 at 2:30 pm
Tutt Science 229
Speaker: Charles Morgenstern, CC alum, and graduate student at the Colorado School of Mines.
Title: Acoustic Wave Scattering with FEM-BEM
Rated: PG-13 (A lot of undergraduate mathematics or computer science assumed.)
Abstract:
This talk is a brief investigation into numeric methods for approximating time harmonic acoustic wave scattering in two-dimensions by bounded inhomogeneities and impenetrable objects. First boundary element methods (BEM) and finite element methods (FEM) are considered alone, and then a new method which couples these two is presented. Using the coupled method global solutions can be computed for problems too complex for a boundary element method alone. Parallel and serial computing performance as well as convergence for the method will be discussed. This is a joint project with Prof. Mahadevan Ganesh at the Colorado School of Mines and is supported by the National Science Foundation.
***
Friday, November 8 at 12 pm
Tutt Science, Kresge Lecture Hall (TSC 122)
Speaker: Matthew Whitehead
Title: Can Robots Build Robots that Build Robots?
Rated: G
Abstract:
Imagine a large warehouse filled with spare electronic and mechanical parts. Is it possible to build a robot that goes into the warehouse and builds an exact copy of itself? If so, then those copies could build more and more robots until the building materials were exhausted.
This talk will explore the computational requirements of machine self-replication using cellular automata. After seeing several examples of simple self replicators, we will then consider replicating Turing-complete machines: machines that can compute any computable function.
***
Friday, November 1, 2:30pm
Tutt Science 229
Speaker: Dr. Gerald de Souz, PhD from The University of Albany, Albany - New York
Title: From Fourier Analysis to Wavelet Analysis
Rated: PG-13
Abstract: In this talk, we discuss the origin of Fourier and wavelet analyses and explain the connections between them. In fact, in this presentation we will discuss the following:
How much information is needed to reconstruct a situation (signal)? And how we accomplish it? If a situation (signal) is represented by a function f(x). How can we decompose this function f(x) into "simpler" functions and from these "simpler functions" recovery all necessary information about f(x)? - Building block functions.
Assume we have too much information about a given situation (signal). How can we throw away some of these information and still have a complete necessary information about the situation (signal)? Compression
This is an informative talk and it is suitable for a general audience including students. My friend and late Paul Halmos often advised colloquium speakers "to make the first 15 minutes of their talks accessible to the entire audience. Do you know how difficult that is?" Notices of the AMS, February 2009.
I will try to follow his advice.
"Mathematics contains ideas that can be, and deserve to be, communicated to the wider public - even if what is communicated is at the level of appreciation rather than practical knowledge." - Barbara Burke Hubbard, The World According to Wavelets, 1996.
A bit about our speaker: Geraldo Soares de souza, PhD from The University of Albany, Albany - New York in the area of Harmonic Analysis. He is currently a full Professor of Mathematics at Auburn University in Auburn, Alabama. He has had several Master and PhD students and publications in his area of expertise. For over 25 years, he has led a series of conferences in Harmonic Analysis and Related Areas at Auburn University sponsored by NSF. He and his colleagues have led several international projects in Africa, South America, Central America, etc. Recently, he and some colleagues have been awarded nearly 500 thousand dollars from NSF and other agencies to create a more robust infrastructure to support mathematics education in Africa.
BLOCK 2, 2013 - October
Denali Molitor, Hanson Smith and Ravi Donepudi (three of our current students) will give a PG rated talk on their research.
Time: 2pm (Note this is not a usual time for us!)
Location: Kresge Lecture Hall, Tutt Science 122 Reception to Follow.
Titles: Fractal Analysis, Equal Circle Packing on Flat Tori, Rational Numbers and Function Fields: Similarities and Differences
Fractal Analysis (Denali Molitor): The real world is full of rough surfaces, which poses the challenge of how to represent and study these surfaces mathematically. Fractal analysis, although it still poses unrealistic structural restrictions on these rough surfaces, provides a first step toward this goal. By defining Laplacians on fractals, we can also then study how some functions behave on these rougher spaces. This presentation will focus on how space-filling Peano curves can be used to define Laplacians on certain classes of fractals by defining a way to continuously trace through subsequent graph approximations of the fractals.
Equal Circle Packing on Flat Tori (Hanson Smith): The study of maximally dense packings of disjoint equal circles in di fferent types of containers is a problem in Discrete Geometry that has developed over the past forty years. The optimal densities and arrangements are known for packings of small numbers of equal circles in hard boundary containers, including squares, equilateral triangles and circles. In this presentation, I will explore packings of small numbers of equal circles in a boundaryless container called a flat torus. We will introduce the basic concepts (including the notion of a flat torus, an optimal packing and the graph of a packing), demonstrate many maximally dense arrangements for four equal circles, and outline a proof of their optimality. This research was conducted as part of the 2013 REU program at Grand Valley State University.
Rational Numbers and Function Fields: Similarities and Differences (Ravi Donepudi) : Function fields are interesting analogs of the familiar rational numbers. Although these two objects appear very different at a first glance, they have many properties in common. Many problems that are difficult to solve in the rational numbers become very simple in this new setting. Among the most famous of these is the Riemann Hypothesis, which we will prove in this setting. I also present work I did over the summer relating to extensions of these function fields. There should be something for everyone at this talk. Please pass the word along to anyone who might be interested!
Rating: PG
**************************************************************************************
**************************************************************************************
2012-2013
BLOCK 7, 2013 -April
Friday April 12th
Noon in TSC 122 Tutt Science Lecture Hall
Colin Weir, University of Calgary
Title: What the L? An Intro and Application to L-functions
Abstract: The main goal of this talk is to introduce Artin L-funtions, a generalization of the Riemann zeta function, by wandering various realms in the world of mathematics. These will include small secluded islands within the lands of number theory, abstract algebra, representation theory, and complex analysis. Our travels will eventually lead us to the promised land hidden in the very depths of math world - the fountain of eternal theorems. It is here where we will unravel the mysteries of L and harness the power to produce an infinitude of number theory results.
Rating: PG
*********************
BLOCK 6, 2013 - February & March
Monday March 11
Noon - TSC 122 Lecture Hall
Emma Norbrothen (candidate for visiting professor position)
Title: Number Systems Base P
Abstract: Rational numbers can construct the real numbers by using the absolute value metric. Under different metrics, rationals can construct different types of numbers. In particular, the p-norm evaluates how much a prime, p, is a factor in a given rational. We will explore some consequences of the p-norm and what kind of numbers it creates rom the rationals.
Rating: PG
***
Monday March 4
Noon - TSC 122 Lecture Hall
Colin Weir (candidate for visiting professor position)
Title: When Primes Factor
Abstract: We learned in elementary school that prime numbers are numbers that only factor as 1 times themselves. However, this definition assumes that the only allowable factors are positive integers. What does it mean to be prime if we are allowed to write or ? How can a prime number factor if we extend our allowable factors beyond positive integers? We will explore these questions and many others, eventually leading us to areas of modern research in number theory and cryptography.
Rating: Light PG-13 (we will eventually review some abstract algebra)
Tuesday March 5
2:30 pm- TSC 229
Colin Weir (candidate for visiting professor position)
Title: Constructing and Tabulating Algebraic Function Fields
Abstract: Research into the construction of certain low degree function fields has surged in recent years, in part due to the cryptographic significance of elliptic and hyperelliptic curves. However there is comparatively little data available for higher degree function fields, leaving open many questions about the number of function fields of fixed degree and given discriminant. We present a Kummer theoretic algorithm for constructing degree ℓ dihedral function fields over a finite field with prescribed ramification. We then use this in a tabulation algorithm to construct all cubic function fields over up to a given discriminant bound and compare the data to known asymptotics. We will also survey other applications where these techniques can be made useful, such as constructing curves with many points, or in implementing cover attacks on hyperelliptic curve cryptography. Moreover, we show how our construction techniques can be extended to characteristic zero to construct interesting curves over number fields.
Rating: R (some Galois theory will be assumed)
***
BLOCK 5, 2013 - January & February
Friday February 8
Noon - TSC 122 Lecture Hall
Taylor McNeill (candidate for visiting professor position)
Title: Twisted Sisters - How a knot becomes a braid
Abstract: What do cancer cells, the sun's corona, and your kid sister's hair have in common? Knots! In this talk I'll give a brief introduction to knot theory, including a glimpse into its history and applications. After that we'll discuss braids and their correspondence with knots. Finally we'll show that every knot is a braid in disguise. So say "bye-bye" to those tangled earbuds and "hello" to low dimensional topology.
PIZZA will be served.
Rated: G
*
Thursday February 7
3:00pm - TSC 229
Taylor McNeill (candidate for visiting professor position)
Title: A new filtration of the Magnus kernel of the Torelli group.
Abstract: For a surface S, the mapping class group, Mod(S) is the group of orientation preserving homeomorphisms of S mod isotopy. A key subgroup of the mapping class group, the Torelli group admits a representation onto a linear group via the Magnus representation. For many years it was unknown whether the Magnus representation of the Torelli group is faithful. In recent years there have been many developments on this front including the result of Church and Farb that the kernel of the Magnus representation, denoted Mag(S), is infinitely generated. I show that, not only is Mag(S) highly non-trivial but that it also has a rich structure as a group.
Specifically, I define an infinite filtration of Mag(S) by subgroups, called the higher order Magnus subgroups, M_n. I show that for each n the quotient M_n/M_n+1 is infinitely generated.
Rating: PG-13
*
Friday January 25
2:30pm, TSC 229
Kathy Merrill
Title: "Simple wavelet sets in n dimensions" Rating PG
Abstract: A wavelet set in R^n can be defined analytically as a set whose characteristic function is the Fourier transform of a wavelet. Alternatively, it can be defined geometrically as a set that tiles n-dimensional space under both translation by the integer lattice and dilation by an expansive integer matrix. In both contexts, it is somewhat surprising that such sets exist. However, for some dilations, such sets can be found that are geometrically quite simple, consisting of a finite union of convex sets. This talk will describe the generalization to arbitrary dimensions of a two dimensional construction of simple wavelet sets presented in a Fearless Friday in late 2011. In keeping with the title, the proof itself has become simple by making use of a little known combinatorial result.
Rating: PG (some undergraduate mathematics or computer science understanding)
***
BLOCK 4, 2012 - December
Friday, December 7
Noon, TSC 122
Craig Guilbault (University of Wisconsin-Milwaulkee)
Proof or Swindle? The surprising effectiveness of sketchy technique
Rating: PG (some undergraduate mathematics or computer science understanding)
***
BLOCK 3, 2012 - October
Friday, November 16
2:30pm, TSC 122
Marc Chamberland (Grinnell College)
The Computer's Role in Mathematical Discovery and Proof
Rating: PG (some undergraduate mathematics or computer science understanding)
***
Friday, November 9
Noon, TSC 122
Stefan Erickson (CC)
Mathematics of Poker
BLOCK 2, 2012 - October
Friday, October 5
2:30 pm, TSC 229
Jane McDougall (CC)
Title: Harmonic Mappings and Minimal Surfaces (or what I would be doing if I were on sabbatical)
Abstract: We will examine briefly some of the highlights in the history of minimal surfaces, with a particular goal of setting in context the classical Scherk doubly-periodic surface and its generalization to the notion of a JS surface. We will then discuss harmonic maps (complex functions which are harmonic rather than analytic) and their connection with minimal surfaces. We explore the advantages of using hyperbolic geometry in constructing harmonic maps with specified properties. Time permitting, we will also consider the construction of harmonic maps onto hexagons that admit a JS surface. Technical aspects of this talk will also be communicated by an appeal to geometric intuition (i.e. there will be pictures).
Rating: PG13
***
Friday, October 12
NOON TSC 122
Kathy Merrill (CC)
Title: Sunrise, Sunset
Abstract: Although we expect the earliest sunset and latest sunrise to occur on the winter solstice, they do not. The mathematics behind this phenomenon is related to a curve called the analemma. This talk will explore the factors that complicate our view of the path of the sun, and develop a model that accounts for the difference between the solstice and and the extrema of sunrise and sunset times.
Rating: G/PG
***
Thursday October 18, 2012
6:30 pm, Packard Hall
Joel E. Cohen (The Rockefeller University and Columbia University, New York)
Sustainability, nations, globalization: can we have them all with 7 billion people and more?
Whatever sustainability means, it includes protecting Earth's physical, chemical, and biological environments locally and globally for time periods of human concern, subject to natural variability. National governments usually view their primary responsibilities as lying within their own boundaries on the time scales of the next election cycle or, at most, the education of the next generation. Global businesses look for resources and markets wherever they offer economic advantage to globally dispersed stockholders and management in time for the next quarterly report or the next annual meeting of stockholders, or (rarely) over the coming decade. Since 1820, aggregate economic activity grew more than 74-fold, and now the material inputs and outputs of economic activity have magnitudes comparable to what Earth can yield and absorb. Some scientists warn of possible abrupt changes in vital Earth systems. Half of today's 7 billion people remain poor. Billions more people and massive urbanization and ageing are in prospect in the coming decades. In this new world, it is time to create the information, incentives and institutions needed to reconcile environmental sustainability, national governance, and economic globalization.
Friday October 19, 2012
Noon
Joel E. Cohen, The Rockefeller University and Columbia University, New York
Title: Taylor's power law of population variation, stochastic models of population dynamics, and abrupt change
Abstract:
Vital Earth systems have changed abruptly in the past. Some scientists, based on models and data, warn of possible abrupt changes in prospect. My research on mathematical models of population dynamics led recently to an unexpected theoretical example of a singularity in the exponent of Taylor's power law of population variability (or fluctuation scaling) in response to smooth changes in the power spectrum of an environmental factor like temperature. Abrupt changes can arise even where least expected. Are we ready?
BLOCK 1, 2012 - September
Friday, September 7
2:30 pm, TSC 229
Fred Tinsley (CC)
Title: Noncompact Manifolds that are inward tame or "what I did on my sabbatical"
Abstract: This project has its roots in the summer geometric topology workshop
at Colorado College in 2000. By 2002 Craig Guilbault (UW-Milwaukee) and I had
formulated a '10-year plan' to completely understand the ends of open, inward-
tame manifolds in high dimensions. This effort has used extensively theories from
manifold homology and cohomology, combinatorial and geometric group theory,
algebraic K-theory, and manifold topology. I will describe the problem and report
on our progress. This talk should be accessible to any student who has completed
MA 375 Introduction to Mathematical Analysis. I even will pose a problem in
computer graphics for which a solution would be quite helpful.
Rated: R
Friday, September 14
1:00 pm, TSC 122 (Lecture Hall)
Matthew Whitehead (CC)
Title: The End of Privacy?
Abstract: Do you value your personal privacy? Are you concerned about the degradation of privacy in the Internet Age? Your answers may depend on your age and cultural background, but one thing is clear: technology and data mining are redefining our notions of privacy. In this talk we will discuss domestic surveillance using hummingbird-sized drones, how some of your anonymous data might not be quite so anonymous, and how people are tracked online and shown personalized advertisements chosen by machine learning systems.
Rating: G
Friday, September 21
2:30pm, TSC 229
Mike Siddoway (CC)
Title:"A Wind Erosion Equation"
Abstract: Wind erosion is still a major problem in the Great Plains. If you see dust rising off an acre of land, this translates to about a dime's width of soil being liberated from the surface, approximately five tons of particulate. Beginning in the late 1950's, scientists in Canada and the US began an effort to study the factors behind wind erosion. The idea being: "The better we understand the mechanisms of soil loss to wind, the more we can do to prevent catastrophic losses, like those suffered during the Dust Bowl years." The "equation" (really an algorithm) that was devised in the mid-60's, though improved upon in many ways since, is still the most widely used around the world. The modeling of "wind erosion," the problem that first brought mathematics deeply into the study of soil processes, is still one of the most actively pursued areas in all of soil science.
Rated: G
2011-2012
BLOCK 8, 2012 - April - May
Friday, April 27
2:30 pm, TSC 229
Steven Janke (CC)
Title: Two Statistical Paradigms: Bayesian versus Frequentist
Abstract: The vast majority of statistical analyses done these days is based on Frequentist methods. Certainly all undergraduate statistics courses feature this approach. Yet, the Bayesian method has been around for nearly as long and has many features to recommend it. This talk will give an overview of both methods using some specific estimation examples and then list some of the mathematical and philosophical points of contention between the two. Using some recent results, it becomes a little clearer how to proceed in refining statistical techniques.
Rated: PG-13
Friday, May 4
12:00 pm, TSC 122
Dr. Jugal Kalita (UCCS, Computer Science)
Title: Automated Data Extraction: Retrieving Temporal and Geospatial Information from Text
Abstract: This talk discusses a system that extracts and displays temporal and geospatial entities in text. The first task involves identification of all events in a document followed by identification of important events using a classifier. The second task involves identifying named entities associated with the document. In particular, we extract geospatial named entities. We disambiguate the set of geospatial named entities and geocode them to determine the correct coordinates for each place name, often called grounding. We resolve ambiguity based on sentence and article context. Finally, we present a user with the key events and their associated people, places, and organizations within a document in terms of a timeline and a map. For purposes of testing, we use historical Wikipedia articles, such as those describing wars, battles, and invasions. We focus on extracting major events from the articles, although our ideas and tools can be easily used with articles from other sources such as news articles.
Rating: PG
Friday, May 11
2:20 pm, TSC 229
Stefan Erickson (CC)
Title: Pairing-Based Cryptography
Abstract: In 1985, Victor and Miller and Neal Koblitz independently suggested that the discrete logarithm problem for elliptic curves could be used as the basis for cryptosystems. Elliptic curves offered faster computations and less bandwidth for the same level of security as traditional cryptosystems such as Diffie-Hellman and RSA. Over the past 27 years, elliptic curve cryptography has grown into one of the most interesting applications of theoretical number theory.
A recent development in elliptic curve cryptography is the use of bilinear pairings. At first, pairings were used as an attack on supersingular elliptic curves. Later, constructive applications came in the form of tripartite key exchange, identity-based encryption, and short signatures. In order for pairing to be used, however, efficient implementation and pairing-friendly curves had to be developed. This talk will provide an overview of many of these exciting ideas and applications.
Rated PG13
BLOCK 7, 2012 - March - April
Friday, March 30
2:30 pm, TSC 229
Robin Wilson (Open University, UK)
Title: 100 Years of Graph Theory
Abstract: The years 1890-1990 saw a complete change in the subject now known as graph theory -- from a collection of individual results to a more coherent theory. In this talk I survey the century decade by decade, outlining some of the mossignificant advances. No previous knowledge of graph theory is required.
Rating: PG-13
Friday, April 6
12:00 pm, TSC 122 (pizza will be served)
Robin Wilson (Open University, UK)
Title: Great Mathematicians
Abstract: Last September 'The Great Mathematicians' was published. Written by Raymond Flood and myself, it is a coffee-table book of 200 pages and 350 pictures which is very inexpensive and everyone should buy a copy! In this illustrated talk, I present ten of the featured mathematicians, ranging from Pythagoras and Fibonacci to Florence Nightingale and Hilbert.
Rating: G
BLOCK 6, 2012 - February - March
Friday, Feb. 24
2:30 pm, TSC 229
Dr. Loren Cobb, U.C. Denver
Title: Tracking Epidemics and Wildfires
Abstract: This will be an overview, with lots of computer animations, of the recent research of the Data Assimilation Group at the University of Colorado Denver. We apply our newly developed very-high-dimensional filters to incoming data in real time, to track and forecast emerging epidemics, wildfires, weather fronts, hurricanes, and anything else that has nonlinear spatial dynamics. Of special interest may be our simulation of the first wave of the Black Death in Europe, from 1347 to 1350.
Rated: PG-13
Wednesday, Feb. 29
12:00 pm, Olin 1 (note the special location, under the Olin "Fishbowl")
(pizza will be served)
Dr. Marlow Anderson, CC
Title: A Celebration of Leap Day
Abstract: Why do we have a leap day, anyway? In this talk I shall answer this question and more, exploring the history, customs and background for Leap Day. We'll have a look at the inadequacies of the Roman calendar, and see why and how Julius Caesar was motivated to introduce calendar reform. But the flaws in the Julian calendar became more and more troublesome as the centuries passed, especially for the Church fathers charged with computing the date of Easter, and eventually Pope Gregory XIII convened a commission in the 1580s to reform the calendar again. We'll examine some interested proposed alternatives, including one by Omar Khayyam. In the process we'll encounter some interesting astronomy and mathematics.
Rated: G
Friday, March 9
12:00 pm
TSC 122
Speaker: Andrea Bruder, Colorado College
Title: Period 3 Implies Chaos
Abstract: In 1964, Sharkovsky introduced a new ordering of the positive integers and proved that if a continuous function mapping an interval to itself has a point of period k, then it has a point of period r for all r following k in this ordering. His result unfortunately did not receive the attention it deserved. A decade later, Li and Yorke published a special case of Sharkovsky's theorem in their well-known paper "Period 3 implies chaos", which coined the word chaos in the mathematical sense. We will see how Elaydi proved a converse of Sharkosky's theorem recently by constructing piecewise continuous functions with certain periods, and we will show how continuously differentiable functions with the same properties may be constructed.
Rated: PG-13
BLOCK 5, 2012 - January - February
Friday, January. 27
2:30 pm, TSC 229
Marlow Anderson, Colorado College
TITLE: Cyclotomic Polynomials are Irreducible
The cyclotomic polynomial for a positive integer n is the monic polynomial whose roots are exactly the primitive n-th roots of unity. This polynomial is just
(x^n-1)/(x-1) if n is prime, but is a polynomial of order phi(n) in general (where phi is Euler's phi function). It is a common exercise in a beginning abstract
algebra course to prove that the cyclotomic polynomials for primes are irreducible - they can't be factored. This is also true for non-prime n. I recently
reviewed a paper for the Monthly which consisted of a compendium of many different historical proofs of these results. In this talk I'll give some general
background, and have a look at some of the most interesting and illuminating of these proofs, including proofs by such figures as Gauss, Eisenstein,
Dedekind and Landau. Along the way we'll have the chance to revisit such interesting topics as roots of unity, symmetric polynomials, and Gauss's Lemma.
We'll try to avoid the irresistible temptation to do some Galois theory!
Rated PG13.
Thursday, Feb. 2
12 pm, TSC 122 (pizza will be served)
Dr. Beth Malmskog, Wesleyan University
Title: Beyond Pig Latin: Private Communication over Pubic Channels
Abstract: Credit card numbers, legal documents, medical records, business correspondence, love letters: just a few examples of information that we want to share with certain parties but definitely do not want to share with others. Governments and corporations have similar needs for privacy in communication. How can information be shared privately when eavesdroppers overhear every word? In this talk, I will discuss a few of the methods that people have used to send secret information over public channels. We discuss a sampling of the history and mathematics of symmetric-key cryptography, Diffie-Hellman key exchange, and the big picture of public-key cryptography. If time permits, we conclude with a look at the future-how can we maintain privacy after quantum computers render our current techniques useless?
Rated: PG
Friday, Feb. 3
3pm, TSC 229
Dr. Beth Malmskog, Wesleyan University
Title: Automorphisms of a Family of Maximal Curves
Abstract: Over the real or complex numbers, a system of equations can have infinitely many solutions. That means that curves over these fields can have infinitely many points. If we instead look for solutions over a finite field, there can clearly only be finitely many. The Hasse-Weil bound gives an upper limit for how many points can lie on a curve over a finite field. Curves that actually attain this upper bound are called maximal. Maximal curves have some very interesting properties, and can be used to create good error-correcting codes. They often have rich structure, meaning many symmetries (automorphisms). Recently, Rachel Pries (CSU), Robert Guralnick (USC), and I found the full symmetry group for the curves in a new family of maximal curves. I will discuss the background and context of our result and give an outline of the proof.
Rated: R
Friday, Feb. 10
12 pm , OLIN 1 (lecture hall under the "Fishbowl")
Dr. Radu Cascaval, UCCS
Title: Control Mechanisms in Spatial Networks
Abstract: The dynamic control of spatial networks presents numerous challenging problems, both theoretically and computationally. I will describe a study of the cerebral autoregulation (CA) mechanism in the cardiovascular network, in which both the heart rate (HR) variability and the peripheral resistance (PR) variability act as controls for the pressure and flow rates throughout the system, with the aim of maintaining constant flow in the brain during dynamic changes. The dynamics of the network is characterized by distinct patterns of the nonlinear pressure and flow distribution, and related inverse problems will be presented. Furthermore, we will discuss evidence that the complexity of the underlying network and the presence of different scales in the model influences the effectiveness of these control mechanisms.
Rated: PG -13
BLOCK 4, 2011 - December
Dec. 2, 2:30 pm in TSC 229.
Speaker: Kathy Merrill
Title: Simple wavelet sets in R^2
Abstract: A wavelet set in R^2 can be defined analytically as a set whose characteristic function is the Fourier transform of a wavelet. Alternatively, it can be defined geometrically as a set that tiles the plane under both translation by the integer lattice and dilation by an expansive integer matrix. In both contexts, it is somewhat surprising that such sets exist. However, for some dilations, such sets can be found that are geometrically quite simple, consisting of a finite union of polygons. This talk will present new results about which matrix dilations have such simple wavelet sets.
Rated: PG-13 (linear algebra and some analysis required)
Monday, December 12 at NOON in TSC122
Pizza will be served
Speaker: Matthew Whitehead, Colorado College
Title: Evolving Video Game Bots
Abstract: Many modern video games have computer-controlled characters (bots) that are painstakingly crafted by hand to behave in believable ways. Unfortunately, this process is expensive, time-consuming, and often leads to bots that act in peculiar ways. In this talk we will look at the automatic creation of bot code using biological evolution for motivation. This process of growing code is called genetic programming and can be used to solve a variety of problems without a human programmer manually writing the source code.
Rating: PG
Tuesday, December 13 at 2:30 pm in TSC229
Speaker: Matthew Whitehead, Colorado College
Title: The Provenance of CBR Cases
Abstract: Case-based reasoning systems routinely record the results of prior problem-solving, but not the provenance of new cases: the way in which the new cases were derived. In this talk we will investigate the value added by tracking case provenance information in CBR systems, especially when timely feedback may not be available. In particular, we will examine the effect of using provenance to propagate case maintenance in order to target maintenance effort. We will look at results of such a system on two well-known machine learning datasets.
Rating: PG-13
Thursday December 15 at Noon in TSC122
Pizza will be served
Speaker: Michael Hay, Cornell University
Title: Public Use of Private Data
Abstract: This talk explores the following question: How can an organization --
such as a hospital -- release sensitive data about individuals -- such as
patient medical records -- to the public without violating the privacy of
those individuals? In today's digital age, this problem is much harder
than you might expect. I will show some of the pitfalls of simple
solutions that rely on anonymity and then look at how randomization
(i.e., coin flips) can play a role in protecting privacy.
Rating: G/PG (basic probability and statistics are used, but most of the
talk is accessible without this background)
Friday, December 16 at 2:30 pm in TSC229
Speaker: Michael Hay, Cornell University
Title: Analyzing Private Network Data
Abstract: Many of our social interactions -- email, phone, online networking --
leave behind a digital trace. These traces are a rich source of data for
social science and present an opportunity to fundamentally advance our
understanding of many facets of society. However, these traces are also a
liability. The collected data can be highly sensitive, revealing intimate
details of personal relationships. Concerns about privacy make data
owners reluctant to share the data and threaten to disrupt a vast array
of new digital services, social science, and economic opportunities.
In this talk, I will describe two different approaches for addressing the
problem of enabling the accurate analysis of private network data. In
the first approach, the network data is "anonymized" prior to being
released to the public. While this is a natural approach, I will provide
evidence that the privacy protection is much weaker than one might
expect. In the second approach, I will describe algorithms for releasing
randomly perturbed statistics about the network topology. With this
approach, it is possible to release accurate approximations of key
network statistics while simultaneously guaranteeing rigorous privacy
protection.
Rating: PG-13
Monday December 19, 2011
Speaker: Ben Widermann, Harvey Mudd College
Time: 12 Noon
Title: Keeping Secrets: Language-based Secure Information Flow
Rated PG for mild programming (method calls and if statements) and for propositional logic.
Abstract:
We all have secrets. Sometimes we entrust those secrets to software. And sometimes software blabs. Maybe your phone posts your whereabouts to Facebook, or your favorite website exposes your credit card. Is there any way to guarantee that our software won't intentionally or accidentally leak our secrets? In this talk, we explore this problem by developing a small programming language with an interesting property: every program in this language is guaranteed not to leak. Along the way, we'll run up against some of the fundamental limits of computation.
Note: this talk will be interactive -- please come prepared to do a bit of problem-solving.
Tuesday December 20, 2011
Speaker: Ben Widermann, Harvey Mudd College
Time: 12:30pm
Title: Modular and Efficient Database Access
Rated R for moderate programming (Java + SQL; virtual method dispatch) and for some graduate-level computer science (abstract-interpretation-based program analysis).
Abstract:
All our major financial, health, and government enterprises rely on software systems that manipulate large datasets. These "enterprise applications" are notoriously difficult to design, debug, and maintain. Because enterprise applications specify business logic rules over large datasets, they often combine a general-purpose programming language with a database. The clean, effective integration of these two semantic domains has eluded researchers for over 30 years. As a result, today's programmers are forced to throw out their best programming practices for the sake of program efficiency.
This talk presents a different approach, namely that program analysis and programming language design can help programmers write enterprise applications that are both well-designed and efficient. The premise of our approach is that programmers perform premature optimization when they write SQL queries. Instead, programmers should describe computations over database data in the same way they describe computations over in-memory data. The compiler should differentiate the computations and automatically prefetch database values on behalf of the programmer. We describe two techniques that accomplish this goal: one technique based purely on static program analysis and another technique that combines static program analysis with elegant language design.
BLOCK 3, 2011 - November
Nov. 4, 2:30 pm in TSC229
Speaker: Dr. Zak Mesyan, UCCS
Title: Groups where free subgroups are abundant
SLIDES FROM TALK (PDF)
Abstract: About 20 years ago it was shown that in the group of all
permutations of the natural numbers "almost all" (in some topological
sense) finitely generated subgroups are free. Since then a number of
other groups with this property have been discovered. I will discuss
these examples and the recent efforts to study this phenomenon
systematically.
Rating: R
Nov. 11, 12:00 pm in TSC122 (pizza is served)
Speaker: Dr. Beth Schaubroeck, USAFA
Title: Julia Sets and Surfaces
Abstract: In this talk we will discuss Julia Sets and surfaces related to them (joint work done with Julie Barnes, Clinton Curry, and Elizabeth Russell). I will also talk about how mathematics research can advance in surprising ways. Although the topic uses complex variables, all definitions and terms will be described at a very introductory level, and the only prerequisite for understanding the concepts involved will be calculus.
Rating: PG
Thursday, Nov. 17, 12:00 pm in TSC 229
Speaker: Matthew Whitehead
Title: Information Filtering using Machine Learning
Abstract: Internet searches often return millions of pages of results, many of which are marginally related (or completely unrelated) to the user's actual target. Google News lists dozens of news stories on its front page and each story often has thousands of related articles. Sometimes it can be quite difficult to process and filter through all this data to get to the information that you really want. In this talk we will discuss using various machine learning methods to help us deal with information overload. In particular we will look at the problems of dealing with ambiguous search queries and summarizing news articles.
Rated: PG-13
Nov. 18, Noon, in TSC122
Speaker: Dr. James Powell, Utah State
Title: The Timing of Insect Development and Trajectory of Bark Beetle Outbreaks
Abstract: Temperatures directly but nonlinearly influence the rates at which insects complete development in their various life stages and therefore the timing (phenology) of their emergence. The mountain pine beetle (Dendroctonus ponderosae Hopkins), is an aggressive insect which attacks living pine host trees (genus Pinus). These trees have significant defensive mechanisms, requiring the beetles to attack en masse to successfully colonize. Since the beetle larvae consume the phloem underneath the bark they exhaust their host, requiring dispersal to new hosts every year. Adult beetles must colonize in a relatively narrow window of time to take advantage of warm temperatures for oviposition but also allowing cold-hardened larvae to emerge before winter freeze. Thus the beetles exist in a precarious niche depending on carefully synchronized timing. Changing temperatures in Western North America have broadened that niche across vastly larger regions, leading to mountain pine beetle outbreaks across the West. Impacts due to bark beetles are currently larger than those due to fire in these ecosystems. This talk outlines the development and application of mathematical models for bark beetle phenology and how the dynamics of phenology influences the course and severity of outbreaks.
Rating: PG
BLOCK 2, 2011 - October
Friday Oct. 7, 2:30 pm,
TSC 229
Speaker: David Brown, Colorado College
Title: Quorum sensing in bacteria: Simple models and general results
Abstract: Many bacteria have the ability to detect and respond to their local population density, a phenomenon known as quorum sensing. Over the past decade, a number of mathematical models have explored quorum sensing in several species. While they have shared some core predictions, the models have generally been system-specific, and have used a variety of incompatible assumptions. I will talk about my recent attempt to bring some coherence to the subject by carefully formulating and analyzing simple, generic models of quorum sensing. I will highlight the need to derive differential equations from first principles, show how the models predict key similarities and differences between different kinds of bacteria, and explore the role that spatial structure may play. My methods include qualitative analysis and numerical solutions of ODEs and PDEs.
Rating: PG-13
Friday, October 14, Noon
Kresge Lecture Hall (Tutt Science Center 122)
Speaker: Barry Balof, Whitman College
(Barry is a CC alum, and his talk kicks of our celebration of Homecoming Weekend.)
Title: "Fibonacci and Friends: Famous Number Sequences and the Liberal Arts"
Abstract: One of the most ubiquitous constructions to come out of mathematics is
the Fibonacci Sequence, which has applications to seemingly myriad
different fields, such as Biology, Art, Music, and even Contract
Bridge. But does the Fibonacci Sequence and its associated Golden
Ratio tell the whole story? Are there other, less heralded sequences
and constants that arise in other fields? In this talk we will look
at the Padovan Sequence, and its applications to architecture, as well
as Coordination Sequences and their application to geology.
Rating: G
Friday Oct. 21, 2:30 pm,
TSC 122 Lecture Hall
Speakers will be Elise Hellwig and Hannah Thompson, CC students who will speak about research in mathematical biology that they did last summer. Each talk will last 30 minutes, be rated PG-13, and involve population dynamics.
Speaker 1 : Hannah Thompson
Title: Foraging Behavior and Pattern Formation in a Predator-Prey Model
Abstract: I will be presenting a model of ladybugs (Coccinella septempunctata, Hippodamia convergens) and their aphid prey (Aphis helianti), which are patchily distributed on Yucca glauca near Colorado Springs. Fieldwork has shown that predation by ladybugs causes high variation in aphid population levels over short spatial scales (several meters). Here, we use bifurcation analysis of a two-patch ordinary differential equation model to investigate how ladybug foraging behavior leads to this heterogeneity. We have found that diffusion and density independent immigration of ladybugs promote heterogeneity between the patches in our model.
Speaker 2: Elise Hellwig
Title: Metapopulation Modeling and Analysis with Demographic Stochasticity
Abstract: As human expansion claims more land, previously connected habitats become fragmented, converting larger populations into smaller subpopulations connected through migration. Demographic stochasticity has been shown to have a significant effect in small populations. We explore how taking demographic stochasticity into account affects metapopulations which are typically made up of small subpopulations. We develop a density dependent model using Markov matrices to simulate a metapopulation and compare the predictions with those of a deterministic model. We analyze the asymptotic population size predicted by the deterministic model, and the mean time to extinction and the quasi stationary distributions predicted by the Markov model. We show that there is a significant difference between the two models under certain conditions and that the deterministic model often overestimates metapopulation persistence.
BLOCK 1, 2011 - September
Friday, Sept. 9; 2:30 pm
Kresge Lecture Hall (Tutt Science Center 122)
Speaker: Molly Maxwell, Colorado College
Title: Combinatorial Identities: Binomial Coefficients, Pascal's Triangle, and Stars & Bars
SLIDES FROM TALK (PDF)
Abstract: Binomial coefficients form the entries in Pascal's Triangle and appear frequently in combinatorial identities. In this talk, we'll look at several identities that appear in Pascal's Triangle and provide combinatorial proofs for some of these. Binomial coefficients also appear in a famous identity for the compositions of positive integers. We'll use a combinatorial "stars & bars" argument to prove this identity.
Rating: G
Friday, Sept. 16, 12:00 pm (pizza served)
Kresge Lecture Hall (Tutt Science Center 122)
Speaker: Mike Siddoway, Colorado College
Title: The Field of Origami Numbers
Abstract: In 8th Block of 2010 I gave a talk which included discussion of origami constructions and Groebner Basis techniques for determining whether certain figures could be "paper-folded" and if so, what sequence of moves could produce the desired object. In the questions following the talk, Marlow asked where the "Field of Origami Numbers" fit in the chain of fields beginning with the rational numbers. We'll answer this question in the "one-fold-at-a-time setting" and see where the field of paper-folded numbers sits in relation to the classical field of ruler and compass constructed numbers, and to fields arrived at using more exotic tools.
Rating: G
Friday, Sept. 23, 2:30 pm
Tutt Science Center 229
Speaker: Greg Oman, UCCS
TITLE: An independent axiom system for the real numbers
SLIDES FROM TALK (PDF)
ABSTRACT: Since the birth of formal mathematical logic, mathematicians have considered it aesthetically pleasing to axiomatize theories (which are axiomatizable) irredundantly; that is, in such a way that no axiom may be proved from the remaining axioms. In this case, such an axiom system is said to be independent. A famous early example of an independent axiom system is Hilbert's (original) axioms for Euclidean geometry. Since then, independent axiom systems have been found for various mathematical structures, including fields and vector spaces. To illustrate, it is a fairly well-known exercise in basic algebra to prove that the commutative axiom for addition is redundant in the set of axioms for a ring with identity. In this talk, we present an independent axiomatization of the complete ordered field of real numbers. In the presence of the completeness axiom, we will see that a surprising number of axioms can be 'thrown away' (i.e. can be proved from the remaining axioms). This talk should be accessible to all mathematics faculty as well as advanced undergraduates with a background in real analysis.
Rating: PG-13
Block 8 2011
Friday of Week 1 ( April 29 , 2011 ) at 2:30 PM in Tutt Science 229
Harmonic maps and Scherk surfaces Part II. by Jane McDougall Rating PG13
ABSTRACT: This talk is a continuation (with some review) of the February talk with the same title. In this talk I will look into the geometry that underlies the relationships among domain and range of harmonic maps of a specific type, and the (second complex) dilatation as a "measure of non-conformality". Hyperbolic geometry will be particularly relevant.
Audience: Some understanding of hyperbolic geometry helpful but not necessary. Some understanding of complex variables helpful but not necessary.
February 25th abstract: Minimal surfaces are embedded surfaces in three dimensional space with the property that they are locally area-minimizing. Scherk's doubly periodic surface was developed in 1831 by Heinrich Scherk (along with the singly periodic Scherk surface). Under appropriate parametrization, any minimal surface gives rise to a harmonic map, which is defined as a complex valued function that is harmonic and one-to-one on a complex domain. Using complex variables we can discuss the situations under which a harmonic map "lifts" to a minimal surface. We will focus on minimal surfaces related to Scherk's doubly periodic surface, and also consider the related harmonic maps as interesting functions in their own right.
Friday of Week 2 ( May 6, 2011 ) at noon in Tutt Science 122 (Kresge Lecture Hall)
The Kalman Filter: an algorithm for dealing with uncertainty. by Steven Janke Rating PG
ABSTRACT: Virtually all processes and measurements in the world are victims of error, often called noise. A robot never goes exactly as far as it should and never turns to exactly the direction programmed. With some probability and a classic algorithm, the Kalman Filter, we can at least manage all this uncertainty. This talk introduces the ideas from probability necessary to understand how error propagates and how even noisy measurements can help control the situation.
Friday of Week 3 ( May 13, 2011 ) at 2:30 PM in Tutt Science 229
Apollonian Circle Packings. by Stefan Erickson Rating PG
Apollonius's Theorem states that given three mutually tangent circles, there are exactly two circles which are tangent to all three. Apollonian circle packings are produced by repeating the construction of mutually tangent circles to fill all remaining spaces. A remarkable consequence of Descartes' Theorem is if the initial four tangent circles have integral curvatures, then all of the circles in an Apollonian circle packing will have integral curvatures. This process results a sequence of integers with fascinating arithmetic properties.
In this talk, we will investigate the arithmetic properties of Apollonian circle packings. We will describe the Apollonian group action on the set of Descartes quadruples. We will talk about modular restrictions and density conjectures and theorems. Finally, we will show a correspondence between the root quadruples and reduced binary quadratic forms and answer an open question about finding the root quadruple of a given Descartes quadruple.
Block 7 2011
Friday of Week 1 ( April 1, 2011 ) at 2:30 PM in Tutt Science 229
CM elliptic curves, applications, and generalizations, by Sunil Chetty: Rating PG-13 to R
Friday of Week 2 ( April 8, 2011 ) at noon in Tutt Science 122 (Kresge Lecture Hall)
Design and Construction of Internet Search Engines by Matthew Whitehead: Rating PG
ABSTRACT: We all use search engines to find relevant information online. For almost any search query we nearly instantly receive page after page of interesting results. In this talk we will discuss the theory of search engine design as well as some of the details of implementation. We will also consider some of the problems search engines will have to deal with in the future to maintain good performance.
Block 6 2011
Friday of Week 1 ( February 25, 2011 ) at 2:30 PM in Tutt Science 229
Friday of Week 2 ( March 4, 2011 ) at noon in Tutt Science 122 (Kresge Lecture Hall)
NOVA Series on Artificial Intelligence, hosted by Matthew Whitehead Rating G/PG
ABSTRACT: Matthew is teaching Artificial Intelligence this block. This particular NOVA is appropriate to his class. Although it is not a talk, you may still summarize it and turn in your summary as one the four required for graduation. Try to sit near the front. Matthew is unsure of the film quality :) .
Friday of Week 3 ( March 11, 2011 ) at 2:30 PM in Tutt Science 229
Why is Everyone Interested in Quantum Graphs? by Bob Carlson Rating: PG-13
ABSTRACT: There has been a recent explosion of research activity at the
intersection of graph theory, linear algebra, differential equations,
functional analysis, and applications. We will initially consider some of the
motivating applications and a bit of history. The interaction of
linear algebra and graph theory will be reviewed. Differential
equations will then make their appearance. Having set up the mathematical
models, some recent results and open problems will be discussed.
Block 5 2010
MONDAY, January 31, 2011, at Noon, in Tutt Science 122 (Lecture Hall)
Bioeconomics and optimal control of the spread of invasive species, by Dr. Alan Hastings, U.C. Davis
Rated PG
Abstract: This talk will focus on the development of approaches for designing optimal controls for invasive species, with an emphasis on the spread stage (after establishment). The importance of the details of the model will play a role. Examples will be drawn from Spartina alterniflora and other species.
FRIDAY, February 11, 2011, at 2:30 PM in Tutt Science 229
High-dimensional knots by Fred Tinsley
Rating: PG-13
ABSTRACT: In Fred's recent work on the ends of high-dimensional surfaces, issues of high-dimensional knotted surfaces have arisen. Fred will discuss the similarities and differences between these knots and those in three dimensions that we know and love He also will show how these knots arise naturally in understanding ends of high-dimensional surfaces.
Block 4 2010
Friday, December 3, 2010, at 2:30 PM in Tutt Science 229
Primary Decomposition of Conditional Independence Ideals by Amelia Taylor Rating: R
ABSTRACT: A conditional independence ideal is an ideal generated by binomials which describe the conditional independence relations of a statistical model. The primary decomposition of an ideal is a decomposition that is for ideals what a prime factorization is for integers. The primary decomposition of a conditional independence ideal is interesting as it can give an alternate description of the independence relations for the model or illustrate the failure of the intersection axiom, among other applications and interpretations. I will define conditional independence ideals and then describe what is currently known about their primary decompositions including a new result that is joint with Irena Swanson.
Friday, December 10, 2010 at NOON in Tutt Science 122 (Kresge Lecture Hall)
Making Movie Recommendations Using the Netflix Dataset by Matthew Whitehead Rating: PG
ABSTRACT: Have you ever wondered how Netflix recommends new movies to you based on previous movies that you've seen and rated? These recommendations are made by taking into account movie ratings by other Netflix customers. This process is called collaborative filtering. In this talk, we will discuss several collaborative filtering algorithms and see how they perform on the publicly available Netflix ratings dataset. We will also discuss the results of Netflix's recent $1,000,000 collaborative filtering challenge.
Friday, December 17, 2010 at 2:30 PM in tutt Science 229
Irreducible Cubics Modulo Five: by Marlow Anderson Rating: PG-13
ABSTRACT: When you consider the integers modulo 5, you get a field F_5 with five elements. There are exactly 600 (=4.5.5.5) cubic polynomials with coefficients from this field. It turns out that exactly 160 of these polynomials cannot be factored - they are irreducible. Recently, Hendrik Lenstra has published a short article in the American Mathematical Monthly which gives a cute proof of this fact. In this talk, we will look at several ways to perform this count. In the process, we will give a gentle and student-friendly introduction to the construction of finite algebraic extensions of F_5. We will then encounter a lot of the fundamental theorems about finite fields.
Block 3 2010
Thursday November 4, 2010, 3-4pm
Yulan Qing (CC Alumna)
A talk for students, including those interested in learning more about graduate school experiences.
Title: The Geometry of Groups
Abstract: You know what a group is, but do you know what a group looks
like from far away? We will see a few examples of how groups, graphs, and other spaces can be connected, and literally "draw" a few conclusions about the group of interest.
Friday, November 5 at 2:30 pm
From Bagels to Boundaries of Groups by Yulan Qing (CC Alumna)
Rating: PG
Abstract: Take three bagels and splice them together to form a pretzel. A tiny ant walking along this pretzel explores the space and gets the idea that the space is flat, with vertical walls that it can climb along, and that the space is infinite. We give the ant the concept of a visual boundary, and show that if we change the geometry of the bagel, the topology of the boundary also changes. This is a particular example of a right-angled Coxeter group. It is an open question as to whether right-angled Coxeter groups have unique CAT(0) boundary. This example could potentially give a negative answer to that question.
Friday, November 19 at 2:30 pm, TSC 229
Congruent Numbers and Elliptical Curves by Sunil Chetty
Rating: PG-13
Block 2 2010
Friday, October 8 , 2010 at noon in Tutt Science 122 (Kresge Lecture Hall)
Chaos and the Mandelbrot set by Andrea Bruder
Rating: PG
ABSTRACT: The Mandelbrot set is certainly the most popular fractal and has even been called the most popular object of contemporary mathematics, the most beautiful and the most complex object which has ever been made visible. Benoit Mandelbrot conducted his experiment in 1979 and, realizing that he had discovered a mathematical gem, he pushed further to revitalize an area of mathematics that had been dormant for over 60 years.
Friday, October 15 , 2010 at noon in Tutt Science 122 (Kresge Lecture Hall)
Introduction to Web Development by Benjamin Thomas
Rating: PG
ABSTRACT: Ever wanted to know what happens when you load up facebook.com in your browser? Ever wanted to know what it would take to write the next Twitter? Then this talk is for you! This will give you a brief (mostly) non-technical introduction to the technologies involved in writing web sites. We'll talk about HTML, CSS, Javascript, PHP, Django and Ruby on Rails. There will be code shown, but it should be pretty straightforward.
Friday, October 22, 2010 at 2:30 PM in Tutt Science 229
The Graph Menagerie: Abstract Algebra meets the Mad Veterinarian by Gene Abrams (UCCS)
Rating: PG-13 (with 5 minutes of X at the end)
ABSTRACT: There are recreational math activities known as "Mad Vet Puzzles". I was first exposed to these during a workshop on Math Teacher Circles (at the American Institute of Mathematics in Palo Alto CA, June 2008), where these were used as the basis of group problem solving activities for middle school math teachers and students.
(See, for example. http://www.bumblebeagle.org/madvet/index.html )
After playing with these puzzles for an hour or so, something about them struck me as being eerily familiar. On reflection, I realized that they are intimately and highly non-trivially related to the research work I have been doing recently on ring-theoretic structures called Leavitt path algebras. (Go figure!) In this talk I'll describe Mad Vet puzzles, and how they naturally lead to interesting questions about semigroups, groups, and graphs. The talk will definitely be more-than-accessible to anyone who has seen the definition of a group, and basic concepts about such structures.
Block 1 2010
Friday, September 10, 2010 at 2:30 PM in Tutt Science 229
Modeling Dependent Random Variables using Copulas by Steven Janke
Rating: PG13
ABSTRACT: First courses in probability concentrate on independent random variables with an occasional nod to arbitrary joint distributions. But much of probability is involved with various forms of dependency. Yet, the basic notion of dependence between several random variables is not easy to characterize. Correlation coefficients give a summary view, but not a detailed one. Copulas, introduced somewhat under the radar about 40 years ago offer a more systematic approach to modeling dependency. This talk will define copulas, explore some of their uses, and finally address a paper using copulas that Wired magazine claimed is to blame for the financial meltdown in the last few years.
Friday September 17, 2010 at noon in Tutt Science 122 (Kresge Lecture Hall)
A Problem from the game SET^{TM} by Amelia Taylor
Rating: PG
ABSTRACT: The game SET^{tm} requires players to look at a collection of cards and select three cards which form a set, according to particular rules. The cards have different shapes, colors, shades, and numbers of objects on them and I call these categories parameters. A set is formed when a player finds three cards such that for each parameter the cards are either all equal or all distinct. Many combinatorial questions arise naturally from this game. One in particular has generated a wealth of interest in the mathematical community, including a new result from 2009, but not necessarily stated as a combinatorial question. Amelia will explain this problem, its interpretation in enumerative geometry and in algebra (as well as any algebra or geometry needed for this explanation). She will also talk about approaches to solving the problem in low dimensions and at mention a few other fun mathematical questions related to the game.
Friday September 24, 2010 at 2:30 PM in Tutt Science 229
Manifold learning: by Fred Tinsley
Rating: PG-13
ABSTRACT: A common phenomenon in multivariate statistical analyses is the following: as the complexity of the statistical technique employed increases, the concern with the validity of that technique decreases. A particularly common sin is the tacit assumption of linear relationships among the various variables. Fred will introduce the general idea of dimension reduction in which one determines whether data measures on k variables are secretly of dimension less than k. One very commonly used method is principal components analysis (PCA) where algebraic techniques identify linearsubspaces that for all practical purposes contain the data. Even in the absence of a useful linear reduction, a non-linear one may be possible. Fred will discuss Manifold learning, a collection of techniques for smooth reduction to subspaces that are not necessarily linear. These subsume PCA and most other linear reduction techniques.
BLOCK 8, 2010
Friday April 23rd at 2:30: Courtney Gibbons of the University of Nebraska, Lincoln
Title: The Determinant Trick. Rating PG13
Abstract: Somewhere, buried in your mathematical tool box, you have a mathematical Swiss army knife. Somewhere in that mathematical Swiss army knife, you have the determinant trick. Courtney will (re)introduce you to this handy tool and show you some cute commutative algebra applications. You don't need to have seen much past linear algebra to follow this talk, but having played with modules a little bit would be ideal.
Friday April 30th at NOON with Pizza: Mike Siddoway, Kresge Lecture Hall.
Title: "The 0th Watkins Lecture" (The Zeroth Watkins Lecture). Rating PG13
Abstract: Prof. John Watkins is retiring from our department this spring. Looking back over his widely-varying (and ongoing!) mathematical career I thought it natural to give a talk that calls on seemingly different but amazingly connected areas of mathematics, particularly from general fields that John contributed to. I'll start with some algebra (the computational variety which makes extensive use of Groebner bases) and move on to applications in graph theory, automatic theorem proving, and if time permits some origami theorem proving.
Friday May 7th at 2:30: Steven Janke
Title: Modeling Dependent Random Variables using Copulas. Rating: PG13 - NC17 (!)
First courses in probability concentrate on independent random variables with an occasional nod to arbitrary joint distributions. But much of probability is involved with various forms of dependency; the study of Markov processes is a good example. However, the simplest notion of dependence between several random variables is not easy to characterize. Correlation coefficients give a summary view, but not a detailed one. Copulas, introduced somewhat under the radar about 40 years ago offer an approach to modeling dependency. This talk will define copulas, explore some of their uses, and finally address a paper using copulas that Wired magazine claimed is to blame for the financial meltdown in the last few years.
BLOCK 7, 2010
Friday March 26th at NOON in TSC 122 (Kresge Lecture Hall) with Pizza:
Title: How to draw a Tree (with a computer). By Steven Janke.
Rating PG
It is often difficult to draw organic forms on a computer, but early work in the theory of computation has led to systems (L-systems) for finding algorithms that produce pretty good trees. In fact the systems lead to an interesting model for tree growth and perhaps can answer questions concerning the shapes of trees. This talk will introduce L-systems, explain how they lead to algorithms for drawing trees, discuss some of the math involved, and show how to actually draw some nice trees on the computer screen.
Wednesday March 31st 3:15-4pm and Thursday April 1st, 1:30 pm - ~4pm, in TSC 122 (Kresge lecture hall)
Capstone Talks
WEDNESDAY
3:15-3:35 Sarah Fletcher, "Theory and Practice of Bootstrapping with Applications in R"
3:40-4:00 Leon Li, "Ranks of Elliptic Curves over Number Fields"
THURSDAY
1:30-1:50 Marina Gresham, "(Hyper)Graphs of Zeta Functions and How They Related to Primes"
1:55-2:15 Sam McDowell, "Computer Vision and Robotic Guidance"
2:15-2:30 Intermission
2:30-2:50 Pat Ramsey, "A Design and Implementation of Distributed Generic Matching Framework"
2:55-3:15 Ben Rogers, "Using Leslie Matrices to Study Flammulated Owl Demographics"
Friday April 2nd at NOON in TSC 122 (Kresge Lecture Hall) with Pizza:
Title: Old Mathematics in the Modern World. By Michael Dorff of Brigham Young University.
Rating PG
Have you ever asked yourself "When will I going to use math?" If you search this question on Google, you learn that when "ordering lunch at the local fast food chain [you can] use math to count change." Hopefully, you don't need to study calculus to count change. So, there must be better uses for math. That's the topic of this presentation. We will discuss this question in several ways including movie clips that show how Hollywood sees math saving the world and serious news blurbs declaring the job opportunities for students who know mathematics. We will then discuss two or three topics that employ beautiful mathematics but are also used in many people's daily lives.
Tuesday April 6th at NOON in TSC 122 (Kresge Lecture Hall) with Pizza:
Title: Hedge Fund Manager Speaks
Friday April 9th at 2:30 pm in TSC 229
Title: Infinite Galois Theory. By Stefan Erickson.
For centuries, people have been interested in finding roots of polynomials. The quadratic formula, which has been known for millennia, explicitly finds the roots in terms of the coefficients of a degree 2 polynomial. Renaissance mathematicians produced formulas for the cubic and quartic equations, while the quintic equation eluded solutions until the early 1800s when Abel proved the unsolvability of the quintic. The question remained as to when a polynomial could be solved in terms of radicals. The answer was first found by a 20 year old French mathematician named Evariste Galois. His discovery of the connection between solving polynomials in radicals and symmetry groups is one of the most fundamental and beautiful ideas in all of modern mathematics.
In this talk, we will investigate the various amazing aspects of Galois theory. After giving several examples of finite extensions, we explore what Galois theory for infinite extensions might look like. This talk should be accessible to all math majors, although some background in abstract algebra is recommended.
BLOCK 6, 2010
Friday February 26th at NOON, in TSC 122, with Pizza: Matthew Whitehead of Indiana University Bloomington.
Title: Mining User Opinions on the Web.
Rating G
The Web is full of user opinions about books, CDs, movies, restaurants, political ideas, and just about everything else imaginable. Opinions can be found on blogs, message boards, social networking sites like Facebook and Twitter, and personal webpages. Powerful machine learning models that can automatically classify these opinions can be used to aggregate this information so that it is accessible and understandable by humans. For example, a search engine can rank results from a local search for the query "restaurants" by the percentage of positive opinions posted about each nearby restaurant. In this talk we will discuss the details of mining online opinions and show how performance can be improved with the use of ensemble machine learning algorithms.
Friday February 26th at 2pm, in TSC 229: Matthew Whitehead of Indiana University Bloomington.
Title: Building Accurate and Efficient Machine Learning Ensembles.
Friday March 5th at NOON, in TSC 122, with Pizza: Gary Grunwald of UC Denver.
Title: Statistical models for heart surgery risk.
Rating PG
Heart surgery can be a risky business, better only than the alternative. Deciding which patients are highest risk, and during what periods, is a job for smart statistical models that can use data on dozens of variables and tens of thousands of surgeries and can handle complications in the data including non-normal outcomes, non-linear functional forms, differential follow-up, and risks that change over time. Dr. Grunwald will talk about some of the statistical models he has used, the mathematics behind them, and what this tells us about heart surgery. The talk will include a lot of graphs, and not to assume any statistical background. Dr. Grunwald will also discuss some other projects in Biostatistics at UC Denver, and careers in biostatistics in general.
BLOCK 5, 2010
Friday January 29th at Noon, in Tutt 122: Jennifer Young, PhD Candidate, University of North Carolina-Chapel Hill
Math Department Non-Tenure Track Candidate
Title: The Fish Tank Problem
An aquarium holds 6 gallons of water. Each gallon contains 0.01 gallons of salt. Fish can survive in the tank as long as the total salt level stays below 0.09 gallons. Each week one gallon of water evaporates from the tank (the salt stays), and a new gallon is added.
How many weeks will it be until the fish can no longer survive in the tank? Not very long! So the real question becomes how much water do we need to remove and then add to the tank each week to keep the fish alive? To answer this question, I will present a brief introduction on discrete dynamical models (basically models that describe how a quantity changes over time). Then we will construct a model to figure out how to keep the fish happy!
Tuesday February 2nd at Noon, in Tutt 122: Kevin Woods, University of Michigan
Math Department Non-Tenure Track Candidate
Title: Cubing the Pyramid: or, Why We Need Calculus (and Measure Theory!)
Once you agree that a 1 inch x 1 inch square should have area 1 (isn't that the very definition of one square inch?), you can find the area of any polygon by cutting and rearranging the pieces. Can we do the same in three dimensions? Can you chop up a regular tetrahedron and rearrange the pieces into a cube? This was Hilbert's third problem, solved by Max Dehn, and the answer turns out to be "no". To find the volume of a regular tetrahedron, we (sadly? intriguingly?) need calculus.
But all of this analysis is based on an assumption: if you cut up an object and rearrange the pieces, the new object will have the same volume as the original. We will next challenge this assumption.
Weirdness ensues! A subject called measure theory comes to the rescue.
Talk with Pizza: Tutt Science Center 122
Thursday February 4th at 2:30 pm: Andrea Bruder of Colorado College. Tutt 229.
Title: Applied Left-Definite Theory: the Jacobi Polynomials, their Sobolev Orthogonality, and Self-Adjoint Operators. Rating PG13.
Abstract: It is well known that, for appropriate values of alpha and beta, the Jacobi polynomials are orthogonal on R with respect to a measure mu. However, for negative integer parameters alpha and beta, an application of Favard's theorem shows that the Jacobi polynomials cannot be orthogonal on the real line with respect to any positive or signed measure. But it is known that they are orthogonal with respect to a Sobolev inner product. Here, we will consider the special case where alpha=beta=-1. We shall discuss the Sobolev orthogonality of the Jacobi polynomials and construct a self-adjoint operator in a certain Hilbert-Sobolev space having the entire sequence of Jacobi polynomials as eigenfunctions. The key to this construction is the left-definite theory associated with the Jacobi differential equation, and the left-definite spaces and operators will be constructed explicitly. The results can be generalized to the case where alpha > -1 and beta=-1.
Friday February 5th at NOON with Pizza: Andrea Bruder of Colorado College. Tutt Science 122
Title: Soap bubbles and mylar balloons: the calculus of variations. Rating G
Abstract: Soap bubbles and soap films have been studied mathematically for about 100 years, since the Belgian physicist Joseph Plateau showed that solutions to shortest path problems could be generated by dipping a wire framework into a soap solution. Most recently, the behavior of soap and water films has been studied on board the International Space Station in a zero gravity environment, with surprising results! We will explore the mathematics of soap films, in particular the calculus of variations, to understand the shape of soap films and mylar balloons.
BLOCK 4, 2009
Friday Dec 4th at 2:30 pm: Fred Tinsley of Colorado College. Tutt 229.
Title: Algebraic Topology in a Non-compact Setting. Rating R
Abstract: In a world where pretty much everything of interest is compact, why would one bother studying non-compact objects? Unfortunately, analysis of compact (finite) complexes naturally requires understanding non-compact (infinite) ones, and generalizing the necessary techniques to this more complicated setting often runs into significant obstacles. Fred will illustrate some of these difficulties and discuss how they are affecting his current research.
Friday Dec 11th at NOON with Pizza: Jonathan Bredin of Colorado College. Tutt 122 Lecture Hall
Title: Introduction to Digital Audio. Rating G
Abstract: Do you want to learn about how your iPod and CD collection store your music?
Come to the Mathematics and Computer Science Department's block-4 lunch seminar series where Prof. Jonathan Bredin will illuminate how your digital music player can store thousands of songs, how music representation differs for iPods and CD collection, how to manipulate audio to change pitch and tempo or add reverb using free software, and why computer scientists and mathematicians care about digital audio.
The talk will cover basic signal processing, including interpretation of the Discrete Fourier Transform and filtering signals in the time domain.
No calculus, programming, or music-theory background will be assumed.
Pizza will be served.
BLOCK 3 , 2009
Friday Oct 30th 2:30 pm: Andrea Bruder of Colorado College.
Title: Orthogonal polynomials and left-definite theory
Abstract: Orthogonal polynomials were first studied by Legendre and Laplace in their work on celestial mechanics around 1800. Over 100 years later, the same polynomials came up as solutions to the Schrödinger equation in quantum mechanics. I will talk about the classical orthogonal polynomials (i.e. the Jacobi, Hermite, and Laguerre polynomials) and their properties, and then turn to the general left-definite spectral theory developed in a recent paper by Littlejohn and Wellman (2002). It is best introduced from a differential equations point of view, and I will consider the Legendre equation (and the Legendre polynomials) as an example.
Friday Nov 6th at NOON: Zach Treisman of Western State College
Title: Images and patterns in hyperbolic geometry. Rating G
Abstract: Changing a basic assumption about parallel lines gives rise to a new and fascinating geometry, called hyperbolic geometry. We'll look at some of the models that one can make of this geometry, and use them to explore some of the ways in which it is unlike the geometry that we are used to. This talk will be gratuitously full of pictures and conspicuously short on equations.
Friday Nov 13th 2:30pm: Amelia Taylor of Colorado College
Title: The Geometry of Phylogenetic Invariants. Rating PG13
Phylogenetic trees are one key tool that biologists use to describe evolutionary relationships and phylogenetic invariants are polynomials, based on a given tree and model of evolution, that may be helpful in determining phylogenetic trees. Two major obstacles to using phylogenetic invariants directly in biology are the fact that they are difficult to obtain and the fact that the polynomials do not give a full geometric description of the statistical model. In particular, there are non-trivial polynomial inequalities that must be satisfied and are difficult to describe. I will describe some examples of both the polynomials and the inequalities and give some recent results by myself, co-authors and others in this study of the missing polynomial inequalities.
Familiarity with linear algebra and polynomials helpful
BLOCK 2, 2009
Friday Oct 2nd 2:30 pm: Barbara Prinari of UCCS
Title: Solitons: A singular and beautiful phenomenon: beauty and novelty of nonlinear systems (rated PG/PG13).
Abstract: In 1834 lord Scott Russell described an unexpected phenomenon. He happened to observe a bell-shaped wave (solitary wave), produced by a boat in a narrow channel around Edinburgh, which continued its course along the channel for miles and miles, apparently without change of form or diminution of speed. In spite of this early observation, it was only in 1965 that waves of this type were identified as an expression of nonlinear phenomena (solutions of certain 'special' nonlinear Pies that were later on termed 'integrable'), and the word 'soliton' was coined.
In this seminar I will illustrate the history of solitons and soliton theory, and deal with some of the associated mathematics.
In particular, I will focus on the so-called Inverse Scattering Transform, an extremely powerful technique that can be considered as the nonlinear analogue of the Fourier Transform to solve an initial value problem for linear PDEs.
(Note: This talk will be largely PG with some PG13 material towards the end.)
Friday Oct 9th at NOON: Jane McDougall of Colorado College
Title: Ptolemy's Theorem, past and present (rated PG).
Abstract: Claudius Ptolemy of the first century AD is remembered for many things. In cartography he was first to use the coordinates of latitude and longitude to describe locations on the earth. He leaves us with the first known map projections and is first on record to have discussed the problems of projecting portions of the curved surface of the earth onto a flat map one would find in an atlas. He is known for one of the first trigonometry books, which superseded that of Hipparchus and was used for centuries thereafter. He is also remembered for his theorem which until recently was routinely taught at the high-school level.
This talk will address the origins of Ptolemy's theorem, its refinements, and applications to some of the topics listed above, and including characterizations of curvature in geometric spaces of modern analysis.
Friday Oct 16th 2:30pm: Sunil Chetty of Colorado College
Title: Rank and a generalized parity conjecture for elliptic curves (rated R).
Abstract: Following Mordell's proof that the group of rational points of an elliptic curve is finitely generated, the study of elliptic curves has largely centered around understanding the size, known as the rank, of a minimal set of generators for a given curve. The famous Birch and Swinnerton-Dyer Conjecture asserts that this rank exactly coincides with the order of vanishing of the L-function of the curve at the value s = 1. As a stepping stone, one attempts to understand this conjecture modulo 2, i.e. to determine if the parity of the rank matches the parity of the order of vanishing of the L-function. We will discuss a method of approaching this type of question via theories of analytic and arithmetic local constants.
BLOCK 1, 2009
Friday September 4th - 2:30pm, TSC 221:
Title: Functions of Graphs and Hypergraphs by Stefan Erickson- Rated PG
Abstract: Zeta functions are one of the most important tools in number theory. Broadly speaking, zeta functions are built out of the "primes" of a mathematical object. In fact, zeta functions are the subject of the Riemann Hypothesis and the Birch Swinnerton-Dyer Conjecture, two of the seven million-dollar Millennium problems.
An interesting development in recent years is to define zeta function for graphs. One can hope that graph zeta functions behave similarly to other zeta functions. In this talk, we will investigate what a "prime" means in a graph, how to define the zeta function of a graph, and some nice properties of these functions. Along the way, we will draw many pretty pictures and explore the possibility of generalizing to hypergraphs. Some familiarity with infinite series and products would be useful.
Friday September 11th - NOON, TSC 122:
Title: Exploring Number Theory via Diophantine Equations by Sunil Chetty (Riley Scholar)- Rated PG
Abstract: Number theory is one of the oldest areas of mathematics, with many problems which are very easy to state and yet very difficult to solve. In so-called ''Diophantine Equations'', one wishes to find integral or rational solutions to polynomial equations in several variables.
Understanding the solutions to particular Diophantine equations allows one to make progress on some of the more easily stated number-theoretic problems. Our aim is to explore some Diophantine equations, their solutions, and some related elementary and advanced problems.
Friday Sep 18th - NOON, TSC 122:
Shandelle Henson of Andrew's University to give a talk, title and abstract to be supplied.
BLOCK 8 2009
Friday, April 24th, at noon in TSC122:
Putnam and Modeling Team Report, from CC students (Rated PG)
In this session, students who participated in the two important annual contests (the Mathematical Modeling Contest and the Putnam Exam) will discuss their experience, and describe some of the mathematics involved in the problems they worked on. Come join our CC participants!
Friday, May 1st, at noon in TSC122:
Tilings in Wonderland, by Kathy Merrill (Rated PG)
We in the math department are extremely pleased to have Kathy Merrill back in our department for block eight! Tilings, such as those that appear in M.C. Escher's art or in the Alhambra, have long been of interest to mathematicians, artists and scientists. The tiles we usually see have the property that identical copies of them can be moved around to cover the plane without overlap. This talk will focus on shapes that not only tile the plane by copies moved around, but also tile by copies expanded and contracted (like Alice nibbling the mushroom). Such a shape is called a wavelet set, and is related to the mathematical theory of image compression.
Friday, May 8th, at 2:30 p.m. in TSC229:
Brouwer's Fixed Point Theorem, by Stefan Erickson (Rated PG)
Fixed points of functions (points where f(x)=x) play an important role throughout mathematics. There are many applications, including general equilibrium theory in economics and the football college ranking system. Although there are many methods of finding fixed points, we would like to know when a function actually has a fixed point. The Brouwer Fixed Point Theorem provides one set of conditions when a function is guaranteed to have a fixed point.
After a brief introduction and motivation, Stefan will present a simple and elegant proof of the Brouwer Fixed Point Theorem. Along the way, he will prove and make use of a combinatorial result known as Sperner's Lemma. He will also discuss some of the neat applications of fixed point theorems.
The talk will be followed by a wine & cocktail party at Stefan's house (1627 N. Franklin St.) starting at 4:00pm. Significant others and small children are encouraged to come!
BLOCK 7 2009
Friday, April 10th, 2009: at 2:30 p.m. in TSC229
Joseph Silverman's Elliptic Curves, by Stefan Erickson (Rated R)
Elliptic curves are one of the most mysterious and powerful objects in all of number theory. Elliptic curves were used to prove Fermat's Last Theorem, the most famous and longest unsolved conjecture. One of the $1,000,000 Millenium Problems is the Birch Swinnerton-Dyer Conjecture is about elliptic curves. They are also used in modern cryptography, including protecting President Obama's Blackberry.
Stefan will present a talk given by Joseph Silverman, a professor at Brown University and a leading expert in elliptic curves. His slides tells the tale of sublime nature of these curves, explains the amazing connection between the algebraic elliptic curves and analytic L-functions associated with those curves, and gives a heuristic reason why we should believe the Birch Swinnerton-Dyer Conjecture. Although this talk does not assume any knowledge of elliptic curves, some familiarity with number theory, algebra, and analysis is recommended.
Friday, March 6th at 2:30 p.m., in Tutt Science 122
Dr. Robin Wilson, speaking on Lewis Carroll in Numberland.
( This is a general interest talk which presumes no mathematical background.)
Abstract: If Charles Dodgson (Lewis Carroll) had not written 'Alice's Adventures in Wonderland' and 'Through the Looking-Glass', he would probably be remembered as a pioneering photographer, one of the first to consider photography as an art rather than simply as a way of recording images. But his day job was as a mathematics lecturer at Christ Church, the largest college of Oxford University. But what mathematics did he do? How good a mathematician was he? And how influential was his work? In this illustrated talk Robin will answer these questions by describing Dodgson's work in geometry, algebra, logic and the mathematics of voting, and on the lighter side Robin will present some of the puzzles and paradoxes with which he entertained his contemporaries
Tuesday, March 10th at 12 Noon in Tutt Science Center 122:
The mathematics of rock climbing, by ANDREA BRUDER, Baylor University (Rated PG)
Andrea will talk about some applications of mathematics in rock climbing. First order linear differential equations can be used to determine the optimal shape of camming devices (aka "friends"), and a second order equation models how a (dynamic) rope catches a falling climber. This has implication for how we climb and for the testing of climbing gear in terms of the maximal force that the rope exerts on the climber at the end of a fall.
Friday, February 20th at 1 p.m. in Packard Hall:
YEA, WHY TRY HER RAW WET HAT?
by Dr. Robin Wilson of the Open University in England (Rated G)
Our frequent visitor Dr. Robin Wilson will give an interesting and entertaining illustrated lecture exploring the connections between mathematics and music, as part of the Music Department's celebration of emeritus professor Carlton Gamer's 80th birthday. This talk will feature musical examples and commentary by CC composers. In order to understand the title, you will need to attend the talk! The first event in the Carleton Gamer Retrospective is a concert in Packard Hall at 7:30 p.m. on Thursday, February 19th, which is free and open to the public.
Monday, February 23rd at 2:30 p.m., in TSC 122:
Projective Spaces (Rated PG)
by Amanda Beeson, a Ph.D. candidate at the University of California, San Diego; she is a candidate for a non-tenure-track job in our department.
What happens to the real plane if we add on a so-called line at infinity and declare that parallel lines intersect in a point on that line so that, in this new space, ANY two distinct lines intersect in exactly one point? We get what is called real projective two-space and, as you shall see, lots of interesting beautiful geometry ensues!
Tuesday, February 24th at noon, in TSC 229:
Groups of Special Units (Rated R)
by Amanda Beeson
We will introduce briefly the philosophy of the Stark conjectures about special values of L-functions, the work of Sinnott regarding unit groups, and Anderson's construction of the maximal almost abelian extension of the rational numbers. We will see that these three areas tie neatly together in the form of groups of special units, out of which we will produce new and surprising trigonometric and L-function identities.
Friday, February 27th at noon, in Tutt Science Center 122:
CANCAN YOU KENKEN?
by John Watkins and Maija Benitz (Rated G)
One week ago The New York Times began carrying a numerical logic puzzle next to its daily crossword puzzle. The new puzzle, called KENKEN ®, which loosely means "wisdom squared," was invented in Japan in 2004 and, like Sudoku, it involves filling a square grid with numbers without repeating a number in any row or column. But, unlike Sudoku, doing a KENKEN ® puzzle requires arithmetic and sometimes a good deal of cleverness. Join John and Maija for an introductory session on "The World's Most ADDictive Puzzle ™". There will be free pizza available at the talk!
There will a third Fearless Friday talk this block too - watch this space for further details!!
BLOCK 5 2009
Thursday January 29th, at 2:30pm in TSC 122
Frames and the Uncertainty Principle in Time-Frequency Analysis (Rated PG)
by Jan Cameron, Riley Scholar Candidate
You may have heard of the Heisenberg uncertainty principle, or even studied the result in a physics or chemistry class. It is less known that a similar principle is at the heart of the "localization problem," one of the deepest mathematical problems in time-frequency analysis. Time-frequency analysis is the area of mathematics devoted to understanding how the frequency and spectral content of signals (e.g. sounds, images, electrical activity) evolve through time, and how this information can be stored digitally. In this talk, we will develop some basic tools of time-frequency analysis, and see how one tool in particular, frame theory, emerges as a means to address the localization problem. Frame theory is not only useful for applications in engineering and signal analysis, but also has deep connections to several areas of mathematics. As time permits, we will explore a few such connections, and discuss some current avenues of research in frame theory that may be of interest to you.
Friday, January 30th, at noon in TSC 122 (featuring Pizza!!)
Web Security for Liberal-arts Majors (Rated G)
Business Logic Flaws are a large problem for the Computer Security industry. The security group, OWASP put out their top ten flaws in web applications. These include vulnerabilities such as leaving configuration scripts available to anyone after the installation of a product, SQL Injection, and Cross Site Scripting. All of these are problems that can be detected and fixed by code review and configuration management
Business Logic Flaws are much harder. These problems cannot be detected by normal means because they are not errors. They come about by the lack of understanding by software developers of the customer's ability to do the unexpected.
We will discuss a group of these Business Logic Errors and how to fix each one, if they can be fixed. The lecture will be understandable by anyone who has used the Internet.
Friday, February 6th, at 2:30 p.m. in TSC 229:
Constructing and Squaring Lunes Whose Fundamental Quotient is Algebraic, by Mike Siddoway (Rated R)
Hippocrates of Chios (5th century BC), with perhaps our first known proved theorem, showed that a special lune could be constructed with ruler and compass, and could also be "squared" using the same tools. This was the first "curved" figure that admitted such properties. Hippocrates would find two other such lunes, and several more were found about 2200 years later bringing the total to five. Using Galois theory and advanced techniques for finding solutions to polynomials, it was settled in the mid-20th century that these were the only five "constructible squarable lunes" given the classical strictures placed on the problem. In particular, the classical problem assumes (in part) that the quotient of the arcs defining the lune (the "fundamental quotient') is rational. Using deep theorems of Hermite and Lindemann, Gelfond and Schneider, and Fields medalist Alan Baker it can be shown that no new "constructible squarable lunes" are gained by extending beyond the commensurable setting to lunes whose fundamental quotient is properly algebraic. Along the way many other stunning results play crucial roles including the Eisenstein irreducibility theorem and Ferrari's method for finding the roots of quartic equations. This grand problem is a treasure trove of mathematical history whose solution spans two and a half millennia and relies on many beautiful theorems that came to light along the way. In this talk I'll follow on my 4th block presentation "Call of the Lune," with three basic goals. I'll first provide some of the details that settled the classical question. Then we will answer the question raised by distinguished biology professor Werner Heim, "Are there any constructible, squarable lenses? ("Lenses" are lunes which bow out on both sides.) Finally, we'll put to rest the other question raised at the last talk, "Are there any constructible, squarable lunes whose fundamental quotient is a non-rational algebraic number?"
BLOCK 4 2008
Friday, December 5th at 2:30 p.m., in TSC 221: Looking back from a theorem of Kaplansky to a famous result due to Krull, and forward to a theorem on Bezout rings with (many) stops in a paper by Matlis along the way, by Mike Siddoway, Colorado College
(Rated R)
We will look at an old theorem due to Krull on valuation domains, and move on to a new view of this work by Kaplansky that led to a result for more general domains. We'll then consider non-domains (rings with zero divisors) and see how Kaplansky's idea provides a setting for work in reduced rings (special rings with zero divisors for which a^2 = 0 implies a=0). In particular we will be studying semihereditary Bezout rings and semigroups. (I'll define these in the talk). A Bezout semigroup S is called "semi-hereditary" if for each a in S there is an idempotent (e^2 = e) in S that generates the annihilator of a. Our main result states that a semigroup is a semi-hereditary Bezout semigroup if and only if it is isomorphic to a "semigroup of divisibility" of a semi-hereditary Bezout ring partially ordered by reverse inclusion. This theorem harkens back to Krull's famous theorem and is inspired by Kaplansky's work with Bezout domains, and Matlis's work with reduced rings.
Friday, December 12th at Noon in TSC 122 (with PIZZA!)
Title: The Life and Legacy of Alan Turing, by David Brown (Rated G)
In 1935, a young graduate student named Alan Turing lay in a field near Cambridge, thinking about a deep problem in mathematical logic. He imagined machines that were capable of carrying out calculations according to a set of rules. Turing's daydreams eventually became the mathematical foundation for the invention of computers (to a computer scientist, your laptop and your iPhone are examples of "Turing machines"). In his remarkable and tragically short career, Turing helped lay the groundwork for computer science and artificial intelligence, and explained how patterns can form spontaneously in biological systems. He helped crack Nazi codes during WWII, but was later persecuted by his own government for his homosexuality. In this talk, I will provide a sketch of Turing's life and some of his key mathematical ideas. I will also try to make the case that this shy, rather obscure English mathematician belongs in the short list of the greatest scientists of all time.
BLOCK 3 2008
Friday, November 14th, at 2:30 p.m. in TSC229: Transforming results for monomial ideals to toric ideals, by Amelia Taylor
(Rated R)
In combinatorial and computational commutative algebra it is common that research is presented for monomial ideals with the caveat that "the work also holds for toric ideals." I will give examples of such research, including work of my own, and then talk about how to make the leap from a monomial ideal result to a result on toric ideals.
Friday, November 7th, at noon in TSC122 (featuring PIZZA!): The Call of the Lune: A 2500 year journey from perhaps our first proof, through Euler in the 1700s, and on to a complete solution in the 20th century, by Mike Siddoway
(Rated PG)
A loon is a large odd duck, whose eerie call makes Midwesterners wax nostalgic. A lune is a curved figure formed by the arcs of two circles that has similarly haunted the longings of mathematicians. Think of a crescent moon. The Greeks knew how to square arbitrary polygons. That is, they could construct a square with the area of any given polygon using simple tools. When a figure can be squared we mathematicians say it is quadrable. The big test was to construct an equal area square" for a figure with curved sides. The circle was the big prize. Could the circle be squared? Hippocrates of Chios (c. 470 - c. 410 BCE) found that he could square several lunes that had special properties, and it seems he even believed he had a proof for squaring the circle. One wonders. It turns out the proof is (very) flawed, and the search for squarable lunes went on. Euler (1707 -1783) took on the problem early in his life, and again when in his twilight years. He found several new lunes that admitted construction with classical tools, bringing the total to five quadrable lunes. In the decades that followed, revolutionary advances in algebra led to a proof that the circle couldn't be squared. A consequence of this was that arbitrary lunes were not quadrable. But was Euler's collection of five squarable lunes complete? Advances were made through the 19th Century, but it wasn't until the mid-20th Century that several young Soviet mathematicians (Chebotarev and his student Dorodnov) proved that Hippocrates and Euler had found all the quadrable lunes. We'll look at this grand story and marvel at the deep insights of Hippocrates, the technical acumen of Euler, and the beauty of modern methods that settled the problem once and for all.
Friday, October 31st, at 2:30 p.m. in TSC229: Three Short Talks About Bacterial Genetics, by David Brown
(Rated PG-13 for content, R for pace)
I'll give brief and probably incoherent summaries of three of my research projects in varying stages of completion. First, I'll talk about a project with Phoebe Lostroh in which we developed a method for reconstructing time series for gene expression dynamics from reporter protein data. This will be a review and slight extension of a talk I gave last fall. Second, I'll talk about my ongoing attempt to model a set of gene interactions in the bacterium Pseudomonas fluorescens. This system is an example of quorum sensing, in which bacteria detect their population density and adjust their behavior in response. This part will be an extension of a talk I gave last spring. Finally, I'll talk briefly about the next project I have in mind: a stochastic model of quorum sensing. The material is not overly challenging, but I will be going kind of fast.
BLOCK 2 2008
Friday, October 17, 2008, at 2:45 (note the special time), in TSC229: Amelia Taylor
Vanishing Ext and Tor
(Rated R)
In commutative algebra, the vanishing of Ext and Tor modules often gives information about key properties of a ring, a module, or, in some of Amelia's recent research, complexes. Amelia will start with defining a complex. Then she will work her way towards giving some of the motivation and recent results on the impact of vanishing of Ext (mostly, but maybe some Tor as well) of semi-dualizing complexes.
Monday October 13th - Noon, TSC 122: Fred Adler, University of Utah
Title: Time lags and sex-biased prevalence in hantavirus
Abstract: This talk examines two aspects of the population dynamics of Sin Nombre virus, the cause of hantavirus pulmonary syndrome in humans. First, we use population trajectories from the literature and mathematical modeling to analyze the expected time lag between deer mouse population peaks and peaks in deer mouse seroprevalence. Because the virus is not transmitted vertically, rapid population growth can lead initially to reduced prevalence, but the resulting higher population size may later increase contact rates and generate increased prevalence. Second, we investigate the role of sex-biased prevalence in persistence of the virus. Analyzing data on over 2000 individual mice captured, we determined that males are more likely to contract the infection due to higher susceptibility or higher encounter rates and that they live longer than females. Mathematical models predict that these differences can buffer the disease from extinction by concentrating it in the subgroup most likely to survive population bottlenecks.
Friday October 10th - Noon, TSC 122: John Watkins
Teen Mathematics: a tour of amazing mathematical discoveries
(Rated G)
John will give an interesting report on mathematical discoveries made by the very young. Come here about some exciting mathematics, and enjoy pizza while you're doing so. This talk is in celebration of Homecoming Weekend.
Friday October 3rd - 2:30pm, TSC 229: Associate Professor Michael Dorff from Brigham Young University
Title: Harmonic mappings, minimal surfaces, and linear combinations.
(Rated PG-13)
Abstract: Complex-valued harmonic mappings can be regarded as generalizations of analytic functions studied in a beginning complex analysis course. Minimal surfaces in R^3 are saddle surfaces whose mean curvature vanishes everywhere and can be visualized as the soap film formed on wire frames. In this talk, I will present some general background about harmonic mappings, minimal surfaces, and the connection between the two.
Then I discuss some new results on the convex combinations of mappings and surfaces. One application of these results is that they offer an easy way to construct harmonic mappings onto nonconvex polygonal domains and to construct the corresponding minimal graphs over these nonconvex domains.
This provides us with a method to construct new families of minimal surfaces that are generalizations of the Jenkins-Serrin surfaces. These results are related to work done by Peter Duren, Jane McDougall, and Beth Schaubroeck.
BLOCK 1, 2008
Friday September 12th - NOON, TSC 122:
Research opportunities in the math department, by Amelia Taylor (and Jonathan Bredin, David Brown, Stefan Erickson, and Mide Siddoway :) ) --Rated G
(Please click on the link to view the PDF presentation!)
ch by students takes many forms in the mathematics department. We will talk about some of the departmental student research projects from the recent past and most importantly advertise questions and opportunities for students to do research in the future. Problems will be presented by Amelia Taylor, David Brown, Jonathan Bredin, Mike Siddoway and Stefan Erickson. Please join us to find out about all the fun problems to work on!
This talk will be immediately followed by the fall math department picnic - there'll be Mexican food available in the Skilling Commons (near the math department on the second floor)!
Friday September 19th - 2:30pm, TSC 229:
More Fantastic p-adics, by Stefan Erickson -- Rated PG-13
A standard question in analysis is how to construct the real numbers from the rationals. But what happens when we measure distances between rational numbers in different ways? We get the p-adic numbers, every bit interesting and exciting as the reals.
After a playful introduction, we will delve into some of the fascinating properties of the p-adic numbers. We will develop the p-adic metric, p-adic functions, and the local-global principle. Finally, we will explore some applications to number theory. This is a continuation of a talk given in Block 8 of the last school year.
BLOCK 8, 2008 (April -May)
Friday April 25th at 2:30 p.m., TSC229:
A mathematical model of the Gac/Rsm signal transduction network in Pseudomonas fluorescens, by David Brown (Rated PG-13)
Living cells must make many decisions about what genes to express in response to the conditions in their environment. For example, the soil-dwelling bacteria Pseudomonas fluorescens detects its local population density and adjusts various processes like antibiotic production in response. This behavior is regulated by a network of genes that activate and inactivate each other, and control the production of antibiotics and signaling molecules. David will present a mathematical model of this system, consisting of a set of differential equations for the cell population and various molecular concentrations. He will show how the model can be tuned to match published data on gene expression dynamics, then explore the behavior of the system under various conditions.
Friday May 2nd at noon, TSC 122: Putnam Exam Discussion (Rated PG)
The Putnam Exam is a highly competitive national competition offered each December. Undergraduates from all over the country take it. Each year Colorado College fields a team, after spending the fall working on sample problems and problem-solving strategies. A group of CC students will report on the problems on this year's exam, and discuss how they can be solved.
Friday May 9th at 2:30 p.m., TSC 229:
The Fantastic p-adics, by Stefan Erickson (Rated PG13)
A standard question in analysis is how to construct the real numbers from the rational numbers. But what happens when we measure distances between rational numbers in different ways? We get the p-adic numbers, every bit interesting and exciting as the reals.
After a playful introduction, Stefan will delve into some of the fascinating properties of the p-adic numbers. He will develop the p-adic metric, p-adic functions, and the local-global principle. Finally, he will explore some applications to number theory.
BLOCK 7, 2008 (March-April)
Thursday, April 3rd starting at 2:00 p.m. in TSC 122:
STUDENT DISTINCTION TALKS!
Schedule of Talks
Distinction
3:00: Tra Ho, "Explicit Formulas for Real Hyperelliptic Curves of Genus 2 on the Projective Coordinate System"
3:30: Jes Coyle, "The Effect of Fire on Ponderosa Pine Forest Structure"
4:00: Matthew Swanger, "A Fault Tolerable Content Addressable Network"
4:30: Jette Petersen, "The Initiation of Cancer via Two Mutations"
5:00: Andrea Buchwald, "The Unit Group of Real Biquadratic Number Fields"
Friday, April 4th at 2:30 p.m. in TSC 229:
"The classification question for Leavitt path algebras", by Enrique Espino, University of Cadiz (Rated R)
Enrique will obtain isomorphisms between the Leavitt path algebras of specified graphs. From these isomorphisms he is able to achieve two ends. First, he shows that the K_0 groups of various sets of purely infinite simple Leavitt path algebras, together with the position of the identity element in K_0, classify the algebras in these sets up to isomorphism. Second, he shows that the isomorphism between matrix rings over the classical Leavitt algebras, established previously using number-theoretic methods, can be re-obtained via appropriate isomorphisms between Leavitt path algebras.
Friday, April 11th at Noon. in TSC 122:
"Counting the days", by Marlow Anderson, Colorado College (Rated PG)
The 16th century classicist J. J. Scaliger introduced the modern science of chronology, which is the careful correlation of historical dates. He combined three important calendric cycles (the Julian cycle of 28 years, the indiction cycle of 15 years, and the Metonic cycle of 19 years), to obtain a cycle of 7980 years, which is long enough to include all dates in written human history. Scaliger's cycle happens to begin on Jan. 1 4713 BC. Modern astronomers have refined this to make a literal count of days, leading to the Julian Day Number for any date in historical times. The Julian Day Number is the tool used to translate from any one calendar to any other. There is some modestly interesting modular arithmetic involved in making these calculations. As a bonus, it's easy to compute how many days old you are!
Friday, April 11th at 2:30 in TSC 122:
VENTURE GRANT RECIPIENT TALKS!
2:30: Andrea Buchwald, "The Tradition of Geometry in Architecture: from Ancient Greece to the Ottoman Empire"
3:00: Melissa Miller, "Exploring Geometries and Symmetries of Greek and Turkish Mosaics "
Tuesday, March 25th at 2:30 p.m. in Tutt Science Center 122:
"Squaring the Square" by Molly Maxwell, PhD University of Minnesota, rated PG.
A squared rectangle is a dissection of a rectangle into non-overlapping squares, all of different sizes. The problem of finding a squared square caught the interest of Brooks, Smith, Stone, and Tutte in the 1930's while they were undergraduates at Trinity College in England. The "Trinity Four" used two approaches for finding squared rectangles. Their first approach involved solving a system of equations, while the second involved rephrasing the problem in terms of electrical networks. This talk includes a description of the Trinity Four's approaches and their resulting squared squares. Since this time, many more example have been found, and several artists have included these patterns in their work. The talk concludes with a discussion of several generalizations of this problem, including squared cylinders, squared tori, cubed cubes, and triangulated equilateral triangles. (Student Level talk)
Wednesday, March 26th at 2:30 p.m. in Tutt Science Center 229:
"Self-Dual Spanning Trees and Self-Dual Matroid Bases" by Molly Maxwell, PhD University of Minnesota, rated R.
In 1979 Tutte studied the properties of a class of graphs called central reflexes. In particular, he proved that the number of spanning trees is the square of the number of selfdual spanning trees. Several years later, Kalai defined higher dimensional spanning trees in the boundaries of simplices and proved analogues of Cayley's Theorem and the Cayley-Pr¨ufer Theorem for these trees. In addition, Kalai conjectured that an enumerator for these higher dimensional trees is the square of an enumerator for self-dual trees.
In this talk, we'll discuss a class of cell complexes that contains both Tutte's central reflexes and Kalai's boundaries of simplices. We'll look at k-dimensional spanning trees in these complexes and a result which shows that an enumerator for these trees is the square of a related enumerator for self-dual trees. This result gives a new proof of Tutte's theorem and solves Kalai's conjecture when k is odd. We'll also discuss a weighted version of Kalai's conjecture and a bijective proof of Tutte's theorem for odd wheels, which are a specific class of central reflexes. (Faculty Talk)
Friday, March 28th at 2:30 p.m. in TSC 229:
"An unusual polynomial, and Error-correcting Codes", by Rachel Pries of Colorado State University (Rated PG13)
Rachel will describe a polynomial that has special properties in number theory. She will then show how it is important for error-correcting data-transmission codes.
BLOCK 6, 2008 (February - March)
Friday, February 22nd at 2:30 p.m. in Tutt Science Center 229:
"Special Low Dimensional One-sided h-cobordisms" by Fred Tinsley and Roberto Pelayo Rated R (with a little bit of X)
During the 1990's Fred and Bob Daverman proved that in high dimensions (ambient dimension larger than five), every one-sided h-cobordism admits a special Morse function. We obtained partial results for ambient dimension five but learned nothing about ambient dimension four. A cobordism is an n-manifold W with two boundary components M and N. A Morse function is a continuous map f:W ? [0,1] with f -1(0) = M, f -1(1) = N. To be special, we require in addition that f -1(t) be a closed (n-1)-manifold for each t Î (0,1). In other words, W is a generalization of a product.
Fred and Roberto have embarked upon a study of the ambient dimension-four case in which we plan to combine Roberto's knowledge of three-dimensional topology with Fred's knowledge of high-dimensional topology. They will talk about the differing techniques required for the differing ambient dimensions and then present strategies for attack.
Friday, February 29th at noon in Tutt Science Center 122 (featuring free pizza!)
"The Other Polyhedra" By Steven Janke (rated PG)
Nearly everyone has seen the five Platonic polyhedra (tetrahedron, cube, octahedron, dodecahedron, and iscosahedron) and probably many of us know a few things about them. However, there are a lot more polyhedra that are interesting for various reasons. This talk will review some of the geometry of the Platonic polyhedra and then look at four more that should be included in the same category. And then there are another 66 that we shouldn't dismiss. With the help of software, the talk will examine some of these polyhedra visually, trace a little history, outline some of the main mathematical results, and perhaps uncover what makes these shapes aesthetically pleasing.
BLOCK 5, 2008 (January- February)
Tenure Track Candidate Talks!
Stephanie Treneer, whose Ph. D. is from the University of Illinois; she is presently at Dartmouth College.
Monday, February 11th at 2:30 p.m. in Tutt Science Center 122:
"Exploring Partitions of Numbers" (Rated PG)
A partition of a positive integer n is a sequence of positive integers that sum to n. The partition function p(n) counts the partitions of n without regard to order. This deceptively simple function has led to a rich theory. We'll look at two elementary methods for analyzing partitions: Ferrers graphs and generating functions. We'll then briefly discuss the connection between partitions and modular forms, which has led to some recent surprising results about p(n).
Tuesday, February 12th at noon in Tutt Science Center 229:
"Arithmetic of the Fourier Coefficients of Modular Forms" (Rated R)
In the last decade, the theory of modular forms has provided an important framework in which to study questions in arithmetic number theory. Modular forms act as generating functions for many partition functions, divisor functions, and other combinatorial functions. We will review the role modular forms have played in establishing congruence properties for such functions, and give general results about congruences for the coefficients of weakly holomorphic modular forms. We will also discuss new work involving harmonic Maass forms.
Stefan Erickson, whose Ph. D. is from the University of California at San Diego; he is presently a member of our department.
Thursday, February 7th at 2:30 p.m. in Tutt Science Center 122:
"Secret Codes and Algebra: How to Use Curves to Take Over the World " (Rated PG)
Since ancient times, codes and ciphers have been used to transmit vital information without it falling into the wrong hands. The advent of the computer age has produced a strong demand for fast and secure cryptosystems. Today, cryptography is found in military, economic, and personal applications, including Internet commerce and digital signatures.
Most modern cryptosystems are based on computationally hard problems from mathematics, such as the factoring of the product of two large prime numbers in RSA. Recently, there has much interest in using elliptic curves as the basis for cryptosystems. After describing elliptic curves and motivating their use, we will demonstrate a variety of applications. We will also introduce hyper-elliptic curves and describe a number of open questions related to their arithmetic and suitability for cryptography.
Friday, February 8th at 2:30 p.m. in Tutt Science Center 229:
"Computational Evidence of the Stark Conjectures" (Rated R)
The Stark Conjectures predict that the leading term of Taylor series of the L-functions for an abelian extension K/k can be evaluated using certain algebraic units in K. These units should also produce abelian extensions of k, which allows one to do explicit class field theory. It is possible to numerically verify the Stark Conjectures by evaluating the L-function to high precision, then recognizing the coefficients of the defining polynomial of the units. After giving a gentle introduction to evaluating L-functions, we give an example of how the Stark Conjectures can be computationally verified.
Katherine Ott is a Ph.D. candidate at the University of Virginia. She will be giving two talks during her visit; the first is aimed particularly at students.
Thursday, January 31st at 2:30 p.m., in Tutt Science Center 122
"Buffon's Needle Problem" (Rated PG), by Katherine Ott, University of Virginia.
In this talk Katy will introduce a classic problem in geometric probability known as Buffon's Needle Problem. Using geometry and calculus techniques Katy will solve the problem two ways and discuss its very surprising answer. Finally, we'll see how this simply-stated problem applies to Monte Carlo methods and some homeless ants.
Friday, February 1st at 2:30 p.m., in Tutt Science Center 229
"Boundary Integral Equations in Non-Smooth Domains" (Rated R), by Katherine Ott, University of Virginia.
A method for solving boundary value problems, such as the Dirichlet problem, on non-smooth domains is the method of layer potentials. In this talk Katy will discuss how she employs the techniques of layer potential operators and the tools of Mellin transform analysis to study the well-posedness of several other boundary integral equations related to mathematical physics, engineering and computer science.
BLOCK 4, 2007 (November-December)
Friday, November 30th at 2:30 p.m. in TSC229:
"Heegaard Decompositions and the Combinatorics of 3-Manifolds", by Bob Pelayo (Rated PG13)
A Heegaard structure on a 3-manifold M is a natural decomposition of M into two particular subsets (called handle-bodies), which are glued together via a self-homeomorphism of a 2-dimensional surface. Since every 3-manifold has a Heegaard decomposition, understanding the various Heegaard structures on M would allow us to combinatorially classify all 3-manifolds. Furthermore, since these Heegaard decompositions are given in terms of a 2-dimensional surface S, we will explore a simplicial complex called the complex of curves, which categorifies the space of simple closed curves on S. Using these various notions, we will explore the combinatorics, dynamics, geometry, and algebra associated to Heegaard decomposition and rephrase important topological theorems (e.g., the Poincare Theorem) in terms of Heegaard structures. This talk will begin with an introduction to 2- and 3-manifold topology and culminate in the unification of various seemingly disparate subfields of mathematics involved in 3-manifold topology.
Friday, December 7th, at noon in TSC122:
"Inferring Gene Activity Dynamics from Reporter Protein Data, or You Might Already Know Enough Math to Do Research",
by David Brown (Rated PG) PIZZA will be served
View seminar slides from David's talk (pdf)
We all know that genes encode proteins. What most of us don't think about is that they do so in a highly regulated, dynamic manner. Genes can be "turned on", "turned off", or somewhere in between, depending on the type of cell and the cues that it receives from other cells and the environment. Measuring the activity level of a gene directly is difficult, so biologists measure the accumulation of a "reporter" protein that is easy to detect. This type of data reveals average gene activity over a period of time, but the underlying dynamic patterns are not immediately obvious. This summer Phoebe Lostroh and I realized that the dynamic patterns are in fact encoded in the reporter protein data, and that they can be extracted using some basic calculus. I will discuss the method and its biological uses, and reflect on how I stumbled across this problem and what it implies about doing applied mathematical research.
BLOCK 3, 2007 (November)
Friday, November 2nd at 2:30 p.m. in TSC 229
"Robust multivariate statistics and data depth", by
RYAN ELMORE, Colorado State University (Rated PG-13)
In this talk, Ryan will discuss multivariate analogues to the univariate "sign"-based suite of procedures. Specifically, we will introduce a multivariate median and hypothesis test for estimating and testing central location of a multivariate distribution. The methods are developed using the concept of spherical and elliptical data depth. The talk will focus on the geometry and computational details related to Ryan's proposed methods. Basic issues pertaining to multivariate statistics will be introduced as part of the talk; however, a rudimentary understanding of beginning statistical concepts is assumed.
Friday, November 9th at noon in TSC122 (Kresge Lecture Hall)
"The Archimedes Palimpsest", by MARLOW ANDERSON, Colorado College (Rated PG)
In 1906 J. L. Heiberg discovered that a medieval prayer book contained previously unknown works by Archimedes, as an erased text on the parchment used to construct the prayer book. His discovery revolutionized the study of Archimedes - one of these works (The Method) contained valuable insights into how Archimedes approached problems we would today solve by calculus. The manuscript was in private hands for much of the twentieth century - during its disappearance it suffered considerable damage. In 1998 Christie's sold it at public auction for $2 million to an anonymous bidder - who has made it available for study by scholars. In this talk Marlow will relate a bit more of this romantic tale, and in addition explain how Archimedes approached what we would call integration.
Friday, November 16th at 2:30 p.m. in TSC229
"Applications of Mathematical Computing to Probability" by FRED TINSLEY, Colorado College (Rated PG 13)
The Math Department bestows upon the calculus-based probability and statistics sequence a role central to the statistics track of our major. One objective for this talk is to institute a dialogue about the reasons for placing this subject matter at the heart of the curriculum. Fortunately, liberal arts students crave to understand the whys and wherefores of conventional practice, and the probability-statistics sequence continues to satisfy this desire. However, incorporating concrete applications to understanding actual phenomena would yield a significant benefit. Fred will combine mathematical probability with modern mathematical computing to highlight useful ways of analyzing data. Fred will argue that in a rush to purge mathematics from their courses, contemporary statistics educators miss a valuable opportunity to support their students in discovering the wonderful connections among all these subjects.
At the conclusion Fred will suggest ways for students interested in the statistics track of the major to engage in research incorporating the mathematical side of these subjects.
BLOCK 2, 2007 (October)
Friday, October 12th, at noon, in TSC 122
JONATHAN BREDIN of Colorado College (Rated PG)
A Coming to Terms:
Bodies Trump Brains,
Elephants Can't Play Chess,
Boys Blow Things Up,
and We All Hate Complexity
(Managing Complexity with a Subsumptive Architecture)
Controlling and planning actions for a mobile robot is a classic challenge in artificial intelligence (AI). The traditional approach leverages a sense-model-plan-act loop and found success in formulating and solving complex goals in laboratory settings, but also serves as one of the most visible research directions to succumb to the AI winter-a collapse in AI research and funding lasting into the mid 1980s. Several obstacles stymied the traditional approach. Researchers had trouble modeling real-world settings and logical planning proved fragile in dynamic environments. Furthermore, few projects could scale to utilize multiple processors, accommodate multiple robot goals, or handle conflicting sensor measurements.
The introduction of subsumption architecture is frequently credited as the paradigm that thawed the AI winter for robotics. The idea coordinates independent modules, each competing to represent a simple low-level behavior, and serves as a useful paradigm to tackle complexity.
We'll look back at a critical moment in AI history, introduce subsumption-style organization, and apply the paradigm through designing a subsumption architecture to control virtual spaceships inside a multi-player programming game. The bulk of the material presented will be accessible to a general audience. No programming experience will be assumed.
BLOCK 1, 2007 (September)
Mathematics and the Chessboard by JOHN WATKINS (Rated PG)
The simple and familiar 8×8 grid of squares we call the chessboard has been the inspiration for countless puzzles and mathematical problems over the years. The Knight's Tour Problem - in which a knight visits every square of the board - is perhaps the most famous of these puzzles and was recently featured by magician Ricky Jay in his hit Broadway show, even though Euler found a solution to this problem 250 years ago! The 8-Queens Problem - place eight queens on the board so that none of them attacks any of the others - was solved by Gauss. This talk, in which John will discuss these problems as well as many of the variations that continue to challenge mathematicians to this day, is based on his book Across the Board: The Mathematics of Chessboard Problems, which has just been reissued in paperback. FOLLOWED IMMEDIATELY BY THE FALL MATH PICNIC, FEATURING FUN & GAMES AND MEXICAN FOOD!
Friday, September 14th at noon, in TSC 122:
Frodo Meets Neo: Studying rings using matrices by AMELIA TAYLOR (Rated PG-13)
Recently, quotients of polynomial rings have been popping up in unexpected places, such as the study of phylogenetic trees in evolutionary biology and the game SET. We will start the talk with an introduction to quotients of polynomial rings and motivate our study of rings by introducing a few of these recent new uses for rings. A powerful tool for studying such rings (and modules) is the minimal free resolution. Minimal free resolutions in this context consist of a sequence of maps given by matrices and we can give many examples. In fact, there are algorithms for computing minimal free resolutions, but describing the structure based only on data from the ideal can be difficult and many of the results combine algebra, topology and combinatorics. We conclude with recent results and open questions on the structure of the minimal free resolution of certain quotient rings, including results by undergraduates. FREE PIZZA AT THIS TALK!
Friday, September 22nd at 2:30pm, in TSC 223:
Isomorphisms between matrix rings over Levitt algebras: some elementary number theory yields a not-so-elementary result by GENE ABRAMS of UCCS (Rated PG-13)
For a field K, the Levitt K-algebra R=L_K(1,n) was defined and investigated in the early 1960s, and has enjoyed an intense revival of interest over the past few years. Although this algebra might look somewhat strange at first glance, it is pretty easy to describe in terms of generators and relations.
Consider the ring M(t, R) of t by t matrices with entries from the Levitt K-algebra R. In this talk Gene will prove that R and M(t, R) are actually isomorphic as rings if and only if gcd(t,n-1)=1. (Really! A ring isomorphic to a matrix ring over itself!) The proof of this result involves some straightforward number theory (which Gene will describe in detail).
This result is joint work with E. Pardo and P. N. Anh, and was obtained during the fall of 2006, when Professor Anh was a visitor at Colorado College.
If there's time, Gene will also talk about a connection between the Levitt algebra and Cuntz C* algebras from analysis.
Friday, May 12th, at 2:30 in TSC 229:
PRIMES OF THE FORM x^2+ny^2 (Rated PG13)
By Stefan Erickson of Colorado College
A fundamental question in number theory is: "Which primes can be written as x^2 + n*y^2?" Pierre Fermat was the first to give complete answers for n = 1, 2, and 3. As n grows larger, the solution becomes more difficult to obtain. The search for an answer for arbitrary n leads first to the study of quadratic forms, which produces partial success. Class field theory provides a straightforward answer for all n (certain polynomials must have a solution modulo p). However, finding these polynomials remains a challenge. Complex multiplication finally provides an algorithm for explicitly constructing the desired polynomials. Along this mathematical journey, we will encounter the likes of Euler, Lagrange, Legendre, Gauss, Dirichlet, Hilbert, and others.
Friday, May 5th, at noon in TSC122:
PROBLEMS FROM THE PUTNAM AND RAWLES EXAM (Rated PG)
Presentations by CC students who took these exams, plus Wojciech Kosek and Rahbar Virk
Each year CC fields a team of students who take the national Putnam Exam, and we also sponsor a local competition called the Rawles Exam. Come for pizza and the opportunity to hear about solutions to selected problems from these two exams.
Friday, April 28th at 2:30 p.m. in TSC 229:
Dynamical methods in combinatorial number theory: history and some new results about prime numbers
By Wojciech Kosek of Colorado College (Rated PG13)
A deep connection between Ramsey theory and the measurable and topological dynamics was first discovered in 1977, when Furstenberg proved the Multiple Recurrence Theorem and showed its combinatorial consequences. This led to new proofs of known theorems in combinatorics such as theorems of Hindman, van der Waerden and Szemeredi. Dynamical perspective on combinatorics stimulated a very fruitful interplay between the two disciplines, leading to new combinatorial results as well as to the development of new tools in dynamics.
Prime numbers have fascinated people since the dawn of time. There are statements and conjectures about them, which are easy to state, yet whose proof is still evading us. In 2004, Ben Green and Terrence Tao announced their latest result: the primes contain arithmetic progressions of arbitrary length. To prove this result they adapted some methods from ergodic theory, harmonic analysis and number theory. Time permitting, some ideas used in the proof of this result will be discussed. Also, we will look at a short an elegant proof of the Hindman's theorem which uses ultrafilters.
Except for a few brief comments, technical details will be avoided and the talk will be accessible to students majoring in mathematics, who are encouraged to come. Hope you will join us.
BLOCK 7, 2006
Friday, April 14: 2:30, TSC 122
Representations of groups and other algebraic objects in mathematics
Fred Tinsley
PG-13 (linear algebra is a help but not a necessity)
In algebra, groups are sets with a single (binary) operation imposed that satisfies certain properties. A favorite example is the group of symmetries of the equilateral triangle. For example, we can let j be the group element that reflects the triangle through one of its medians and r the group element that rotates the triangle counterclockwise by 120°:
These two elements can be used to generate the entire group of symmetries of the equilateral triangle that turns out to have 6 elements. The complete multiplication table is given below where i is the identity (i.e., leaves the triangle alone):

Classical representation theory desires to realize this group in terms of matrices and matrix multiplication. Computations become mechanical and do not require a table. Students of linear algebra can check that the assignments,
precisely realize these symmetries.
We will look at representations of this type and also as objects other than matrices. We hope to appreciate the utility of viewing algebraic objects in several different settings.
Thursday , April 6th: (TSC 122)
Student Distinction Talks
2:30-4pm
Friday, March 31st: Noon (TSC 122)
How To Solve Sudoku
Robin Wilson, Open University
Rated G
This talk is rated G, and should be of wide interest to those of you who are addicts, and those of you who are not! The sudoku epidemic has swept the world faster than you can count to nine. But what is sudoku? How did it arise? How many possible sudoku puzzles are there? And how do you solve the wretched things? Robin Wilson is the author of two sudoku books and he will reveal all! Enjoy the talk, and the pizza provided.
BLOCK 6, 2006
Tuesday, March 14th,
at 3:30 p.m. in TSC229:
Sobolev on ends: a story of growth and decay - (Rated R)
Stephen Buckley, National University of Maynooth, Ireland
In 2001, Li and Wang showed that if the Dirichlet Problem for the Laplacian on an end of a complete manifold has a positive leasteigenvalue, then that end must have at least a certain exponential rate of growth or decay, and these rates are sharp. In this talk we discuss similar sharp results for (q,p)-Sobolev inequalities, the case p=q=2 of which implies the Li-Wang theorem. This is joint work with Pekka Koskela.
Friday, March 10th
TSC229 , at 2:30 p.m.
Revisiting Calculus, and a few Function Spaces - (Rated PG13)
Jane McDougall
Over the past 100 years, updated versions of integration and differentiation have become mainstream. Although calculus is useful for solving so many problems, its processes have some limitations in terms of what converges where and when. In this talk, I will present some of the ideas that allow us to extend our notion of what can be integrated and/or differentiated. We will also consider some particular sets of functions where convergence works better (be prepared for the infamous D). One of the themes will be that life is easier when we have dense subsets and decent limit theorems.
Friday, March 3rd,
TSC122 , Noon
The Banach-Tarski Paradox - (Rated PG)
by John Watkins
The worlds of mathematics and physics have provided us with many instances of things that are almost impossible to believe and yet are true. Quite naturally, some of the more delightful of these "impossible, yet true" statements have been given names: Zeno's Paradox, Russell's Paradox, Einstein's Twin Paradox, Braess's Traffic Flow Paradox, and so on. But, surely, the finest paradox of all is the Banach-Tarski Paradox.
The Banach-Tarski Paradox says that it is possible to dissect a ball - that is, a solid sphere - into a finite number of pieces and then rearrange these pieces to form two balls exactly the same size as the original ball. Yet, nothing has been created or stretched in the process! All that has happened is that pieces of the original ball have been moved around in space like the pieces of a jigsaw puzzle and yet somehow you manage to end up with twice as much stuff as you started with.
Impossible, you say. If that's what you think, then come and hear this talk. I will even show you a remarkable relationship between this paradox and the famous woodcut of Escher, Circle Limit IV, that is hanging in our hallway.
BLOCK 5, 2006
Friday, January 27th
TSC 223, 2:30pm
Divisibility in the Fibonacci Numbers -(Rated PG)
by Stefan Erickson
The Fibonacci numbers arose from a famous problem posed 800 years ago about reproducing rabbits. There are many remarkable properties of this sequence. We will dive into two questions. Which Fibonacci numbers divide other Fibonacci numbers? Which primes divide some Fibonacci number? The incredible answers will be revealed, along with their analogues for a similar sequence called the Lucas numbers. In the process, we will uncover some of the marvelous interconnections between the Fibonacci numbers, the Lucas numbers, and the Golden Ratio.
BLOCK 4, 2005
Friday, December 16th,
at 2:30 p.m., TSC 223
The dynamics of multiplication by 2 and 3 mod 1
By Dan Rudolph, Colorado State University, Rated R
The dynamics of the single endomorphism x maps to nx mod 1, with n > 1, is extremely chaotic but not difficult to understand. It is modeled by the one-sided shift map on infinite sequences of digits from {0,1, ..., n-1}. In this form it is seen to have many periodic points, many closed invariant subsets and many invariant measures.
> When one extends this to the dynamics of a commuting pair of such maps, the simplest being x maps to 2x and x maps to 3x mod 1, the situation changes dramatically. Dr. Rudolph will offer a proof of the celebrated theorem of Furstenberg, that any closed invariant set for both these maps is either finite or all of [0,1). He will discuss what is known about the space of invariant measures and what remains unknown.
Friday, December 9th,
Noon, TSC 122
Penrose tiles
By Kathy Merrill, Rated PG
Most tilings of the plane that we are familiar with, such as those that appear in M.C.Escher prints or on floors or wallpapers, are periodic. That is, most tilings have a pattern that repeats in two independent directions. Roger Penrose discovered a pair of tiles called kites and darts that fit together to tile the plane, but only in ways that have no repeating pattern. This talk will introduce you to Penrose tiles, their relationship to the Golden ratio, and their applications to understanding quasi-crystals of aluminum and manganese and even to making toilet paper. As a part of the talk, you will have a chance to build some Penrose tilings yourself.
Friday, December 2nd, 2005
2:30 p.m., TSC 122
The Indian Wars, Eugenics, and Statistics: A Broader View of Scientific Racism Before the Outbreak of World War II
By Mike Siddoway, Rated G
Racial theories figured to devastating effect in the "Indian Wars" of the American West, and a half-century later in the "Final Solution" in Nazi Germany. Arguments surfaced from the across the political spectrum in late 19th century America in support of eradicating the native population. When the Minneconjou followers of Chief Big Foot were shot down at Wounded Knee Creek in 1890, marking the end of the war on the plains, US laws were already excluding immigrants on the basis of race and ethnicity. The British anthropologist Francis Galton coined the term "eugenics" in the 1880's. The US eugenics movement was very strong through the early part of the 20th century and was followed closely by adherents in Europe. Sterilization and miscegenation laws appeared in numerous states. Two of the most prominent figures in the history of statistics, Karl Pearson and Ronald Fisher both held positions in England as Professors of Eugenics in the decades before the start of WWII. By the mid 30's, when National Socialism was tightening its grip on Germany, eugenics had become a fixture in the intellectual landscape in the US and Europe. The rigor that mathematics brought to the eugenics movement through the development and study of statistics made it all the more accepted as a serious branch of science. In this talk I will look a little wider than Stefan
Kühl did in his excellent book on the links between American eugenics and National Socialism by including discussion of American racial attitudes during the "Indian Wars" and the possible role that mathematics played in legitimizing the broader eugenics movement before the outbreak of WWII.
BLOCK 3, 2005
Establishing Multi-Path Connectivity in Sensor Networks
Jonathan Bredin, Colorado College
Rated PG
November 18th at 2:30 (TSC 223)
A sensor network is a collection of small devices that use radios and sensors, such as photometers, microphones, and accelerometers, to collect information to monitor an environment and distributively process their data. We are interested in methods to distributively detect and repair multi-path connectivity (k-vertex connectivity) of the radio network imposed by a sensor-network deployment. Guaranteeing k-vertex connectivity simultaneously provides fault tolerance against node failures and high capacity through multi-path routing.
Jonathan will present the first approximation algorithms that compute the placement of a minimum number of additional sensors to augment an existing network into a k-connected network, for any desired parameter k. He will also present a distributed augmentation algorithm, and its implementation using Mica2 Motes.
This talk will cover a wide range of work from theory to application and will be accessible to students with little college math or computing experience.
Predicting Retirements of CC Faculty
Fred Tinsley, The Colorado College
Rated PG
November 11th at noon (TSC 122)
Fred and John Stinespring (Economics) have been working on a model to predict major trends in the cost of paying full-time faculty at Colorado College. Given the rather careful adherence by the Administration to the clearly delineated Faculty Salary Model, much of the work is entirely straightforward. However, the most unpredictable and, unfortunately, the most significant element is the distribution of retirements. The replacement of highly paid full professors by lower paid beginning assistant professors mitigates the factors that drive up costs.
Fred will discuss their approach, which is a blend of two major methodologies in statistics: Bayesian and frequentist. Don't let these terms scare you off. If you have taken the equivalent of either Calculus I or Intro to Stats, then you will see an intriguing application of what you have learned.
Of course, an extensive literature on predicting retirements exists. However, Fred will argue the importance of first investigating the problem on your own before diving into the literature. This has always been for Fred one of the most valuable approaches to learning.
The mathematics of representing human population density on a map
Michael Gastner, The Sante Fe Institute
Rating: PG13
November 4th at 2:30 (TSC 223)
Dr. Gastner will present a method to construct "cartograms," maps in which the sizes of geographic regions such as countries or provinces appear in proportion to their population. Such maps, although unconventional, are often the best way to represent human data. The construction of cartograms turns out to be a challenging mathematical problem. A variety of methods have been put forward, but none is entirely satisfactory. In particular, they often produce highly distorted maps that are difficult to read or projections that are badly behaved under some circumstances. In many cases the methods proposed are also computationally demanding, sometimes taking hours to produce a single map.
The method discussed, based on the mathematics of diffusing gas particles, makes low demands on our computational resources, while also producing elegant, well-behaved, and useful cartograms. The speaker will illustrate the technique with applications to election results, disease incidence, and the optimum distribution of service facilities.
BLOCK 2, 2005
Friday, October 21st, 2:30 in TSC 229
Luis Melara
Title: "A Homotopy Method in Regularization of Total Variation Image Denoising Problems"
Rating: R (Some graduate mathematics assumed)
Homotopy methods can often be used to make mathematical programming problems easier to solve. One source of notoriously difficult problems is total variation image
denoising. In this talk, a direct relationship is established between the radius of the Kantorovich ball guaranteeing the convergence of Newton's method and the regularization parameter. This radius of convergence increases with the choice of the regularization parameter. A homotopy method in the regularization parameter is employed to improve numerical performance of Newton's method applied to these problems. The talk will summarize a convergence analysis and present numerical results.
Friday, October 7th, 2:30 p.m. in TSC 229:
Dr. Craig Guilbault of the University of Wisconsin-Milwaukee
Title: "Exotic Behavior near infinity on Manifolds"
Rating: R (Some graduate mathematics assumed)
Most of Dr. Guilbault's research involves the topology of manifolds. Currently, he studies "open" or "non-compact" manifolds. In simple terms, these are surfaces that are (in some sense) infinite in size. For example, the Euclidean plane? that extends infinitely outward? is non-compact; whereas the 2-sphere is compact. The extra flexibility permitted by non-compactness opens the door to exotic behavior "near infinity". There are many examples of non-compact spaces that decompose into simple finite pieces but have quite complicated structure at infinity. More technically, Professor Guilbault's research is motivated by current work in geometric group theory and by new approaches to the well known Borel and Novikov Conjectures in manifold topology. He is especially interested in Z-compactifications of open manifolds and complexes, and structure theorems for open manifolds with non-stable fundamental groups at infinity. Of special interest are universal covers of aspherical manifolds, and manifolds which admit CAT(0) metrics (a generalized version of non-positive curvature). Professor Fred Tinsley of Colorado College has collaborated with Professor Guilbault in studying non-stable fundamental groups at infinity.
Friday, October 14th, noon in TSC 227
Steven Janke
Title: "Making Rotations Easier: The Quaternion Method"
Rating: PG13 (A lot of undergraduate mathematics assumed)
Rotating objects in three dimensions is a problem key to many fields: computer graphics, aeronautics, physics. The traditional way to find the coordinates of points on a rotated object is to use trigonometry to describe rotations around each of the standard axes. However, in the early nineteenth century, William Hamilton discovered another method that falls in the intersection of abstract algebra and geometry. Although his method of quaternions fell into disfavor at the end of the nineteenth century, it has been revived recently by those interested in computer graphics and offers an often-useful perspective on rotations.
BLOCK 2, 2005
October 7th at 2:30: Craig Guilbault with a research talk
October 14th at noon: The second Friday student talk, by Steven Janke
October 21st at 2:30: Luis Melara
BLOCK 1, 2005
Friday, September 23rd, noon, TSC 122:
Mike Siddoway
Title: "Closed Chains with Tetrahedral Links?"
Rating: PG.
Abstract: Is it possible to make a complete chain using tetrahedrons that share a
complete face with nearest neighbors? We can make a chain in two-space using
equilateral triangles or squares that share edges. In three-space there are
infinitely many complete chains with cubes as links that share sides. What if
we use tetrahedrons in three-space? Josh Laison and CC student Luc Wilson
posed this problem last spring during the distinction talks and Mike will give
a solution that uses linear algebra and some polynomial theory. It turns out
that the deciding factor is whether 2/3 can be the root of certain polynomials
with integer coefficients.
Friday, September 16th, 2:30 p.m., TSC 229:
Jane McDougall, on "More about star domains"
Rated R.
Jane gives a follow-up talk to her seminar last spring on her work with Beth
Schaubroeck on Harmonic Analysis on Star Domains, including some really recent
developments.
Friday Sept. 9, 2:30, TSC 229
Jonathan Bredin
Title: Models for Truthful Online Double Auctions
Rating: G
Abstract:
An online double auction (DA) is a market where traders arrive and depart
a market at arbitrary times to exchange a commodity. Each trader wishes
to maximize its economic surplus and possess private information regarding
its values, costs, and lifetimes. We will present an auction primer and
move on to present a general method to design truthful online DAs, such
that
no agent can benefit from misreporting its arrival time, duration, or
valuation. The family of DAs is parameterized by a pricing rule, and
includes a generalization of McAfee's truthful DA to this dynamic setting.
We present an empirical study, in which we study the allocative-surplus and
agent surplus for a number of different DAs. Our results illustrate that
dynamic pricing rules are important to provide good market efficiency for
markets with high volatility or low volume.
No mathematical, economic, or computational background is assumed. Our work
should be consumable for all interested parties (rated G). The work
presented is a joint effort with David Parkes.